Is 1 6 Bigger Than 1 4
Juapaving
May 12, 2025 · 5 min read
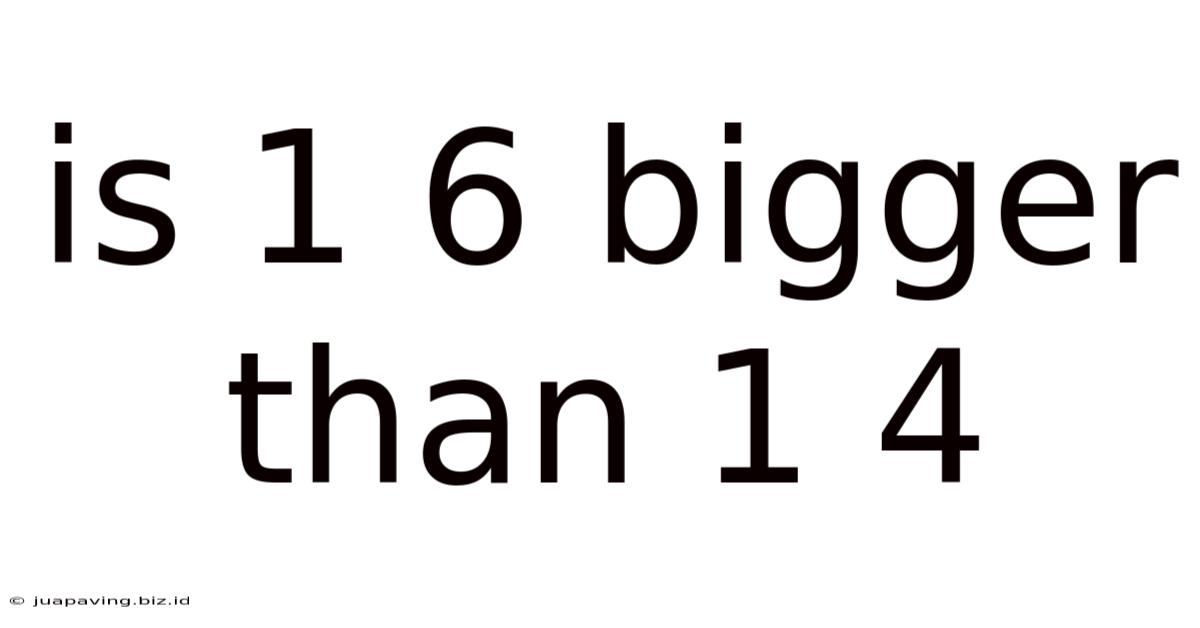
Table of Contents
Is 1/6 Bigger Than 1/4? Understanding Fractions and Comparisons
The question, "Is 1/6 bigger than 1/4?" might seem simple at first glance, but it touches upon fundamental concepts in mathematics, particularly the understanding and comparison of fractions. This article will delve into a comprehensive explanation of how to determine which fraction is larger, explore various methods for comparing fractions, and discuss the broader implications of understanding fraction relationships.
Understanding Fractions: Parts of a Whole
Before directly comparing 1/6 and 1/4, let's establish a solid understanding of what fractions represent. A fraction, in its simplest form, represents a part of a whole. It consists of two main components:
- Numerator: The top number of a fraction, indicating the number of parts we're considering.
- Denominator: The bottom number of a fraction, indicating the total number of equal parts the whole is divided into.
For instance, in the fraction 1/4, the numerator (1) tells us we have one part, and the denominator (4) tells us the whole is divided into four equal parts. Similarly, 1/6 represents one part out of a whole divided into six equal parts.
Visualizing Fractions: A Pictorial Approach
A powerful way to grasp the concept of fractions and compare their sizes is through visualization. Imagine two identical pizzas.
- Pizza 1: Cut into four equal slices (representing 1/4).
- Pizza 2: Cut into six equal slices (representing 1/6).
If you take one slice from each pizza, you'll immediately see that the slice from Pizza 1 (1/4) is larger than the slice from Pizza 2 (1/6). This visual representation provides an intuitive understanding of the relative sizes of the fractions.
Comparing Fractions: Different Methods
Several methods exist for comparing fractions, each with its own advantages and applications. Let's examine the most common approaches:
1. Visual Comparison (as demonstrated above)
This method is excellent for building an initial intuitive understanding, especially for smaller fractions. However, it becomes less practical and less precise when dealing with larger or more complex fractions.
2. Finding a Common Denominator
This is a fundamental and widely used method for comparing fractions. The key principle is to rewrite both fractions so that they share the same denominator. This allows for a direct comparison of the numerators.
To compare 1/6 and 1/4, we need to find the least common multiple (LCM) of their denominators, 6 and 4. The LCM of 6 and 4 is 12. We then rewrite both fractions with a denominator of 12:
- 1/6 = (1 x 2) / (6 x 2) = 2/12
- 1/4 = (1 x 3) / (4 x 3) = 3/12
Now, comparing the numerators, we see that 3/12 (1/4) is greater than 2/12 (1/6).
3. Converting to Decimals
Another approach is to convert both fractions into their decimal equivalents. This provides a numerical comparison that's easily understood.
- 1/6 ≈ 0.1667
- 1/4 = 0.25
Comparing the decimal values, it's clear that 0.25 (1/4) is larger than 0.1667 (1/6).
4. Cross-Multiplication
This method offers a concise way to compare two fractions without the need to find a common denominator. We cross-multiply the numerators and denominators:
- 1/6 ? 1/4
- (1 x 4) ? (1 x 6)
- 4 ? 6
Since 4 is less than 6, we conclude that 1/6 is smaller than 1/4.
Why Understanding Fraction Comparison is Crucial
The ability to compare fractions is a fundamental skill that extends far beyond basic arithmetic. It's crucial in various aspects of life, including:
- Baking and Cooking: Following recipes often requires understanding fraction ratios of ingredients.
- Construction and Engineering: Precise measurements and calculations in construction rely heavily on fraction manipulation.
- Financial Literacy: Understanding fractions is essential for comprehending percentages, interest rates, and other financial concepts.
- Data Analysis: Interpreting data presented in fractional or percentage forms requires a strong grasp of fraction comparisons.
- Science and Technology: Many scientific and technological calculations involve fractions and their relationships.
Beyond the Basics: Exploring More Complex Fraction Comparisons
The principles discussed above can be extended to compare more complex fractions, those with larger numerators and denominators. The same methods – finding a common denominator, converting to decimals, or cross-multiplication – can be applied effectively. However, with larger fractions, the calculations might become more involved.
For instance, consider comparing 7/12 and 5/8. Finding a common denominator (24) would lead to:
- 7/12 = 14/24
- 5/8 = 15/24
Thus, 5/8 is larger than 7/12.
Common Mistakes to Avoid When Comparing Fractions
Several common errors can lead to inaccurate comparisons of fractions. These include:
- Ignoring the denominator: Simply comparing numerators without considering the denominators will always lead to incorrect results.
- Incorrectly finding the LCM: Errors in calculating the least common multiple can throw off the entire comparison.
- Miscalculations during conversion: When converting fractions to decimals, even small calculation mistakes can lead to incorrect conclusions.
Conclusion: Mastering Fractions for a Stronger Mathematical Foundation
The seemingly simple question, "Is 1/6 bigger than 1/4?" opens a door to a deeper understanding of fractions and their importance in various aspects of life. By mastering the techniques for comparing fractions—whether through visual representation, finding a common denominator, converting to decimals, or cross-multiplication—you build a stronger mathematical foundation and enhance your ability to tackle more complex problems and real-world scenarios involving fractions. Remember to practice regularly and double-check your calculations to avoid common errors. The ability to confidently compare fractions is an invaluable skill that contributes significantly to success in many areas of study and work.
Latest Posts
Latest Posts
-
What Is The Lcm Of 36 And 18
May 12, 2025
-
Which Of The Following Is Not A Pure Substance
May 12, 2025
-
What Is The Difference Between Nuclear Reaction And Chemical Reaction
May 12, 2025
-
Is Brass A Mixture Compound Or Element
May 12, 2025
-
How Many Tens Are In 700
May 12, 2025
Related Post
Thank you for visiting our website which covers about Is 1 6 Bigger Than 1 4 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.