Identity Property Of Addition And Multiplication
Juapaving
Apr 02, 2025 · 7 min read
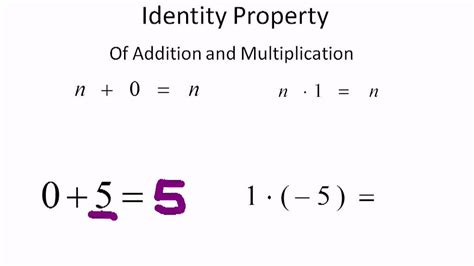
Table of Contents
The Identity Property of Addition and Multiplication: A Deep Dive
The identity property, a fundamental concept in mathematics, plays a crucial role in simplifying calculations and understanding the structure of number systems. It dictates how certain numbers, known as identity elements, interact with other numbers under specific operations. This article delves deep into the identity property of addition and multiplication, exploring its applications, proofs, and significance across various mathematical contexts.
Understanding the Identity Property
The identity property, also called the identity law, states that there exists a unique element within a set that, when combined with any other element in the set under a given operation, leaves that element unchanged. Let's break this down for addition and multiplication separately.
Identity Property of Addition
The identity property of addition states that there exists a number, called the additive identity, which, when added to any other number, results in the original number. This additive identity is zero (0). Formally, we can express it as:
a + 0 = a and 0 + a = a, where 'a' represents any real number.
This property is intuitively clear. Adding zero to any number doesn't alter its value. For example:
- 5 + 0 = 5
- -12 + 0 = -12
- 0 + 100 = 100
This property is essential for simplifying algebraic expressions and solving equations. It allows us to add zero without affecting the equation's balance.
Identity Property of Multiplication
Similarly, the identity property of multiplication states that there exists a number, called the multiplicative identity, which, when multiplied by any other number, results in the original number. This multiplicative identity is one (1). Formally, we can express it as:
a × 1 = a and 1 × a = a, where 'a' represents any real number.
Again, this is intuitively understandable. Multiplying any number by one doesn't change its value. For instance:
- 7 × 1 = 7
- -3 × 1 = -3
- 1 × 25 = 25
This property is crucial in algebra and beyond. It simplifies expressions, helps solve equations, and forms the foundation for many advanced mathematical concepts.
Applications of the Identity Property
The identity properties of addition and multiplication aren't just theoretical concepts; they have practical applications across numerous mathematical areas and real-world scenarios.
1. Simplifying Algebraic Expressions
The identity properties are invaluable tools for simplifying algebraic expressions. We can add or multiply by the identity element without changing the expression's value, allowing us to manipulate the expression into a simpler form.
Example:
Simplify the expression: 3x + 0 + 5x – 2x + 1 × x
Using the identity properties:
- 3x + 0 = 3x (Additive Identity)
- 1 × x = x (Multiplicative Identity)
The expression simplifies to: 3x + 5x – 2x + x = 7x
2. Solving Equations
The identity properties are fundamental to solving equations. Adding zero or multiplying by one does not alter the equality. This allows us to isolate variables and find solutions without changing the equation's truth.
Example:
Solve the equation: x + 5 = 5
Subtract 5 from both sides: x + 5 - 5 = 5 - 5
Using the additive identity: x + 0 = 0
Therefore, x = 0
Example:
Solve the equation: 2x = 2
Divide both sides by 2: 2x/2 = 2/2
Using the multiplicative identity (implicitly, since dividing by 2 is multiplying by 1/2): 1x = 1
Therefore, x = 1
3. Number Systems and Abstract Algebra
The identity properties extend far beyond basic arithmetic. They are fundamental axioms in the study of different number systems (integers, rational numbers, real numbers, complex numbers) and in abstract algebra, forming the basis for groups, rings, and fields. The existence of an additive and multiplicative identity is a key characteristic defining these algebraic structures.
4. Real-World Applications
While less directly apparent, the identity properties underpin numerous real-world applications where mathematical calculations are involved. From simple accounting and financial calculations to complex engineering and scientific models, the ability to add zero or multiply by one without affecting the outcome is an inherent part of the mathematical foundation.
Proofs of the Identity Properties
While intuitively obvious, the identity properties can be formally proven within various axiomatic systems. The proofs typically rely on the field axioms or similar foundational postulates. Here's a conceptual outline of a proof for real numbers:
Proof of the Additive Identity (Zero)
- Axiom: For every real number 'a', there exists an additive inverse '-a' such that a + (-a) = 0.
- Axiom: Addition is associative: (a + b) + c = a + (b + c).
- Consider: a + 0. We want to show this equals 'a'.
- Additive Inverse: We know a + (-a) = 0.
- Associativity: a + 0 = a + (a + (-a)) = (a + a) + (-a).
- Further steps (depending on the axiomatic system): The exact steps to reach a + 0 = a will depend on other axioms of the system. This might involve showing that the additive inverse is unique.
Proof of the Multiplicative Identity (One)
- Axiom: For every non-zero real number 'a', there exists a multiplicative inverse '1/a' such that a × (1/a) = 1.
- Axiom: Multiplication is associative: (a × b) × c = a × (b × c).
- Axiom: Multiplication distributes over addition: a × (b + c) = (a × b) + (a × c).
- Consider: a × 1. We want to show this equals 'a'.
- Multiplicative Inverse: We know a × (1/a) = 1.
- Associativity and Distributivity: Further steps using the above axioms (and possibly others, like the existence of 0 and its properties) will lead to the conclusion that a × 1 = a.
These proofs illustrate that the identity properties aren't arbitrary rules but logical consequences of more fundamental axioms defining number systems. The specific steps and axioms needed will vary depending on the chosen axiomatic framework.
Distinguishing the Identity Property from Other Properties
It's important to distinguish the identity property from other properties frequently encountered in mathematics, such as the commutative, associative, and distributive properties.
- Commutative Property: The order of operation doesn't affect the result (a + b = b + a; a × b = b × a). The identity property doesn't deal with the order of operations; it focuses on the special element that doesn't change the outcome.
- Associative Property: The grouping of numbers doesn't affect the result ((a + b) + c = a + (b + c); (a × b) × c = a × (b × c)). The identity property doesn't change the grouping; it changes the number being acted on.
- Distributive Property: Multiplication distributes over addition (a × (b + c) = (a × b) + (a × c)). This property links addition and multiplication, whereas the identity property deals with each operation separately.
Understanding these distinctions is essential for applying the properties correctly in calculations and problem-solving.
The Identity Property in Different Number Systems
The identity property holds true for various number systems, including:
- Natural Numbers: The additive identity (0) isn't included in natural numbers (1, 2, 3...), but the multiplicative identity (1) is.
- Integers: Both the additive identity (0) and multiplicative identity (1) are present.
- Rational Numbers: Both the additive identity (0) and multiplicative identity (1) are present.
- Real Numbers: Both the additive identity (0) and multiplicative identity (1) are present.
- Complex Numbers: Both the additive identity (0) and multiplicative identity (1) are present.
Even in more advanced mathematical structures like matrices and vectors, the concept of identity elements extends, albeit with different representations and properties. For instance, in matrix algebra, the identity matrix plays a role analogous to the number 1 in scalar multiplication.
Conclusion
The identity property of addition and multiplication, though seemingly simple, is a cornerstone of mathematics. It provides a foundational understanding of how numbers interact under these operations, enabling simplification of expressions, solving equations, and building more complex mathematical structures. Its consistent application across various number systems and advanced mathematical fields underscores its significance as a fundamental principle in the broader landscape of mathematical reasoning. Mastering the identity property is essential for anyone seeking to build a strong foundation in mathematics and its numerous applications.
Latest Posts
Latest Posts
-
120 Cm Is How Many Inches
Apr 03, 2025
-
The Scattering Of Light By A Colloid Is Called The
Apr 03, 2025
-
Roots Forming A Number In Logo
Apr 03, 2025
-
Alloy Of Mercury With Another Metal
Apr 03, 2025
-
Can Be Separated By Physical Means
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about Identity Property Of Addition And Multiplication . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
