How To Make A Ratio Into A Percentage
Juapaving
Mar 30, 2025 · 5 min read
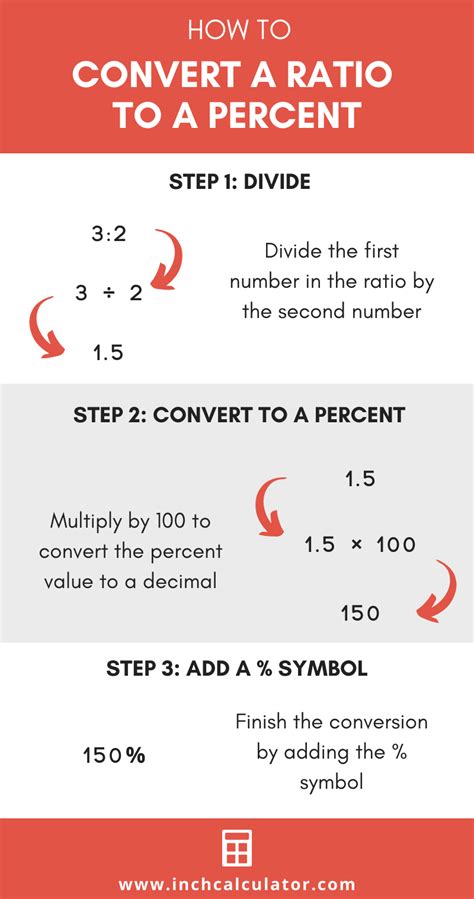
Table of Contents
How to Turn a Ratio into a Percentage: A Comprehensive Guide
Understanding how to convert ratios into percentages is a fundamental skill with wide-ranging applications, from everyday budgeting and cooking to complex statistical analysis and financial modeling. This comprehensive guide will walk you through the process step-by-step, clarifying the underlying concepts and providing practical examples to solidify your understanding. We'll explore various scenarios, including ratios with whole numbers, decimals, and even those involving more than two parts. By the end, you'll be confident in transforming ratios into percentages with ease.
Understanding Ratios and Percentages
Before diving into the conversion process, let's refresh our understanding of ratios and percentages.
What is a Ratio?
A ratio is a mathematical expression that compares the size of one quantity to the size of another. It shows the relative proportions of two or more quantities. Ratios can be expressed in several ways:
- Using a colon:
a:b(reads as "a to b") - Using the word "to":
a to b - As a fraction:
a/b
What is a Percentage?
A percentage is a way of expressing a number as a fraction of 100. It represents a proportion out of a whole, where the whole is always considered 100%. The symbol "%" is used to denote a percentage.
Converting a Ratio to a Percentage: The General Method
The core principle behind converting a ratio to a percentage lies in converting the ratio into a fraction and then expressing that fraction as a percentage. Here's the general method:
-
Express the ratio as a fraction: If your ratio is given as
a:b, rewrite it asa/b. -
Divide the numerator by the denominator: Perform the division
a ÷ b. This gives you a decimal. -
Multiply the decimal by 100: Multiply the result of step 2 by 100. This converts the decimal to a percentage.
-
Add the percentage symbol: Add the "%" symbol to the final result to clearly indicate it's a percentage.
Examples: Ratio to Percentage Conversion
Let's illustrate this with a few examples:
Example 1: Simple Ratio
Let's say you have a ratio of 3:5. Following the steps:
- Fraction: 3/5
- Division: 3 ÷ 5 = 0.6
- Multiplication: 0.6 x 100 = 60
- Percentage: 60%
Therefore, the ratio 3:5 is equivalent to 60%.
Example 2: Ratio with a Decimal
Suppose you have a ratio of 1.5:2.5. Let's convert it:
- Fraction: 1.5/2.5
- Division: 1.5 ÷ 2.5 = 0.6
- Multiplication: 0.6 x 100 = 60
- Percentage: 60%
Again, the result is 60%. Notice that even with decimals in the ratio, the process remains the same.
Example 3: Ratio with Larger Numbers
Let's consider a more complex ratio, 250:500.
- Fraction: 250/500
- Division: 250 ÷ 500 = 0.5
- Multiplication: 0.5 x 100 = 50
- Percentage: 50%
This demonstrates that the method works efficiently even with larger numbers.
Example 4: Ratios with More Than Two Parts
While the above examples focused on two-part ratios, the principle can be extended to ratios with three or more parts. However, you will need to specify which part you want to express as a percentage of the total.
For example, consider the ratio 2:3:5. To find the percentage represented by the '2', we first find the total (2+3+5=10). Then:
- Fraction: 2/10
- Division: 2 ÷ 10 = 0.2
- Multiplication: 0.2 x 100 = 20
- Percentage: 20%
So, '2' represents 20% of the total. You would repeat this process for the other parts (3 and 5) to find their respective percentages.
Practical Applications of Ratio to Percentage Conversion
The ability to convert ratios to percentages has numerous applications across various fields:
-
Finance: Analyzing financial statements, calculating interest rates, and understanding investment returns often involve working with ratios that need to be expressed as percentages.
-
Statistics: Presenting statistical data clearly and concisely often involves converting ratios to percentages for easier interpretation. For instance, expressing the proportion of survey respondents who prefer a particular product.
-
Science: In scientific experiments, ratios are frequently used to represent proportions or concentrations. Converting these ratios to percentages can make the results more accessible and understandable.
-
Everyday Life: Calculating discounts, understanding sales tax, and even figuring out the proportion of ingredients in a recipe all involve working with ratios and percentages.
Common Mistakes to Avoid
While the conversion process is straightforward, some common mistakes can lead to inaccurate results:
-
Incorrect order of division: Always divide the numerator (the first number in the ratio) by the denominator (the second number). Reversing the order will yield the wrong result.
-
Forgetting to multiply by 100: This is a crucial step in converting the decimal to a percentage. Omitting this will give you a decimal representation, not a percentage.
-
Misinterpreting ratios with more than two parts: Clearly identify which part of the ratio you want to express as a percentage of the total.
Advanced Scenarios and Tips for Accuracy
While the basic method suffices for most scenarios, understanding some nuances can improve accuracy and efficiency.
-
Dealing with very small or large numbers: When dealing with extremely small or large numbers, using a calculator is recommended to minimize the risk of calculation errors.
-
Rounding: When the resulting decimal has many digits after the decimal point, you might need to round it to a certain number of decimal places to maintain clarity. However, be mindful of the context and the level of precision required. For example, in financial calculations, precision is critical, while in some everyday calculations, rounding to one or two decimal places is sufficient.
Conclusion
Converting ratios to percentages is a valuable skill that simplifies the understanding and communication of proportions. By mastering the steps outlined in this guide and understanding the underlying principles, you'll be equipped to confidently tackle a wide range of ratio-to-percentage conversion problems, whether in academic settings, professional contexts, or everyday life. Remember to practice regularly to reinforce your understanding and build confidence in this essential mathematical skill. Accurate conversion ensures clear communication and facilitates better decision-making in various situations.
Latest Posts
Latest Posts
-
What Is On A Physical Map
Apr 01, 2025
-
P Block Elements In Periodic Table
Apr 01, 2025
-
Adjective That Starts With L To Describe A Mom
Apr 01, 2025
-
Is 35 A Multiple Of 6
Apr 01, 2025
-
Exponents Worksheets Pdf With Answers 7th
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about How To Make A Ratio Into A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
