How To Find Average Speed With Two Speeds
Juapaving
Mar 29, 2025 · 5 min read
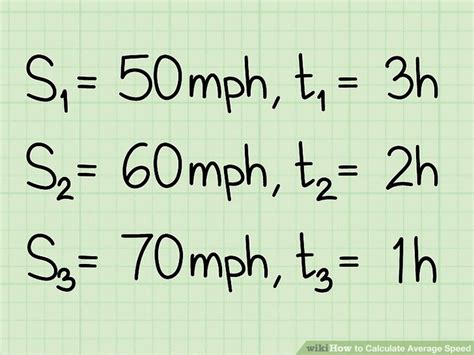
Table of Contents
How to Find Average Speed with Two Speeds: A Comprehensive Guide
Calculating average speed when you have two different speeds might seem straightforward, but there's a crucial distinction to understand: average speed is not simply the arithmetic mean of the two speeds. The correct calculation depends on the time spent at each speed. This article will delve into the intricacies of this calculation, providing various scenarios and formulas to help you master this concept. We'll also explore common misconceptions and offer practical examples to solidify your understanding.
Understanding the Difference: Arithmetic Mean vs. Average Speed
Before diving into the calculations, let's clarify the difference between the arithmetic mean and the true average speed.
-
Arithmetic Mean: This is simply the sum of the speeds divided by the number of speeds. For instance, the arithmetic mean of 40 mph and 60 mph is (40 + 60) / 2 = 50 mph. This is not the correct way to calculate average speed unless the time spent at each speed is equal.
-
Average Speed (Harmonic Mean): This considers the time spent at each speed. It's the total distance traveled divided by the total time taken. This is the accurate representation of average speed in most real-world scenarios.
Calculating Average Speed: The Formula and its Application
The formula for calculating average speed when traveling at two different speeds for different durations is:
Average Speed = (Total Distance) / (Total Time)
Let's break down how to calculate each component:
1. Calculating Total Distance
If you know the speed and time for each leg of the journey, you can calculate the distance for each leg using the formula:
Distance = Speed × Time
Let's say you traveled at 40 mph for 2 hours and then at 60 mph for 3 hours. The distances would be:
- Distance 1: 40 mph × 2 hours = 80 miles
- Distance 2: 60 mph × 3 hours = 180 miles
Total Distance: 80 miles + 180 miles = 260 miles
2. Calculating Total Time
This is simply the sum of the time spent at each speed.
In our example:
Total Time: 2 hours + 3 hours = 5 hours
3. Calculating Average Speed
Now, plug the total distance and total time into the average speed formula:
Average Speed = 260 miles / 5 hours = 52 mph
Therefore, the average speed for the entire journey is 52 mph, significantly different from the arithmetic mean of 50 mph.
Scenario Variations: Different Time Units and Unknown Variables
The basic formula can be adapted to various situations.
a) Different Time Units
Ensure all time units are consistent before calculation. If one speed is given in mph for hours and another in km/h for minutes, convert them to the same unit (e.g., both to mph or both to km/h) before proceeding.
b) One Distance and Two Speeds
Suppose you know the total distance but not the time for each segment. You'll need to use algebra to solve for the average speed.
Let's say the total distance is 260 miles, speed 1 is 40 mph, and speed 2 is 60 mph. We can represent the time spent at each speed as 't1' and 't2'.
We have two equations:
- 40t1 + 60t2 = 260 (Total distance)
- The relationship between
t1andt2needs more context to be solvable. We would need additional information to be able to use these equations. For example, we might know the ratio of time spent at each speed. Or it might be possible to work backwards using the overall average speed, if known.
c) Unknown Speed and Known Time and Distance
If you know the total time, total distance, and one speed, you can calculate the unknown speed using a similar algebraic approach.
Common Mistakes to Avoid
Many mistakes arise from a misunderstanding of the concept of average speed. Here are some common pitfalls to watch out for:
-
Using the Arithmetic Mean: As discussed earlier, this is incorrect unless the time spent at each speed is identical.
-
Incorrect Unit Conversion: Always ensure your units are consistent throughout the calculation.
-
Mixing Distance and Time: Keep track of whether you are dealing with distance or time at each step.
Practical Applications of Average Speed Calculation
Calculating average speed is not just an academic exercise. It has numerous practical applications, including:
-
Travel Planning: Estimate travel time for journeys with varying speeds (e.g., highway driving versus city driving).
-
Fuel Efficiency: Analyze fuel consumption based on different driving speeds and conditions.
-
Sports Analysis: Calculate the average speed of athletes in events with varying pace.
-
Logistics and Transportation: Optimize routes and delivery schedules based on average speeds.
Advanced Scenarios: More Than Two Speeds
The concept extends to scenarios with more than two speeds. The same principle applies: calculate the total distance and total time, then divide to find the average speed.
Example:
Suppose you travel at 30 mph for 1 hour, 40 mph for 2 hours, and 50 mph for 3 hours.
- Distance 1: 30 mph * 1 hour = 30 miles
- Distance 2: 40 mph * 2 hours = 80 miles
- Distance 3: 50 mph * 3 hours = 150 miles
Total Distance: 30 miles + 80 miles + 150 miles = 260 miles
Total Time: 1 hour + 2 hours + 3 hours = 6 hours
Average Speed: 260 miles / 6 hours = 43.33 mph (approximately)
Conclusion
Calculating average speed accurately requires understanding that it is not simply the arithmetic mean of the individual speeds. The time spent at each speed is crucial. By applying the correct formula—Total Distance / Total Time—and paying attention to units and potential pitfalls, you can accurately calculate average speed in various scenarios, paving the way for better travel planning, improved fuel efficiency analysis, and enhanced understanding in many other areas. Remember to break down complex problems into smaller, manageable steps, focusing on calculating total distance and total time accurately before finding the final average speed.
Latest Posts
Latest Posts
-
Similarities Between A Square And A Rhombus
Mar 31, 2025
-
Coordination Of Balance And Body Movement Is Controlled By The
Mar 31, 2025
-
Why Are The Cells Generally Of A Small Size
Mar 31, 2025
-
Is Calcium Hydroxide A Base Or Acid
Mar 31, 2025
-
The Ultimate Source Of Energy From Fossil Fuels Is The
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about How To Find Average Speed With Two Speeds . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
