How To Find A Supplementary Angle
Juapaving
Apr 02, 2025 · 5 min read
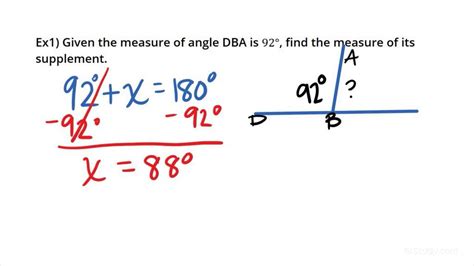
Table of Contents
How to Find a Supplementary Angle: A Comprehensive Guide
Supplementary angles are a fundamental concept in geometry, playing a crucial role in various mathematical applications. Understanding how to find supplementary angles is essential for students and professionals alike. This comprehensive guide provides a detailed explanation of supplementary angles, along with various methods and examples to help you master this concept.
What are Supplementary Angles?
Supplementary angles are two angles whose sum equals 180 degrees. They don't necessarily need to be adjacent (next to each other); they just need to add up to 180°. Think of it like two angles perfectly fitting together to form a straight line. This is a key characteristic to remember when working with supplementary angles.
Key characteristics of supplementary angles:
- Sum of 180°: The defining feature – their measures always add up to 180 degrees.
- Not necessarily adjacent: While they often appear adjacent in diagrams, this isn't a requirement. Two non-adjacent angles can still be supplementary if their sum is 180°.
- Linear pairs: A special case of supplementary angles is a linear pair. A linear pair consists of two adjacent angles that form a straight line. All linear pairs are supplementary, but not all supplementary angles are linear pairs.
Methods for Finding Supplementary Angles
Let's delve into the different methods you can use to find supplementary angles, depending on the information provided:
1. When One Angle is Known
This is the simplest scenario. If you know the measure of one angle, finding its supplement is straightforward. Simply subtract the known angle's measure from 180°.
Formula:
Supplementary Angle = 180° - Known Angle
Example:
If one angle measures 75°, its supplementary angle is:
180° - 75° = 105°
Therefore, 75° and 105° are supplementary angles.
2. Using Algebra and Equations
Problems often involve algebraic expressions representing angle measures. In such cases, you'll need to set up and solve an equation.
Example:
Two angles are supplementary. One angle is represented by 'x', and the other is twice the first angle (2x). Find the measure of each angle.
Solution:
Since the angles are supplementary, their sum is 180°:
x + 2x = 180°
Combine like terms:
3x = 180°
Solve for x:
x = 180° / 3 = 60°
Now, substitute the value of x to find the second angle:
2x = 2 * 60° = 120°
Therefore, the two supplementary angles are 60° and 120°.
3. Using Geometry and Diagrams
Many problems involve geometric shapes and diagrams. In these cases, you'll need to apply your knowledge of geometric principles alongside the concept of supplementary angles.
Example:
Consider a triangle with angles A, B, and C. If angle A is 60° and angle B is 80°, find the supplementary angle to angle C.
Solution:
The sum of angles in a triangle is always 180°. Therefore:
A + B + C = 180°
Substitute the known values:
60° + 80° + C = 180°
140° + C = 180°
C = 180° - 140° = 40°
Now, to find the supplementary angle to angle C, subtract its measure from 180°:
180° - 40° = 140°
The supplementary angle to angle C is 140°.
4. Working with Linear Pairs
Remember that linear pairs are a specific type of supplementary angles. If you're working with a linear pair, you can directly use the fact that they add up to 180°.
Example:
Two angles, angle X and angle Y, form a linear pair. If angle X measures 110°, what is the measure of angle Y?
Solution:
Since they form a linear pair, they are supplementary:
X + Y = 180°
Substitute the known value:
110° + Y = 180°
Y = 180° - 110° = 70°
Angle Y measures 70°.
Advanced Applications of Supplementary Angles
Supplementary angles aren't just a simple geometric concept; they have wide-ranging applications in various fields:
-
Trigonometry: Supplementary angles play a crucial role in understanding trigonometric identities and solving trigonometric equations. For instance, sin(x) = sin(180° - x).
-
Engineering and Architecture: Supplementary angles are essential in structural design, ensuring the stability and proper functioning of structures. Accurate angle measurements are vital in construction.
-
Computer Graphics and Animation: Understanding supplementary angles is crucial in creating realistic and accurate computer-generated images and animations. The manipulation of angles is fundamental to 3D modeling and animation.
-
Navigation: Supplementary angles are used in navigation to calculate bearings and directions. Understanding angle relationships is vital for accurate navigation.
Troubleshooting Common Mistakes
Here are some common mistakes students make when dealing with supplementary angles, and how to avoid them:
-
Confusing supplementary with complementary: Remember that complementary angles add up to 90°, while supplementary angles add up to 180°. Pay close attention to the definitions.
-
Incorrect algebraic manipulation: When solving equations involving supplementary angles, carefully follow the rules of algebra to avoid errors in calculations. Double-check your work.
-
Misinterpreting diagrams: Carefully analyze diagrams to identify which angles are supplementary. Don't assume angles are supplementary based solely on their appearance.
Practice Problems
To solidify your understanding, try these practice problems:
- Find the supplement of a 42° angle.
- Two supplementary angles are in the ratio 2:3. Find the measure of each angle.
- Angle A and Angle B are supplementary. Angle A is represented by (3x + 10)° and Angle B is (2x - 20)°. Find the value of x and the measure of each angle.
- In a quadrilateral, three angles measure 70°, 95°, and 115°. Find the measure of the fourth angle and its supplementary angle.
By working through these problems and applying the methods described above, you'll develop a strong understanding of how to find supplementary angles in various contexts. Remember, consistent practice is key to mastering this fundamental geometric concept. Don't hesitate to revisit the explanations and examples provided if you encounter any difficulties. The more you practice, the more confident you'll become in solving problems related to supplementary angles.
Latest Posts
Latest Posts
-
How Many Electrons Are In Phosphorus
Apr 03, 2025
-
Common Multiples Of 5 And 2
Apr 03, 2025
-
Choose The True Statement About The Krebs Cycle
Apr 03, 2025
-
Essay On The Pleasure Of Reading
Apr 03, 2025
-
Compare And Contrast Pulmonary Circulation With Systemic Circulation
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about How To Find A Supplementary Angle . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
