How To Find A Gcf Using Prime Factorization
Juapaving
Apr 02, 2025 · 5 min read
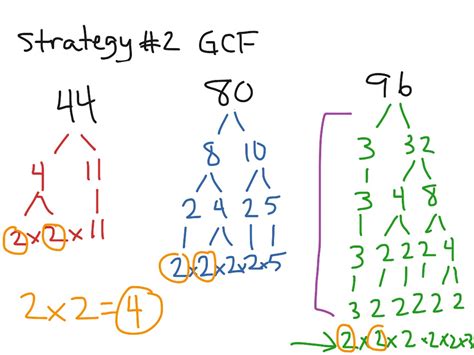
Table of Contents
How to Find the Greatest Common Factor (GCF) Using Prime Factorization
Finding the greatest common factor (GCF), also known as the greatest common divisor (GCD), is a fundamental concept in mathematics with applications ranging from simplifying fractions to solving algebraic equations. While several methods exist to determine the GCF, prime factorization stands out as a particularly insightful and reliable technique, especially when dealing with larger numbers or multiple numbers. This comprehensive guide will equip you with a thorough understanding of how to find the GCF using prime factorization, complete with practical examples and tips to enhance your understanding.
Understanding Prime Factorization
Before delving into the GCF calculation, let's solidify our understanding of prime factorization. Prime factorization is the process of expressing a number as a product of its prime factors. A prime number is a whole number greater than 1 that has only two divisors: 1 and itself (e.g., 2, 3, 5, 7, 11, etc.). Any composite number (a whole number greater than 1 that is not prime) can be uniquely expressed as a product of prime numbers.
For example:
- 12: 2 x 2 x 3 (or 2² x 3)
- 18: 2 x 3 x 3 (or 2 x 3²)
- 30: 2 x 3 x 5
- 100: 2 x 2 x 5 x 5 (or 2² x 5²)
Finding Prime Factors: A Step-by-Step Approach
Several methods exist for finding the prime factors of a number. One common approach involves repeatedly dividing the number by the smallest prime number that divides it evenly until you reach 1.
Let's illustrate this with an example: Find the prime factorization of 72.
- Start with the smallest prime number, 2: 72 ÷ 2 = 36
- Continue dividing by 2: 36 ÷ 2 = 18
- Continue dividing by 2: 18 ÷ 2 = 9
- Now, 2 doesn't divide 9 evenly. Move to the next prime number, 3: 9 ÷ 3 = 3
- Finally, divide by 3: 3 ÷ 3 = 1
Therefore, the prime factorization of 72 is 2 x 2 x 2 x 3 x 3, or 2³ x 3².
Calculating the GCF Using Prime Factorization
Once you have the prime factorization of each number, finding the GCF becomes straightforward. The GCF is the product of the lowest powers of all common prime factors.
Let's illustrate this with an example: Find the GCF of 12 and 18.
- Prime factorization of 12: 2² x 3
- Prime factorization of 18: 2 x 3²
Both 12 and 18 share the prime factors 2 and 3. The lowest power of 2 is 2¹ (or simply 2), and the lowest power of 3 is 3¹.
Therefore, the GCF of 12 and 18 is 2 x 3 = 6.
Working with Multiple Numbers
The method extends seamlessly to finding the GCF of more than two numbers. Let's find the GCF of 24, 36, and 60.
- Prime factorization of 24: 2³ x 3
- Prime factorization of 36: 2² x 3²
- Prime factorization of 60: 2² x 3 x 5
Identifying common prime factors: All three numbers share 2 and 3. The lowest power of 2 is 2², and the lowest power of 3 is 3¹.
Therefore, the GCF of 24, 36, and 60 is 2² x 3 = 4 x 3 = 12.
Advanced Examples and Troubleshooting
Let's tackle some more complex scenarios to solidify our understanding.
Example 1: Finding the GCF of numbers with no common prime factors.
Consider the numbers 15 (3 x 5) and 28 (2² x 7). These numbers share no common prime factors. In such cases, the GCF is 1. Numbers that have a GCF of 1 are called relatively prime or coprime.
Example 2: Dealing with large numbers.
Finding the prime factors of large numbers can be time-consuming. However, the process remains the same. For instance, let’s find the GCF of 360 and 540.
- Prime factorization of 360: 2³ x 3² x 5
- Prime factorization of 540: 2² x 3³ x 5
Common prime factors: 2, 3, and 5. The lowest powers are 2², 3², and 5¹.
Therefore, the GCF of 360 and 540 is 2² x 3² x 5 = 4 x 9 x 5 = 180
Example 3: Numbers with many factors.
Numbers with numerous factors might appear daunting, but the approach remains consistent. Let's find the GCF of 720, 1080, and 1440.
- Prime factorization of 720: 2⁴ x 3² x 5
- Prime factorization of 1080: 2³ x 3³ x 5
- Prime factorization of 1440: 2⁵ x 3² x 5
Common prime factors are 2, 3, and 5. The lowest powers are 2³, 3², and 5¹.
Therefore, the GCF of 720, 1080, and 1440 is 2³ x 3² x 5 = 8 x 9 x 5 = 360.
Applications of Finding the GCF
The ability to find the GCF is crucial in various mathematical contexts:
-
Simplifying Fractions: To simplify a fraction to its lowest terms, divide both the numerator and the denominator by their GCF. For example, simplifying 18/24 involves finding the GCF of 18 and 24 (which is 6). Dividing both by 6 yields the simplified fraction 3/4.
-
Solving Algebraic Equations: GCF plays a critical role in factoring algebraic expressions. Finding the GCF of the terms in an expression allows you to factor it, which is essential for solving many algebraic equations.
-
Word Problems: Many word problems involving quantities require finding the GCF to solve them effectively. This is particularly true in problems involving grouping or dividing items into equal groups.
-
Geometry: GCF can be used to solve problems related to finding the dimensions of the largest square that can be used to tile a rectangular area.
Conclusion
Prime factorization provides a robust and reliable method for finding the greatest common factor of two or more numbers. While seemingly simple for small numbers, the method remains efficient and effective even when dealing with large numbers or multiple numbers. Mastering this technique is essential for simplifying fractions, factoring algebraic expressions, and solving a wide array of mathematical problems. By systematically applying the steps outlined above, you'll confidently navigate the world of GCF calculations, enhancing your mathematical proficiency and problem-solving skills. Remember to practice regularly to refine your skills and gain a deeper understanding of this fundamental mathematical concept.
Latest Posts
Latest Posts
-
96 Inches Is How Many Feet
Apr 03, 2025
-
Kg M 3 To G Cm3
Apr 03, 2025
-
120 Cm Is How Many Inches
Apr 03, 2025
-
The Scattering Of Light By A Colloid Is Called The
Apr 03, 2025
-
Roots Forming A Number In Logo
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about How To Find A Gcf Using Prime Factorization . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
