How Many Lines Of Symmetry Does H Have
Juapaving
May 09, 2025 · 5 min read
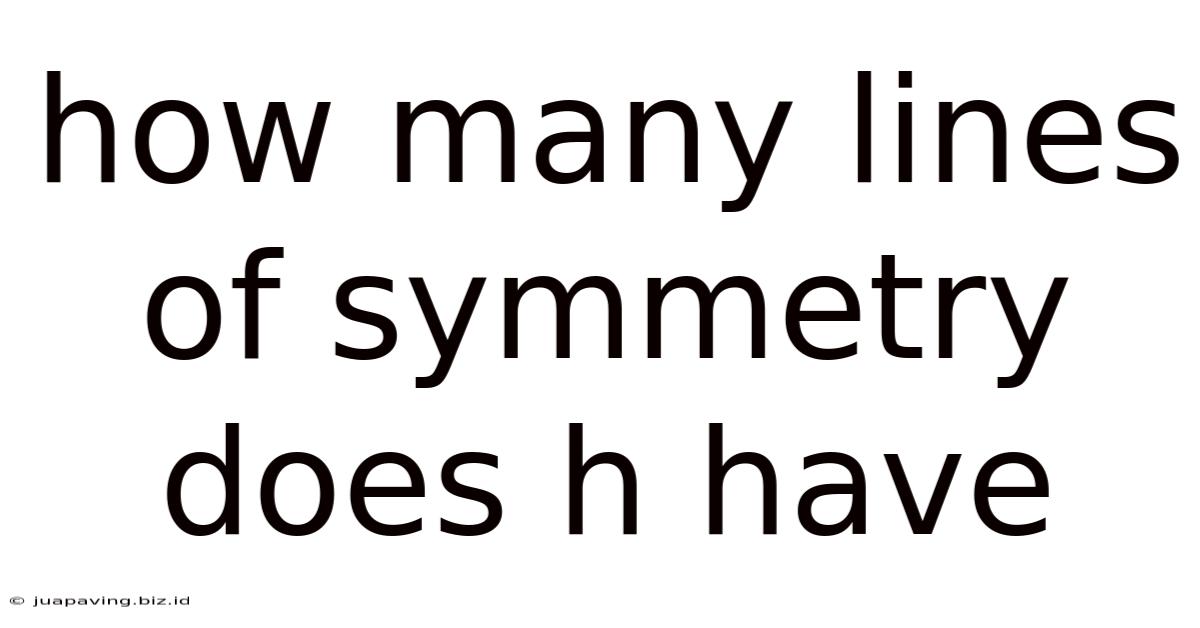
Table of Contents
How Many Lines of Symmetry Does the Letter 'H' Have? A Deep Dive into Symmetry
The seemingly simple question, "How many lines of symmetry does the letter 'H' have?" opens a fascinating exploration into the world of geometry, specifically, lines of symmetry and reflectional symmetry. While the answer might seem immediately obvious to some, a deeper understanding requires delving into the precise definition of symmetry and its application to various shapes and figures, including letters of the alphabet. This comprehensive article will not only answer the central question but also explore related concepts, providing a solid foundation in geometrical symmetry.
Understanding Lines of Symmetry
Before we tackle the letter 'H', let's define the key concept: a line of symmetry, also known as a line of reflection or axis of symmetry. A line of symmetry is a line that divides a shape into two identical halves that are mirror images of each other. If you were to fold the shape along the line of symmetry, the two halves would perfectly overlap. This type of symmetry is formally known as reflectional symmetry or bilateral symmetry.
It's crucial to note that not all shapes possess lines of symmetry. Some shapes might have one, others several, and some might have none at all. The number of lines of symmetry depends entirely on the shape's form and characteristics. Consider a circle, for instance. A circle has an infinite number of lines of symmetry, as any line passing through its center will divide it into two identical halves. In contrast, a scalene triangle (a triangle with all sides of different lengths) has no lines of symmetry.
Analyzing the Letter 'H' for Symmetry
Now, let's focus on the uppercase letter 'H'. The typical representation of 'H' consists of two parallel vertical lines connected by a shorter horizontal line in the middle. To determine the number of lines of symmetry, we need to systematically examine potential lines that could divide the 'H' into mirror-image halves.
The Vertical Line of Symmetry
The most obvious line of symmetry for the letter 'H' is the vertical line running exactly down the center. If you were to fold the letter along this line, the left and right halves would perfectly overlap. This is a clear and undeniable line of symmetry.
The Horizontal Line of Symmetry?
Let's consider whether a horizontal line could also serve as a line of symmetry. If we draw a horizontal line through the middle of the letter 'H', the top and bottom parts are not mirror images of each other. The top part consists of two separate vertical lines, while the bottom is a single horizontal line connecting them. Therefore, a horizontal line does not represent a line of symmetry for the letter 'H'.
Diagonal Lines of Symmetry?
Are there any diagonal lines of symmetry? Let's analyze this possibility. No matter what diagonal line we attempt to draw through the letter 'H', we cannot divide it into two identical, mirror-image halves. The shapes formed on either side of any diagonal line are distinctly different. Thus, no diagonal lines act as lines of symmetry.
The Final Verdict: How Many Lines of Symmetry Does 'H' Have?
Based on our comprehensive analysis, the letter 'H' possesses only one line of symmetry: the vertical line passing through its center. There are no horizontal or diagonal lines that satisfy the condition of dividing the 'H' into perfectly overlapping mirror-image halves.
Expanding the Concept: Symmetry in Different Contexts
The exploration of symmetry in the letter 'H' provides a springboard for understanding its broader applications. Symmetry is a fundamental concept in various fields:
1. Mathematics and Geometry:
Symmetry is a crucial aspect of many mathematical concepts, including transformations, group theory, and fractals. Understanding symmetry allows mathematicians to classify and analyze shapes and patterns efficiently.
2. Science and Nature:
Symmetry is ubiquitous in the natural world. From the bilateral symmetry of animals (including humans!) to the radial symmetry of flowers and snowflakes, nature showcases symmetry in diverse forms. This symmetry often reflects underlying biological or physical principles. The study of symmetry helps scientists understand the underlying patterns and structures in nature.
3. Art and Design:
Artists and designers have long utilized symmetry to create aesthetically pleasing and balanced compositions. Symmetrical designs evoke a sense of harmony and order, while deviations from symmetry can create dynamism and visual interest.
4. Architecture:
Symmetrical designs are frequently seen in architecture. The balanced proportions and visual harmony they offer contribute to the aesthetic appeal and structural integrity of buildings. Many famous buildings, from ancient temples to modern skyscrapers, demonstrate the artistic and functional uses of symmetry.
Beyond the Basic: Exploring Other Types of Symmetry
While we've primarily focused on reflectional symmetry, it's important to know that other types of symmetry exist:
-
Rotational Symmetry: A shape possesses rotational symmetry if it can be rotated less than 360 degrees about a central point and still look identical to its original position. A square, for instance, has rotational symmetry of order 4, meaning it can be rotated four times (90 degrees each time) and still appear unchanged.
-
Translational Symmetry: This type of symmetry is found in patterns that repeat themselves regularly along a line or in a plane. Wallpaper patterns are a prime example of translational symmetry.
-
Scale Symmetry: This type of symmetry deals with shapes that can be scaled up or down without changing their overall shape. Fractals are well-known examples of objects exhibiting scale symmetry.
Conclusion: The Power of Symmetry
The seemingly simple question regarding the lines of symmetry in the letter 'H' has led us on a journey into the fascinating world of geometric symmetry. We've learned that the 'H' possesses only one line of symmetry, a vertical line running through its center. However, the exploration extends far beyond this specific case. Understanding the different types of symmetry and their applications in various fields provides a deeper appreciation of the mathematical principles that underlie the order and beauty of the world around us. Symmetry is a fundamental concept that permeates art, science, and nature, demonstrating its importance in understanding patterns and structures across diverse domains. The analysis of the simple letter 'H' has opened a gateway to the profound and elegant world of symmetry.
Latest Posts
Latest Posts
-
What Is The Prime Factorization Of 86
May 09, 2025
-
What Is The Lcm For 36 And 45
May 09, 2025
-
Moment Of Inertia For Rectangular Cross Section
May 09, 2025
-
When The Price Of A Good Increases
May 09, 2025
-
What Is The Percentage Of 6 5
May 09, 2025
Related Post
Thank you for visiting our website which covers about How Many Lines Of Symmetry Does H Have . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.