Find The Mean Proportional Between 3 And 27
Juapaving
Apr 02, 2025 · 5 min read
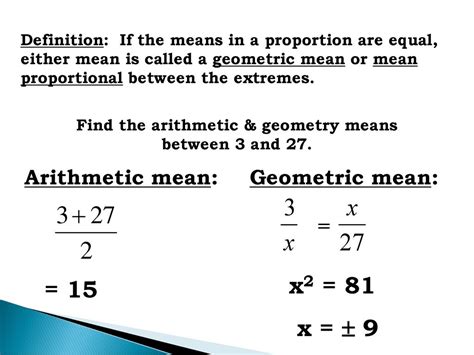
Table of Contents
Find the Mean Proportional Between 3 and 27: A Deep Dive into Proportions and Geometric Means
Finding the mean proportional between two numbers might sound like a niche mathematical problem, but understanding this concept unlocks a deeper appreciation for proportions, geometric means, and their applications across various fields. This article will not only show you how to find the mean proportional between 3 and 27 but will also explore the underlying mathematical principles, delve into practical applications, and offer insightful examples to solidify your understanding.
Understanding Proportions and Mean Proportionals
Before tackling the specific problem, let's establish a firm grasp of the fundamental concepts. A proportion is a statement that two ratios are equal. For example, 1:2 = 3:6 is a proportion because both ratios simplify to 1/2. In general, a proportion can be expressed as a/b = c/d.
Now, let's introduce the mean proportional. Given two numbers, 'a' and 'b', the mean proportional, often denoted as 'x', is a number such that the ratio of 'a' to 'x' is equal to the ratio of 'x' to 'b'. This can be written as:
a/x = x/b
This equation highlights the core concept: the mean proportional acts as a bridge, maintaining a consistent ratio between the two given numbers. Solving for 'x', we get:
x² = ab x = √(ab)
This formula shows that the mean proportional is simply the square root of the product of the two given numbers. This is also known as the geometric mean.
Finding the Mean Proportional Between 3 and 27
Now, armed with the theoretical understanding, let's solve the problem at hand: finding the mean proportional between 3 and 27. Using the formula derived above:
x = √(ab) = √(3 * 27) = √81 = 9
Therefore, the mean proportional between 3 and 27 is 9. We can verify this by checking the proportion:
3/9 = 9/27 which simplifies to 1/3 = 1/3. The proportion holds true.
Deeper Dive: Geometric Mean vs. Arithmetic Mean
It's crucial to distinguish between the geometric mean (mean proportional) and the arithmetic mean. The arithmetic mean is simply the average, calculated by adding the numbers and dividing by the count. For 3 and 27, the arithmetic mean is (3 + 27)/2 = 15.
The key difference lies in how the means are calculated and their implications. The arithmetic mean is sensitive to outliers; a single large number can significantly skew the average. The geometric mean, on the other hand, is less sensitive to extreme values and is particularly useful when dealing with multiplicative relationships, such as growth rates or ratios.
When to Use the Geometric Mean
The geometric mean finds applications in numerous fields:
-
Finance: Calculating average investment returns over multiple periods, especially when dealing with fluctuating returns. The geometric mean provides a more accurate representation than the arithmetic mean in these scenarios.
-
Statistics: In situations where data is skewed, the geometric mean offers a more robust measure of central tendency than the arithmetic mean.
-
Geometry: The geometric mean is inherently linked to geometric concepts, such as similar triangles and finding the length of segments. The mean proportional is directly related to problems involving similar figures.
-
Science and Engineering: Various scientific and engineering applications use the geometric mean to analyze data exhibiting multiplicative relationships, such as rates of change or growth factors.
-
Data Analysis: For datasets where values span orders of magnitude, the geometric mean provides a more representative measure of central tendency than the arithmetic mean, which can be heavily influenced by extreme values.
Illustrative Examples: Applying the Mean Proportional
Let's explore a few examples to further illustrate the practical application of the mean proportional:
Example 1: Investment Growth
Suppose an investment grows by 10% in the first year and 20% in the second year. To find the average annual growth rate, we use the geometric mean. We have growth factors of 1.1 (1 + 0.1) and 1.2 (1 + 0.2). The geometric mean is √(1.1 * 1.2) ≈ 1.1489. This means the average annual growth rate is approximately 14.89%.
Example 2: Similar Triangles
Consider two similar triangles. If the corresponding sides of the triangles have lengths 'a' and 'b', then the length of the altitude to the corresponding sides is the mean proportional between 'a' and 'b'.
Example 3: Geometric Progression
The mean proportional represents the middle term in a geometric progression. For instance, the sequence 3, 9, 27 is a geometric progression, and 9 is the mean proportional between 3 and 27. The ratio between consecutive terms remains constant (in this case, it's 3).
Expanding the Concept: Beyond Two Numbers
While we've focused on finding the mean proportional between two numbers, the concept can be extended to more than two numbers. The geometric mean of 'n' numbers is calculated by multiplying the numbers and taking the 'n'th root of the product. This broader perspective offers even greater versatility in various applications.
Conclusion: Mastering Mean Proportionals
Understanding the mean proportional, or geometric mean, is essential for comprehending proportions and their applications in diverse fields. It's not merely an abstract mathematical concept but a powerful tool for solving real-world problems across finance, statistics, geometry, and beyond. This article has provided a comprehensive overview, starting with the fundamental definition and progressing through practical examples and applications. By mastering this concept, you'll enhance your analytical capabilities and gain a deeper understanding of multiplicative relationships within data and geometric structures. Remember, the mean proportional, while seemingly simple, provides a crucial bridge between numbers, ratios, and their practical implications.
Latest Posts
Latest Posts
-
An Npn Transistor Is Made Up Of
Apr 03, 2025
-
How Tall Is 48 Inches In Ft
Apr 03, 2025
-
What Is A Strait In Geography
Apr 03, 2025
-
Which Of The Following Is A Multiple Of 2
Apr 03, 2025
-
Examples Of Elements In Everyday Life
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about Find The Mean Proportional Between 3 And 27 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
