Find Discriminant Of Quadratic Equation Calculator
Juapaving
Mar 27, 2025 · 5 min read
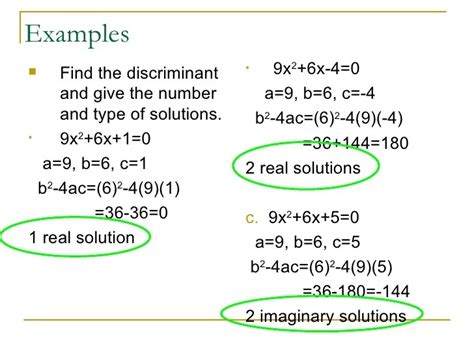
Table of Contents
Find Discriminant of Quadratic Equation Calculator: A Comprehensive Guide
The quadratic equation, a cornerstone of algebra, often presents itself in various mathematical and real-world applications. Understanding its properties, particularly the discriminant, is crucial for solving and interpreting its solutions. This comprehensive guide delves into the concept of the discriminant, its significance, and how to efficiently calculate it using readily available online tools – discriminant of quadratic equation calculators. We'll explore different methods, tackle example problems, and unravel the mysteries behind this fundamental algebraic concept.
What is a Quadratic Equation?
Before diving into the discriminant, let's refresh our understanding of quadratic equations. A quadratic equation is a polynomial equation of the second degree, meaning the highest power of the variable (usually 'x') is 2. Its general form is represented as:
ax² + bx + c = 0
where:
- a, b, and c are constants (real numbers), and
- a ≠ 0 (if a were 0, it would no longer be a quadratic equation).
Introducing the Discriminant
The discriminant, denoted by the Greek letter Δ (delta), is a crucial part of the quadratic formula. It provides invaluable information about the nature of the roots (solutions) of a quadratic equation. It's calculated using the coefficients a, b, and c from the standard quadratic equation form:
Δ = b² - 4ac
The value of the discriminant directly influences the type of solutions the quadratic equation possesses:
1. Δ > 0 (Discriminant is positive):
- The quadratic equation has two distinct real roots. This means there are two different real numbers that satisfy the equation. Graphically, this corresponds to the parabola intersecting the x-axis at two distinct points.
2. Δ = 0 (Discriminant is zero):
- The quadratic equation has one real root (repeated root). This means there's only one real number that satisfies the equation. Graphically, the parabola touches the x-axis at a single point – its vertex.
3. Δ < 0 (Discriminant is negative):
- The quadratic equation has two distinct complex roots (conjugate pairs). These roots are complex numbers involving the imaginary unit 'i' (√-1). Graphically, the parabola does not intersect the x-axis at all.
Why is the Discriminant Important?
The discriminant's importance stems from its ability to quickly determine the characteristics of a quadratic equation's solutions without actually solving the equation. This is invaluable for:
- Understanding the nature of solutions: Knowing whether the roots are real, complex, or repeated allows for a deeper understanding of the equation's behavior.
- Problem-solving efficiency: Determining the type of roots beforehand can significantly simplify the solution process, potentially avoiding unnecessary calculations.
- Applications in various fields: The discriminant plays a role in various fields, including physics, engineering, and economics, where understanding the nature of solutions is crucial for interpreting results.
Using a Discriminant Calculator
Manually calculating the discriminant is straightforward, but using a discriminant of quadratic equation calculator offers several advantages:
- Speed and Accuracy: Calculators provide instant and accurate results, minimizing the risk of calculation errors.
- Convenience: They eliminate the need for manual calculations, saving time and effort.
- Accessibility: Online calculators are readily available and accessible from any device with an internet connection.
- Ease of use: Most calculators have a user-friendly interface, requiring only the input of coefficients a, b, and c.
Step-by-Step Guide to Using a Discriminant Calculator (Hypothetical Example)
Let's assume you have the quadratic equation: 3x² - 7x + 2 = 0
-
Identify the coefficients: From the equation, we have a = 3, b = -7, and c = 2.
-
Input the coefficients: Most online calculators have input fields for a, b, and c. Enter these values into the respective fields.
-
Calculate the discriminant: Click the "Calculate" or equivalent button. The calculator will compute the discriminant (Δ) using the formula: Δ = b² - 4ac.
-
Interpret the result: The calculator will display the calculated value of Δ. Based on the value, you can determine the nature of the roots as described earlier (Δ > 0, Δ = 0, or Δ < 0).
Example Problems
Let's illustrate the process with a few examples:
Example 1:
Find the discriminant of the quadratic equation: x² - 4x + 4 = 0
- a = 1, b = -4, c = 4
- Δ = (-4)² - 4(1)(4) = 16 - 16 = 0
- Interpretation: Since Δ = 0, the equation has one real root (repeated root).
Example 2:
Find the discriminant of the quadratic equation: 2x² + 5x + 2 = 0
- a = 2, b = 5, c = 2
- Δ = (5)² - 4(2)(2) = 25 - 16 = 9
- Interpretation: Since Δ > 0, the equation has two distinct real roots.
Example 3:
Find the discriminant of the quadratic equation: x² + x + 1 = 0
- a = 1, b = 1, c = 1
- Δ = (1)² - 4(1)(1) = 1 - 4 = -3
- Interpretation: Since Δ < 0, the equation has two distinct complex roots.
Beyond the Calculator: Understanding the Quadratic Formula
While discriminant calculators provide a quick solution, it's crucial to understand the complete quadratic formula, which is derived from completing the square:
x = (-b ± √Δ) / 2a
The discriminant (√Δ) is a part of this formula. The ± symbol indicates that there are two possible solutions for x, corresponding to the two roots. The nature of the roots (real, complex, repeated) is determined by the value of Δ, as explained earlier.
Conclusion
The discriminant of a quadratic equation is a powerful tool for quickly assessing the characteristics of its solutions. While manual calculations are straightforward, using online discriminant of quadratic equation calculators provides efficiency and accuracy. Understanding the discriminant's significance goes beyond mere calculation; it's about gaining a deeper insight into the behavior and solutions of quadratic equations – a fundamental concept with far-reaching applications across numerous fields. By mastering both the theoretical understanding and the practical application of calculators, you equip yourself with essential skills for tackling various mathematical challenges. Remember to always check your work and understand the underlying principles, even when using technology to assist in your calculations.
Latest Posts
Latest Posts
-
What Is The Least Common Multiple Of 7 14
Mar 30, 2025
-
What Is The Lcm Of 2 And 10
Mar 30, 2025
-
Why Is Water Considered To Be A Renewable Resource
Mar 30, 2025
-
Find The Area Under The Curve Calculator
Mar 30, 2025
-
Is Butter Melting A Physical Change
Mar 30, 2025
Related Post
Thank you for visiting our website which covers about Find Discriminant Of Quadratic Equation Calculator . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
