Derivation Of Van Der Waals Equation
Juapaving
Apr 03, 2025 · 7 min read
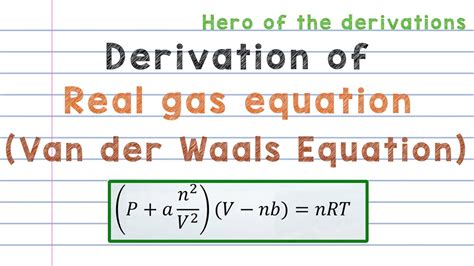
Table of Contents
Derivation of the Van der Waals Equation: A Deep Dive
The ideal gas law, PV = nRT, provides a simplified model for gas behavior. However, it fails to accurately describe real gases, particularly at high pressures and low temperatures. Real gases deviate from ideality due to two primary factors: intermolecular forces and the finite volume occupied by gas molecules themselves. The van der Waals equation incorporates these factors to provide a more realistic description of real gas behavior. This article delves into the derivation of this crucial equation, exploring the underlying physical principles and mathematical steps involved.
Understanding the Limitations of the Ideal Gas Law
The ideal gas law assumes that:
- Gas molecules occupy negligible volume: This implies that the volume available for the gas molecules to move is the entire volume of the container.
- There are no intermolecular forces: This means that gas molecules do not interact with each other, neither attracting nor repelling.
These assumptions break down at high pressures and low temperatures.
- High pressures: At high pressures, the gas molecules are compressed closer together. Their finite volumes become significant compared to the total volume of the container, reducing the space available for movement.
- Low temperatures: At low temperatures, the kinetic energy of the gas molecules decreases, making intermolecular forces more prominent. These attractive forces cause the gas molecules to cluster together, reducing the pressure exerted on the container walls.
Incorporating Intermolecular Forces and Finite Volume: The Van der Waals Equation
The van der Waals equation modifies the ideal gas law to account for the finite volume of gas molecules and the attractive forces between them. It is expressed as:
(P + a(n/V)²)(V - nb) = nRT
Where:
- P is the pressure of the gas
- V is the volume of the gas
- n is the number of moles of gas
- R is the ideal gas constant
- T is the temperature of the gas
- a is a constant that accounts for the strength of the intermolecular attractive forces
- b is a constant that accounts for the volume excluded by the gas molecules
Let's break down the derivation of each term:
Deriving the Correction for Intermolecular Forces: The 'a(n/V)²' Term
The attractive forces between gas molecules reduce the pressure exerted on the container walls. This is because molecules near the wall experience a net inward force, reducing their impact on the wall. The magnitude of this reduction is proportional to the square of the molar density (n/V)². Why squared? Because the probability of a molecule interacting with another is proportional to the concentration of molecules. A higher concentration increases the likelihood of interactions.
The reduction in pressure is therefore proportional to (n/V)². To incorporate this into the ideal gas law, we add a correction term to the observed pressure:
P<sub>observed</sub> = P<sub>ideal</sub> - a(n/V)²
Where:
- P<sub>observed</sub> is the experimentally measured pressure
- P<sub>ideal</sub> is the pressure predicted by the ideal gas law
- a(n/V)² is the correction term accounting for intermolecular forces. The constant 'a' reflects the strength of these attractive forces; higher 'a' values indicate stronger attractions.
This leads to the modified pressure term in the van der Waals equation: (P + a(n/V)²)
Deriving the Correction for Finite Volume: The 'nb' Term
Real gas molecules occupy a finite volume. This reduces the volume available for the gas molecules to move. The excluded volume isn't simply the sum of the volumes of individual molecules, as this ignores the fact that molecules cannot overlap. A more accurate approach involves considering the effective volume excluded by a molecule. This is often approximated as four times the actual volume of a single molecule.
The total excluded volume is then given by nb, where 'b' is the excluded volume per mole of gas. This is subtracted from the total volume to account for the unavailable space:
V<sub>available</sub> = V - nb
This corrected volume is then used in the van der Waals equation.
Combining the Corrections: The Complete Van der Waals Equation
Combining the pressure correction and the volume correction, we obtain the complete van der Waals equation:
(P + a(n/V)²)(V - nb) = nRT
This equation provides a significantly improved description of real gas behavior compared to the ideal gas law. The values of 'a' and 'b' are specific to each gas and are experimentally determined or estimated using molecular parameters.
Significance of the Van der Waals Constants (a and b)
The constants a and b are crucial parameters in the Van der Waals equation, each reflecting a specific aspect of intermolecular interactions and molecular size. Let's delve deeper into their significance:
Understanding the 'a' Constant (Intermolecular Forces)
The constant a quantifies the strength of the attractive intermolecular forces within the gas. A larger value of a indicates stronger attractive forces. These forces arise from various sources, including dipole-dipole interactions, London dispersion forces, and hydrogen bonding.
-
Larger 'a' values: These are characteristic of gases with strong intermolecular attractions. This leads to significant deviations from ideality at moderate pressures. The gas will exhibit lower pressures than predicted by the ideal gas law because the attractive forces pull the molecules together, reducing their impact on the container walls.
-
Smaller 'a' values: These are observed in gases with weaker intermolecular attractions. These gases show less deviation from ideality, behaving more like ideal gases. The attractive forces are relatively weak, thus having a smaller impact on the observed pressure.
The magnitude of 'a' provides insights into the nature of the intermolecular forces at play within the gas.
Understanding the 'b' Constant (Excluded Volume)
The constant b accounts for the finite volume of the gas molecules. It represents the excluded volume per mole of gas, essentially the volume unavailable to other molecules due to the presence of a given molecule. This is not simply the volume of the molecules themselves because it accounts for the fact that the molecules cannot occupy the same space.
-
Larger 'b' values: These are associated with larger gas molecules. Gases with larger molecules occupy a greater volume, leading to a significant reduction in the available volume for molecular movement. This results in higher pressures than predicted by the ideal gas law.
-
Smaller 'b' values: These gases have smaller molecules, resulting in less excluded volume and minimal deviation from the ideal gas law in terms of volume.
The values of 'a' and 'b' are empirically determined and tabulated for various gases. Their values offer crucial insights into the molecular properties and intermolecular interactions of the gases they represent.
Applications and Limitations of the Van der Waals Equation
The van der Waals equation is extensively used in various applications, including:
-
Predicting gas behavior: It provides more accurate predictions of pressure, volume, and temperature relationships for real gases compared to the ideal gas law, especially at high pressures and low temperatures.
-
Modeling phase transitions: It can be used to predict and understand phase transitions, such as the liquefaction of gases.
-
Chemical engineering: It is employed in chemical engineering calculations, particularly those involving high-pressure systems.
However, the van der Waals equation has its limitations:
-
Simplicity: It is a relatively simple model that does not account for all aspects of intermolecular interactions. More complex equations of state exist for situations requiring greater accuracy.
-
Critical point prediction limitations: While it can predict the existence of a critical point (the point beyond which a gas cannot be liquefied), its predictions for the critical parameters (temperature, pressure, and volume) may not always be very precise.
-
Non-polar gases primarily: It works best for non-polar gases. For polar gases, the equation may not be as accurate because it doesn't explicitly account for the directional nature of dipole-dipole interactions.
Despite these limitations, the van der Waals equation remains a valuable tool for understanding and modeling the behavior of real gases, providing a significant improvement over the ideal gas law. Its simplicity and conceptual clarity make it a fundamental concept in thermodynamics and physical chemistry. It serves as a stepping stone towards understanding more complex equations of state.
Latest Posts
Latest Posts
-
How Many Feet Is 400 Yards
Apr 04, 2025
-
What Would Be The Major Product Of The Following Reaction
Apr 04, 2025
-
True False All Flowers Contain Male And Female Parts
Apr 04, 2025
-
What Colour Does Litmus Paper Turn In Acid
Apr 04, 2025
-
Are Coal And Charcoal The Same Thing
Apr 04, 2025
Related Post
Thank you for visiting our website which covers about Derivation Of Van Der Waals Equation . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
