Common Multiples Of 10 And 15
Juapaving
Mar 27, 2025 · 5 min read
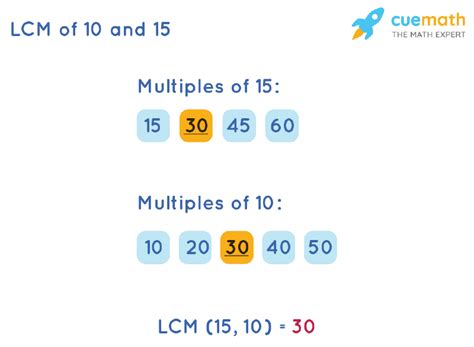
Table of Contents
Unveiling the Secrets of Common Multiples: A Deep Dive into Multiples of 10 and 15
Finding common multiples might seem like a simple arithmetic task, but understanding the underlying concepts and exploring various methods to identify them opens up a fascinating world of number theory. This in-depth article delves into the common multiples of 10 and 15, exploring different approaches to find them, understanding their significance, and uncovering some intriguing patterns. We'll move beyond the basics, touching upon real-world applications and advanced concepts.
Understanding Multiples
Before we dive into the specifics of common multiples of 10 and 15, let's solidify our understanding of the fundamental concept of multiples.
A multiple of a number is the result of multiplying that number by any integer (whole number). For example:
- Multiples of 10: 10, 20, 30, 40, 50, 60, 70, 80, 90, 100... and so on. These are all obtained by multiplying 10 by 1, 2, 3, 4... and so forth.
- Multiples of 15: 15, 30, 45, 60, 75, 90, 105, 120... and so on. These are the results of multiplying 15 by 1, 2, 3, 4... and continuing the pattern.
Notice that some numbers appear in both lists. These are the common multiples of 10 and 15.
Identifying Common Multiples of 10 and 15: Method 1 - Listing Multiples
The most straightforward method to find common multiples is by listing the multiples of each number and identifying the overlaps. Let's do this for 10 and 15:
Multiples of 10: 10, 20, 30, 40, 50, 60, 70, 80, 90, 100, 110, 120, 130, 140, 150...
Multiples of 15: 15, 30, 45, 60, 75, 90, 105, 120, 135, 150...
By comparing the two lists, we can easily see that some numbers appear in both sequences. These are the common multiples: 30, 60, 90, 120, 150... This method is effective for smaller numbers but becomes cumbersome for larger numbers or when dealing with more than two numbers.
Identifying Common Multiples of 10 and 15: Method 2 - Prime Factorization
A more efficient and elegant approach involves using prime factorization. Prime factorization is the process of breaking down a number into its prime factors (numbers divisible only by 1 and themselves).
- Prime factorization of 10: 2 x 5
- Prime factorization of 15: 3 x 5
To find the least common multiple (LCM), we take the highest power of each prime factor present in either factorization and multiply them together.
- LCM(10, 15): 2 x 3 x 5 = 30
The LCM is the smallest number that is a multiple of both 10 and 15. All other common multiples are multiples of the LCM. Therefore, the common multiples of 10 and 15 are 30, 60, 90, 120, 150, and so on. This method is significantly more efficient, especially when dealing with larger numbers.
Understanding the Least Common Multiple (LCM)
The least common multiple (LCM) is the smallest positive number that is a multiple of two or more numbers. In our case, the LCM of 10 and 15 is 30. Understanding the LCM is crucial because it forms the foundation for finding all other common multiples. Once you have the LCM, you can find all other common multiples by multiplying the LCM by consecutive integers (1, 2, 3, 4...).
Finding All Common Multiples: A Formulaic Approach
We can express the common multiples of 10 and 15 using a formula:
Common Multiples = LCM(10, 15) * n, where 'n' is any positive integer (1, 2, 3, 4...).
Since LCM(10, 15) = 30, the formula becomes:
Common Multiples = 30n
Substituting different values for 'n' gives us all the common multiples:
- n = 1: 30
- n = 2: 60
- n = 3: 90
- n = 4: 120
- n = 5: 150
- and so on...
This formula provides a systematic and efficient way to generate all common multiples.
Real-World Applications of Finding Common Multiples
Finding common multiples isn't just an abstract mathematical exercise; it has practical applications in various real-world scenarios:
-
Scheduling: Imagine you need to schedule two events, one that occurs every 10 days and another that occurs every 15 days. To find when both events coincide, you need to find the common multiples of 10 and 15. The first time they coincide is in 30 days (the LCM).
-
Fractions: Finding the LCM is essential when adding or subtracting fractions. The LCM of the denominators is used to find a common denominator, simplifying the calculation.
-
Measurement and Construction: In construction or engineering, materials might come in lengths of 10 units and 15 units. Finding common multiples helps determine the most efficient cutting strategy to minimize waste.
-
Cyclic Patterns: Many natural phenomena exhibit cyclical patterns. Understanding common multiples can help predict when these cycles align.
Advanced Concepts: Greatest Common Divisor (GCD) and Relationship with LCM
The greatest common divisor (GCD) is the largest number that divides both numbers without leaving a remainder. The GCD of 10 and 15 is 5. There's an interesting relationship between the LCM and the GCD:
LCM(a, b) * GCD(a, b) = a * b
For 10 and 15:
LCM(10, 15) * GCD(10, 15) = 30 * 5 = 150
10 * 15 = 150
This relationship provides another way to calculate the LCM if the GCD is known.
Exploring Patterns and Properties of Common Multiples
Observing the common multiples of 10 and 15 reveals some interesting patterns:
-
Arithmetic Progression: The common multiples form an arithmetic progression with a common difference equal to the LCM (30).
-
Divisibility: All common multiples are divisible by both 10 and 15.
-
Infinite Set: There is an infinite number of common multiples for any two numbers.
Conclusion: Mastering the Art of Finding Common Multiples
Finding common multiples, particularly those of 10 and 15, is a fundamental concept in mathematics with far-reaching applications. This article explored various methods for identifying common multiples, from simple listing to the more efficient prime factorization technique. We emphasized the importance of the least common multiple (LCM) and its role in generating all common multiples. Understanding these concepts not only enhances mathematical skills but also provides valuable tools for tackling real-world problems involving scheduling, fractions, measurements, and cyclical patterns. By mastering the art of finding common multiples, you unlock a deeper appreciation for the intricate relationships between numbers and their practical applications.
Latest Posts
Latest Posts
-
Is 6 A Factor Of 12
Mar 30, 2025
-
If What Is The Value Of
Mar 30, 2025
-
Adjectives That Start With A B
Mar 30, 2025
-
How Many Neutrons In Carbon 14
Mar 30, 2025
-
What Is The Lowest Common Multiple Of 5 And 10
Mar 30, 2025
Related Post
Thank you for visiting our website which covers about Common Multiples Of 10 And 15 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
