Common Denominator Of 8 And 3
Juapaving
Mar 30, 2025 · 5 min read
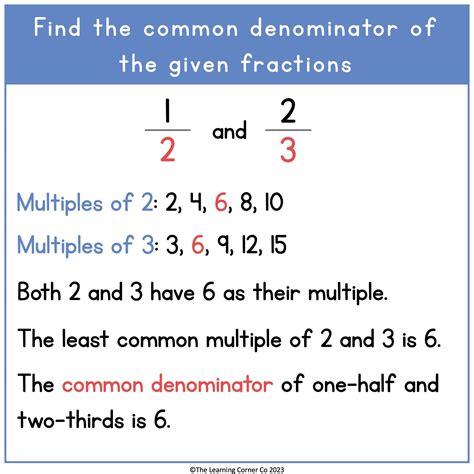
Table of Contents
Unveiling the Common Denominator: A Deep Dive into the Concept of 8 and 3
Finding the common denominator of 8 and 3 might seem like a simple arithmetic task, but it opens the door to a deeper understanding of fundamental mathematical concepts crucial for various applications. This article will delve into the process of finding the common denominator, exploring different methods and demonstrating their practical significance. We'll also touch upon related concepts like least common multiple (LCM) and greatest common divisor (GCD), showcasing their interconnectedness and relevance in diverse mathematical problems.
Understanding the Concept of Common Denominator
Before jumping into the specifics of 8 and 3, let's establish a firm grasp of the core concept. A common denominator, in the context of fractions, is a shared multiple of the denominators of two or more fractions. This shared multiple allows us to add, subtract, compare, or otherwise manipulate the fractions effectively. Without a common denominator, performing these operations becomes significantly more complex, if not impossible.
Why do we need a common denominator?
Imagine trying to add 1/8 of a pizza to 1/3 of a pizza. You can't simply add the numerators (1 + 1 = 2) and keep the denominators (2/11), because the slices are different sizes. A common denominator ensures we're comparing and combining equal-sized pieces. Only then can we accurately represent the combined portion of the pizza.
Methods for Finding the Common Denominator of 8 and 3
Several methods exist for finding the common denominator, each with its advantages and disadvantages. Let's explore the most common ones:
1. Listing Multiples:
This straightforward method involves listing the multiples of each denominator until we find a common multiple.
- Multiples of 8: 8, 16, 24, 32, 40, 48, 56, 64, 72, 80, 88, 96, 104, 112, 120...
- Multiples of 3: 3, 6, 9, 12, 15, 18, 21, 24, 27, 30, 33, 36, 39, 42, 45, 48,...
Notice that 24 and 48 appear in both lists. Therefore, both 24 and 48 are common denominators of 8 and 3.
2. Prime Factorization Method:
This more sophisticated method utilizes prime factorization to find the least common multiple (LCM), which is the smallest common denominator.
- Prime factorization of 8: 2 x 2 x 2 = 2³
- Prime factorization of 3: 3
To find the LCM, we take the highest power of each prime factor present in the factorizations:
LCM(8, 3) = 2³ x 3 = 8 x 3 = 24
Therefore, the least common denominator (LCD) of 8 and 3 is 24. This method guarantees finding the smallest common denominator, simplifying further calculations.
3. Using the Formula: LCM(a, b) = (a x b) / GCD(a, b)
This method leverages the relationship between the least common multiple (LCM) and the greatest common divisor (GCD). First, we need to find the GCD of 8 and 3.
The GCD of two numbers is the largest number that divides both without leaving a remainder. Since 8 and 3 have no common divisors other than 1, their GCD is 1.
Now, we can use the formula:
LCM(8, 3) = (8 x 3) / GCD(8, 3) = (24) / 1 = 24
This confirms that the least common denominator is 24.
Practical Applications: Why Finding the Common Denominator Matters
The ability to find a common denominator is fundamental to numerous mathematical operations and applications. Here are some key examples:
1. Adding and Subtracting Fractions:
As mentioned earlier, adding or subtracting fractions requires a common denominator. Let's add 1/8 and 1/3:
- Find the common denominator: We've established that the least common denominator is 24.
- Convert fractions:
- 1/8 = (1 x 3) / (8 x 3) = 3/24
- 1/3 = (1 x 8) / (3 x 8) = 8/24
- Add the fractions: 3/24 + 8/24 = 11/24
Therefore, 1/8 + 1/3 = 11/24.
2. Comparing Fractions:
Determining which of two fractions is larger necessitates a common denominator. Let's compare 1/8 and 1/3:
- Convert fractions to a common denominator (24):
- 1/8 = 3/24
- 1/3 = 8/24
- Compare: 8/24 > 3/24, so 1/3 > 1/8.
3. Solving Equations with Fractions:
Many algebraic equations involve fractions. Finding a common denominator is essential for simplifying and solving these equations. For instance, consider the equation: x/8 + x/3 = 11/24. The first step towards solving this would be to find a common denominator for the fractions with 'x', which as we know is 24.
4. Real-world applications:
The concept extends far beyond theoretical mathematics. Consider scenarios involving measurements, ratios, or proportions. For example, in carpentry, combining different sized pieces of wood might require calculating with fractions, demanding a common denominator for accurate calculations. Similar situations arise in cooking, construction, or any field requiring precise measurements.
Extending the Concept: LCM and GCD
The quest for the common denominator intrinsically links to the concepts of LCM and GCD. Let's clarify these connections:
-
Least Common Multiple (LCM): The smallest positive number that is a multiple of two or more numbers. In our case, the LCM of 8 and 3 is 24, representing the smallest common denominator.
-
Greatest Common Divisor (GCD): The largest positive integer that divides each of the integers without leaving a remainder. The GCD of 8 and 3 is 1, indicating that they share no common factors greater than 1.
The relationship between LCM and GCD is expressed by the formula we used earlier: LCM(a, b) = (a x b) / GCD(a, b). This formula provides a powerful alternative method for determining the LCM, directly applicable to finding the least common denominator.
Conclusion: Mastering the Common Denominator
Understanding how to find the common denominator, particularly the least common denominator, is a cornerstone of arithmetic and algebra. It's not merely a procedural exercise; it's a key skill with far-reaching implications in various mathematical operations and practical applications. By mastering different methods—from listing multiples to employing prime factorization and leveraging the LCM/GCD relationship—you equip yourself with the tools to tackle more complex problems involving fractions and related mathematical concepts with confidence and efficiency. The seemingly simple task of finding the common denominator of 8 and 3 unveils a wealth of underlying mathematical principles essential for success in numerous academic and real-world endeavors.
Latest Posts
Latest Posts
-
What Is This Phenomenon Known As
Apr 01, 2025
-
What Is The Ultimate Source Of Energy In Ecosystem
Apr 01, 2025
-
What Shape Has 6 Faces 8 Vertices And 12 Edges
Apr 01, 2025
-
5 Letter Words Start With Vi
Apr 01, 2025
-
Which Of The Following Is An Exothermic Reaction
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about Common Denominator Of 8 And 3 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
