829 Rounded To The Nearest Ten
Juapaving
Mar 27, 2025 · 5 min read
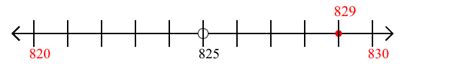
Table of Contents
829 Rounded to the Nearest Ten: A Deep Dive into Rounding Techniques
Rounding numbers is a fundamental mathematical concept with wide-ranging applications in everyday life, from estimating grocery bills to calculating project timelines. This article will explore the process of rounding 829 to the nearest ten, examining the underlying principles and expanding on various rounding methods. We'll also delve into the significance of rounding in different contexts, addressing common misconceptions and offering practical examples.
Understanding the Concept of Rounding
Rounding involves approximating a number to a specified level of precision. This involves simplifying a number by reducing the number of significant digits. The goal is to make the number easier to work with while minimizing the loss of accuracy. The most common rounding techniques involve rounding to the nearest ten, hundred, thousand, and so on.
The process generally focuses on the digit to the right of the place value you're rounding to. If this digit is 5 or greater, you round up. If it's less than 5, you round down. Let's apply this to our target number: 829.
Rounding 829 to the Nearest Ten: A Step-by-Step Guide
To round 829 to the nearest ten, we follow these steps:
-
Identify the tens digit: In 829, the tens digit is 2.
-
Look at the digit to the right: The digit to the right of the tens digit is 9.
-
Apply the rounding rule: Since 9 is greater than or equal to 5, we round the tens digit up.
-
Perform the rounding: Rounding up the tens digit (2) by one results in 3. The units digit becomes 0.
-
The final result: Therefore, 829 rounded to the nearest ten is 830.
Visualizing the Rounding Process
Imagine a number line:
... 820 821 822 823 824 825 826 827 828 829 830 831 ...
829 is closer to 830 than to 820. The midpoint between 820 and 830 is 825. Since 829 is above this midpoint, it rounds up to 830. This visualization helps to solidify the understanding of the rounding process.
Different Rounding Methods: A Comparative Analysis
While the method described above is the most common, other rounding methods exist. Understanding these nuances is crucial for precision in specific contexts.
1. Rounding Down (Truncation):
This method simply discards the digits to the right of the rounding place. In our case, rounding 829 down to the nearest ten would result in 820. This method is simpler but can lead to significant inaccuracies, particularly when dealing with numerous calculations.
2. Rounding Up:
This method always rounds the digit up, regardless of whether the digit to the right is less than 5. Applying this to 829 would still result in 830, but this method consistently overestimates the value.
3. Rounding to the Nearest Even (Banker's Rounding):
This method is designed to mitigate bias and improve accuracy in large datasets. If the digit to the right is exactly 5, the digit being rounded is rounded to the nearest even number. This method is commonly used in financial applications and statistical analysis. If we were rounding 825 to the nearest ten using this method, it would round to 820, as opposed to 830 (which standard rounding would yield).
The Importance of Rounding in Real-World Applications
Rounding numbers is not merely an academic exercise; it has numerous practical applications across various fields:
-
Finance: Rounding is essential for calculating taxes, interest rates, and financial statements. Banker's rounding is frequently employed to ensure fairness and accuracy.
-
Engineering: Rounding is used in engineering designs and calculations to simplify measurements and ensure compatibility between components.
-
Statistics: Rounding is used to present data in a concise and easily understandable manner. Rounding to a certain significant figure allows better data visualization and interpretation.
-
Everyday Life: We implicitly use rounding when estimating costs, distances, or quantities. For example, rounding a grocery bill to the nearest dollar simplifies budgeting.
-
Computer Science: Rounding is crucial in computer programming for managing floating-point numbers and ensuring numerical stability in computations.
Common Misconceptions about Rounding
Several common misconceptions surround rounding:
-
Chained Rounding: Avoid chaining rounding operations. For example, rounding 829 to the nearest hundred first (800) and then to the nearest ten (800) is not equivalent to directly rounding 829 to the nearest ten (830).
-
Misinterpretation of Rounding Rules: It's crucial to understand the specific rounding rule being applied (e.g., rounding to the nearest even). Inconsistencies can lead to significant errors, particularly in large-scale computations.
-
Ignoring Significant Figures: The number of significant figures retained after rounding depends on the context and the desired level of accuracy. Improper handling of significant figures can affect the accuracy and reliability of calculations.
Expanding on the Concept: Rounding to Different Place Values
Understanding rounding to the nearest ten is crucial but it's equally vital to understand its application to other place values:
-
Rounding 829 to the nearest hundred: The hundreds digit is 8. The tens digit (2) is less than 5, so we round down to 800.
-
Rounding 829 to the nearest thousand: The thousands digit is 0 (implicitly). The hundreds digit (8) is greater than or equal to 5, so we round up to 1000.
Conclusion: Mastering Rounding for Enhanced Accuracy and Efficiency
Rounding is a fundamental mathematical operation with broad practical applications. While seemingly simple, understanding its different methods and potential pitfalls is crucial for accurate and efficient calculations in various fields. By mastering rounding techniques, you can enhance your numerical skills and improve your ability to interpret and utilize numerical data effectively. Remember to always consider the context and choose the appropriate rounding method to ensure accurate and meaningful results. Whether estimating a shopping bill or conducting complex engineering calculations, a firm grasp of rounding principles will greatly improve your analytical abilities and problem-solving skills. Furthermore, appreciating the nuances of different rounding methods allows for a deeper understanding of the potential impact of rounding on overall accuracy.
Latest Posts
Latest Posts
-
Who First Used The Term Cell
Mar 30, 2025
-
Light Energy Is Converted To Chemical Energy In The
Mar 30, 2025
-
How Do You Add Scientific Notation
Mar 30, 2025
-
Solving Systems Of Equations By Elimination Solver
Mar 30, 2025
-
What Is The Valence Value Of Carbon
Mar 30, 2025
Related Post
Thank you for visiting our website which covers about 829 Rounded To The Nearest Ten . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
