60 Is What Percent Of 400
Juapaving
May 13, 2025 · 4 min read
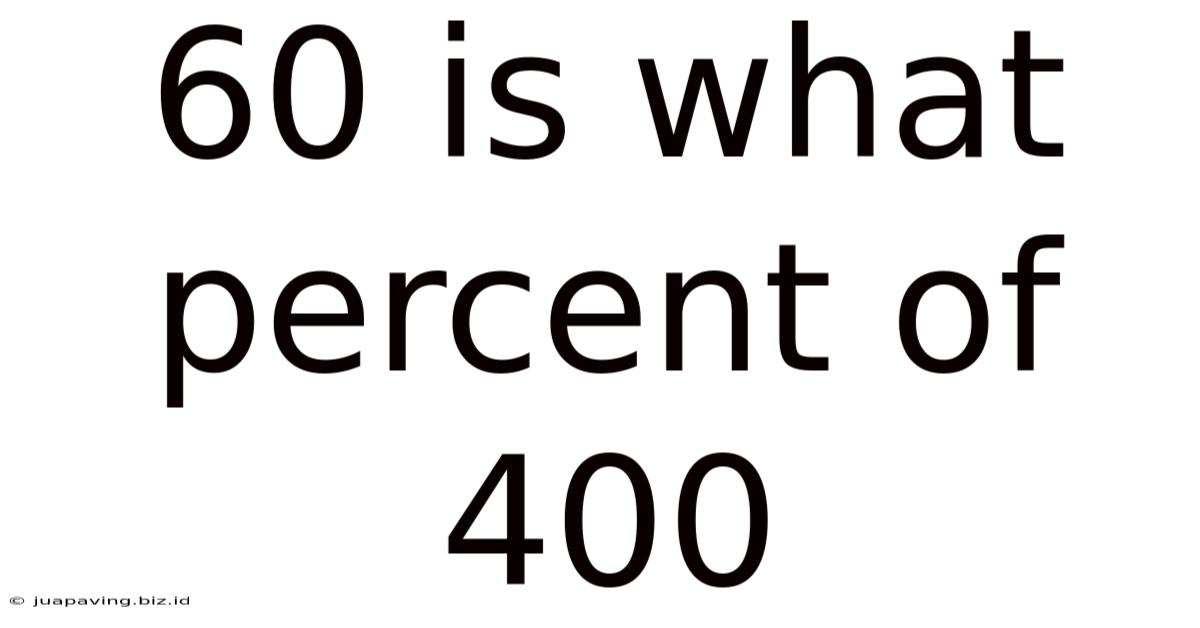
Table of Contents
60 is What Percent of 400? A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill in many areas of life, from calculating discounts and taxes to analyzing data and understanding statistics. This article will delve into how to determine what percent 60 is of 400, providing a step-by-step explanation, exploring different methods, and offering practical applications to solidify your understanding of percentage calculations.
Understanding Percentages
Before we tackle the specific problem of determining what percent 60 is of 400, let's refresh our understanding of percentages. A percentage is a fraction or ratio expressed as a number out of 100. The symbol "%" represents "per cent," meaning "out of one hundred." For instance, 50% means 50 out of 100, which is equivalent to 1/2 or 0.5.
Method 1: Using the Percentage Formula
The most common method for calculating percentages involves using a simple formula:
(Part / Whole) * 100% = Percentage
In our case:
- Part: 60
- Whole: 400
Let's plug these values into the formula:
(60 / 400) * 100% = 15%
Therefore, 60 is 15% of 400.
Method 2: Simplifying the Fraction
Another approach involves simplifying the fraction before converting it to a percentage. This method can be particularly helpful when dealing with larger numbers or fractions that can be easily simplified:
- Form the fraction: 60/400
- Simplify the fraction: Both 60 and 400 are divisible by 20. Dividing both the numerator and the denominator by 20, we get 3/20.
- Convert the fraction to a decimal: Divide the numerator (3) by the denominator (20): 3 ÷ 20 = 0.15
- Convert the decimal to a percentage: Multiply the decimal by 100%: 0.15 * 100% = 15%
This method confirms that 60 is 15% of 400.
Method 3: Using Proportions
Proportions offer another effective way to solve percentage problems. A proportion sets up an equivalence between two ratios. We can set up a proportion like this:
60/400 = x/100
Where 'x' represents the percentage we're trying to find. To solve for 'x', we can cross-multiply:
60 * 100 = 400 * x 6000 = 400x x = 6000 / 400 x = 15
Again, this confirms that 60 is 15% of 400.
Practical Applications of Percentage Calculations
Understanding percentage calculations is crucial in numerous real-world scenarios. Here are a few examples:
1. Sales and Discounts:
Imagine a store offering a 25% discount on an item originally priced at $400. Using our percentage formula, we can calculate the discount amount:
(25/100) * $400 = $100
The discount is $100, making the final price $300.
2. Tax Calculations:
Suppose a sales tax rate is 6%. To calculate the tax on a $400 purchase, we'd use the formula:
(6/100) * $400 = $24
The sales tax would be $24, bringing the total cost to $424.
3. Grade Calculation:
If you scored 60 points out of a possible 400 points on an exam, your percentage score would be:
(60/400) * 100% = 15%
This demonstrates your performance on the exam.
4. Data Analysis:
In data analysis, percentages are frequently used to represent proportions within datasets. For example, if 60 out of 400 respondents in a survey answered "yes" to a particular question, the percentage of "yes" responses would be 15%. This allows for easier comparison and interpretation of data.
5. Financial Calculations:
Percentages are fundamental in finance. Calculating interest rates, returns on investment (ROI), and understanding financial statements all heavily rely on percentage calculations. For instance, if you invest $400 and earn a 15% return, your profit would be $60.
Beyond the Basics: More Complex Percentage Problems
While the problem "60 is what percent of 400?" is relatively straightforward, the principles involved can be applied to more complex percentage problems. For example, you might encounter scenarios where you need to find the whole amount given a part and a percentage, or find the part given the whole and a percentage. Understanding the fundamental percentage formula and the various methods presented here will equip you to tackle these more advanced challenges.
Mastering Percentages: Tips and Practice
To truly master percentage calculations, consistent practice is key. Try working through various percentage problems, starting with simpler examples and gradually progressing to more complex ones. Online resources and workbooks offer ample opportunities for practice. Remember to:
- Understand the formula: Familiarize yourself with the formula (Part / Whole) * 100% = Percentage and how to manipulate it to solve for different unknowns.
- Practice different methods: Experiment with using different methods (simplifying fractions, proportions) to solve the same problems. This will help you develop a deeper understanding and find the most efficient approach for various scenarios.
- Check your work: Always verify your answers using a different method or by working backward from your solution.
- Apply your knowledge: Look for opportunities to apply your percentage skills in everyday life, from shopping to analyzing data.
By understanding the fundamental principles of percentage calculations and practicing regularly, you'll confidently handle percentage problems and apply this valuable skill in a wide range of situations. The seemingly simple question, "60 is what percent of 400?" serves as a gateway to a deeper understanding of a crucial mathematical concept with widespread applications.
Latest Posts
Latest Posts
-
3 1 2 As An Improper Fraction
May 13, 2025
-
5 6 Divided By 1 12
May 13, 2025
-
How Many Ml In A 1 75 Liter
May 13, 2025
-
Two Thirds Of A Number Algebraic Expression
May 13, 2025
-
Does A Voltmeter Have A High Resistance
May 13, 2025
Related Post
Thank you for visiting our website which covers about 60 Is What Percent Of 400 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.