Which Graph Represents Y 3 Sqrt X
Juapaving
May 11, 2025 · 5 min read
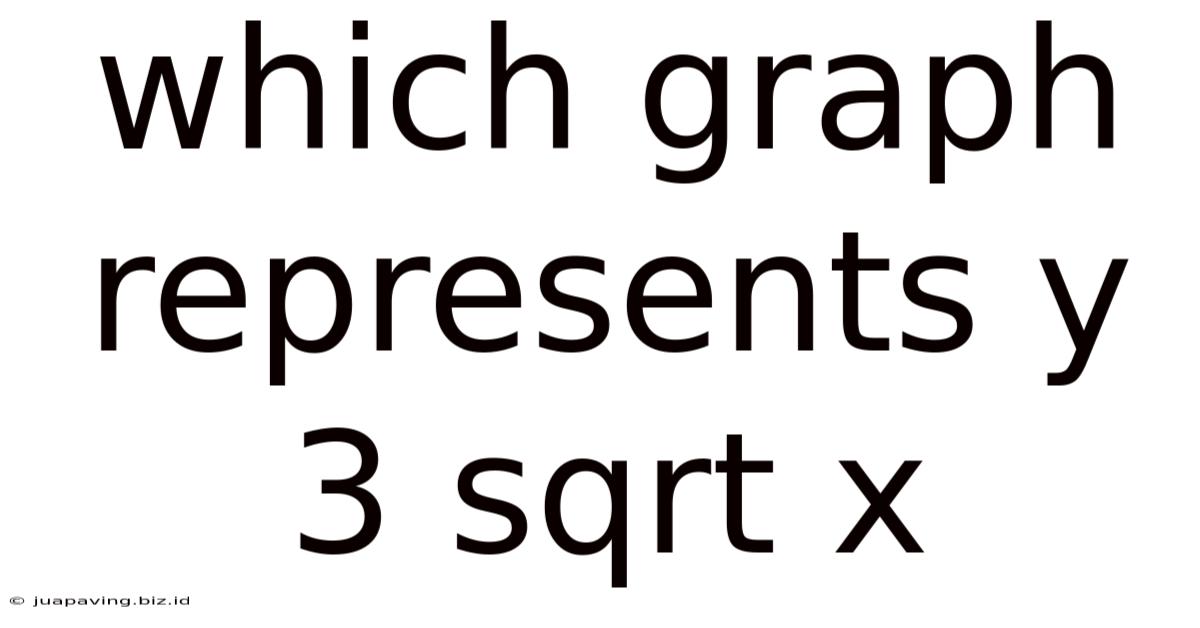
Table of Contents
Which Graph Represents y = 3√x? Understanding Cube Root Functions
The equation y = 3√x represents a cube root function. Understanding its properties and how they translate visually onto a graph is crucial for anyone studying algebra, pre-calculus, or calculus. This article will delve deep into the characteristics of this specific function, exploring its domain, range, behavior, and how to definitively identify its graph amongst other functions.
Understanding the Cube Root Function
Before we pinpoint the graph of y = 3√x, let's establish a firm understanding of cube root functions in general. The cube root of a number is the value that, when multiplied by itself three times, results in the original number. For example, the cube root of 8 is 2 (because 2 x 2 x 2 = 8). Mathematically, we represent the cube root as ∛x or x^(1/3).
The general form of a cube root function is y = a∛(x - h) + k, where:
- a: Determines the vertical stretch or compression and reflection across the x-axis. If |a| > 1, the graph is stretched vertically; if 0 < |a| < 1, it's compressed vertically. If a is negative, the graph is reflected across the x-axis.
- h: Represents the horizontal shift. A positive h shifts the graph to the right, and a negative h shifts it to the left.
- k: Represents the vertical shift. A positive k shifts the graph upward, and a negative k shifts it downward.
In our specific case, y = 3√x, we have a = 1, h = 0, and k = 0. This means the graph will be a basic cube root function without any horizontal or vertical shifts, and only a slight vertical stretch compared to the parent function y = ∛x.
Key Characteristics of y = 3√x
Let's examine the key characteristics that will help us identify the graph:
1. Domain and Range
-
Domain: The domain of a function is the set of all possible input values (x-values). The cube root function is defined for all real numbers. Therefore, the domain of y = 3√x is (-∞, ∞). You can input any real number, positive, negative, or zero, and obtain a real output.
-
Range: The range of a function is the set of all possible output values (y-values). Similar to the domain, the cube root of any real number is also a real number. Therefore, the range of y = 3√x is also (-∞, ∞).
2. Shape and Behavior
Unlike square root functions, which are only defined for non-negative values of x, cube root functions are defined for all real numbers. This leads to a distinct graph shape. The graph of y = 3√x passes through the origin (0,0).
-
As x approaches positive infinity (x → ∞), y also approaches positive infinity (y → ∞). The function increases monotonically (always increasing) as x increases.
-
As x approaches negative infinity (x → -∞), y approaches negative infinity (y → -∞). The function continues to increase as x becomes more negative.
-
The graph is smooth and continuous. There are no breaks, jumps, or asymptotes in the graph.
-
The graph has a point of inflection at (0, 0). This means the curvature of the graph changes at this point; it's neither concave up nor concave down at this point.
3. Comparison to y = ∛x
It's important to compare y = 3√x to its parent function, y = ∛x. The only difference is the coefficient "3". This factor stretches the graph vertically by a factor of 3. Every y-value on y = ∛x will be multiplied by 3 to obtain the corresponding y-value on y = 3√x. The overall shape remains the same; it's just taller and narrower.
Identifying the Graph: A Step-by-Step Approach
Given a selection of graphs, here's how to confidently select the one representing y = 3√x:
-
Check for the Origin: The graph must pass through the origin (0, 0).
-
Observe the Domain and Range: The graph should extend infinitely in both the positive and negative x and y directions.
-
Look for Monotonic Increase: The graph should continuously increase as x increases, without any decreases or plateaus.
-
Identify the Vertical Stretch: Compared to the graph of y = ∛x, the graph of y = 3√x will appear taller and more vertically stretched. Points on y = ∛x will be closer to the x-axis than corresponding points on y = 3√x.
Distinguishing y = 3√x from Other Functions
It’s crucial to differentiate y = 3√x from other similar-looking functions. For instance:
-
y = x³: This is a cubic function, not a cube root function. It will have a very different shape, with y increasing much more rapidly as x increases.
-
y = √x: This is a square root function, only defined for x ≥ 0. Its graph starts at (0, 0) and increases monotonically for x > 0, but it won't extend to the negative x-values.
-
y = 3x: This is a linear function with a slope of 3. It passes through the origin but increases linearly at a constant rate, unlike the increasingly slower growth of the cube root function.
-
y = 3/√x: This involves a reciprocal square root function, which has a vertical asymptote at x = 0 and approaches 0 as x increases.
By carefully analyzing these differences and applying the characteristics discussed above, you can accurately differentiate y = 3√x from these other functions.
Practical Applications and Further Exploration
Cube root functions, like y = 3√x, appear in various fields:
-
Physics: Calculating the volume of a cube from its side length.
-
Engineering: Modeling certain physical phenomena with non-linear relationships.
-
Statistics: Used in some statistical distributions.
-
Mathematics: Foundation for more complex mathematical concepts.
Further exploration could include analyzing the derivative and integral of y = 3√x, examining its concavity, and exploring transformations involving horizontal and vertical shifts and reflections.
Conclusion
Identifying the graph of y = 3√x requires a comprehensive understanding of cube root functions and their properties. By focusing on the domain, range, shape, behavior, and comparing it with related functions, one can confidently identify its unique graphical representation. Remember, understanding the basic characteristics of functions is paramount for success in mathematics and related disciplines. This knowledge will be an invaluable asset as you progress through your studies.
Latest Posts
Latest Posts
-
Collision Theory And Rate Of Reaction
May 13, 2025
-
What Is Group Of Whales Called
May 13, 2025
-
Differentiate Between Capital Expenditure And Revenue Expenditure
May 13, 2025
-
Which Of The Following Is Correct Answer
May 13, 2025
-
6 726 Rounded To The Nearest Hundredth
May 13, 2025
Related Post
Thank you for visiting our website which covers about Which Graph Represents Y 3 Sqrt X . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.