Which Expression Is Equivalent To Sqrt 200
Juapaving
Mar 28, 2025 · 5 min read
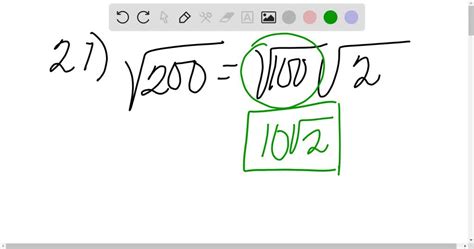
Table of Contents
Which Expression is Equivalent to √200? A Deep Dive into Square Roots and Simplification
Finding an equivalent expression for √200 might seem like a simple algebra problem, but it opens a door to understanding fundamental concepts in mathematics, particularly concerning square roots, prime factorization, and simplification. This comprehensive guide will not only reveal the equivalent expression but also equip you with the skills to tackle similar problems with confidence. We'll delve into the process step-by-step, exploring various methods and highlighting important mathematical principles along the way.
Understanding Square Roots
Before we tackle √200, let's establish a solid understanding of square roots. A square root of a number is a value that, when multiplied by itself, equals the original number. For example, the square root of 9 (√9) is 3 because 3 x 3 = 9. Conversely, the square root of 25 (√25) is 5 because 5 x 5 = 25. However, many numbers don't have perfect square roots – meaning their square roots are not whole numbers. This is where simplification becomes crucial.
Perfect Squares: The Building Blocks of Simplification
Perfect squares are numbers that are the result of squaring a whole number. Some examples include:
- 1 (1 x 1)
- 4 (2 x 2)
- 9 (3 x 3)
- 16 (4 x 4)
- 25 (5 x 5)
- 36 (6 x 6)
- and so on...
Identifying perfect squares within a number is key to simplifying its square root. This process often involves prime factorization, which we'll explore in detail.
Prime Factorization: The Key to Simplifying √200
Prime factorization is the process of breaking down a number into its prime factors. Prime numbers are whole numbers greater than 1 that are only divisible by 1 and themselves (e.g., 2, 3, 5, 7, 11, etc.). Let's apply this to 200:
- Start with the smallest prime number, 2: 200 is divisible by 2, resulting in 100.
- Continue dividing by 2: 100 is divisible by 2, resulting in 50. 50 is also divisible by 2, resulting in 25.
- Move to the next prime number, 5: 25 is divisible by 5, resulting in 5. 5 is a prime number itself.
Therefore, the prime factorization of 200 is 2 x 2 x 2 x 5 x 5, or 2³ x 5².
Simplifying √200 using Prime Factorization
Now that we have the prime factorization of 200 (2³ x 5²), we can simplify its square root:
√200 = √(2³ x 5²) = √(2² x 2 x 5²)
Remember that √(a x a) = a. We can rewrite the expression as:
√(2² x 5²) x √2 = (√2² x √5²) x √2 = (2 x 5) x √2 = 10√2
Therefore, the simplified equivalent expression for √200 is 10√2.
Alternative Methods for Simplification
While prime factorization is the most systematic approach, other methods can also be used to simplify square roots, particularly for smaller numbers. These methods rely on recognizing perfect squares as factors:
-
Recognizing perfect square factors: You might notice that 200 is divisible by 100 (a perfect square). So, √200 = √(100 x 2) = √100 x √2 = 10√2. This method requires a keen eye for perfect square factors.
-
Repeated division by perfect squares: You can repeatedly divide the number under the square root by perfect squares until you reach a number with no perfect square factors left. For example:
√200 = √(4 x 50) = 2√50 = 2√(25 x 2) = 2 x 5√2 = 10√2
Practical Applications and Further Exploration
The ability to simplify square roots has far-reaching applications beyond basic algebra. It's essential in:
- Geometry: Calculating the lengths of sides and diagonals in geometric shapes.
- Trigonometry: Solving problems involving trigonometric functions and angles.
- Calculus: Simplifying expressions and solving equations.
- Physics and Engineering: Many formulas and equations involve square roots.
Beyond √200: Mastering Square Root Simplification
The process we used for simplifying √200 can be applied to any square root. The key steps are:
- Find the prime factorization of the number under the square root.
- Identify pairs of identical prime factors. Each pair can be taken out from under the square root sign.
- Any prime factors without a pair remain under the square root sign.
For example, let's simplify √72:
- Prime factorization of 72: 2³ x 3²
- Identifying pairs: (2²) and (3²)
- Simplification: √(2² x 3² x 2) = 2 x 3√2 = 6√2
Troubleshooting Common Mistakes
When simplifying square roots, several common mistakes can occur:
- Incorrect prime factorization: Ensure you correctly break down the number into its prime factors. A single error can lead to an incorrect simplified expression.
- Forgetting remaining factors: Make sure to include any prime factors that don't form a pair under the square root sign.
- Arithmetic errors: Carefully perform the calculations involved in combining the factors.
By meticulously following the prime factorization method and double-checking your work, you can avoid these mistakes.
Conclusion: Equivalence and Understanding
We've definitively shown that 10√2 is an equivalent expression to √200. More importantly, we've explored the underlying mathematical principles – square roots, prime factorization, and simplification – that allow us to confidently tackle these types of problems. Mastering these concepts is crucial for success in various mathematical fields and for developing a strong understanding of numerical relationships. The ability to simplify square roots is a fundamental skill, and with practice, you can become proficient in this important area of mathematics. Remember that consistent practice and a deep understanding of the underlying concepts are key to mastering square root simplification.
Latest Posts
Latest Posts
-
How Many Feet In 95 Inches
Mar 31, 2025
-
350 Square Meters In Square Feet
Mar 31, 2025
-
Explain How Software Is Distinct From Hardware
Mar 31, 2025
-
Greater Than Less Than Decimals Calculator
Mar 31, 2025
-
Father Of The Constitution Of India
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about Which Expression Is Equivalent To Sqrt 200 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
