What's The Square Root Of 576
Juapaving
Apr 04, 2025 · 5 min read
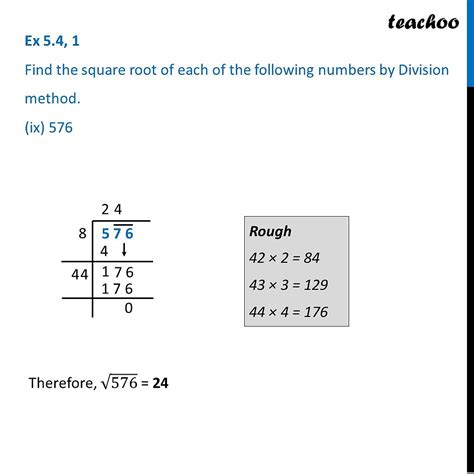
Table of Contents
What's the Square Root of 576? A Deep Dive into Square Roots and Number Theory
Finding the square root of a number might seem like a simple mathematical operation, but it opens a door to a fascinating world of number theory and mathematical concepts. This article will not only answer the question "What's the square root of 576?" but will also explore the underlying principles, methods for calculating square roots, and the broader significance of this seemingly straightforward calculation.
Understanding Square Roots
Before we delve into the specifics of the square root of 576, let's establish a solid understanding of what a square root actually is. Simply put, the square root of a number is a value that, when multiplied by itself (squared), gives the original number. For example, the square root of 9 is 3 because 3 * 3 = 9. We denote the square root using the radical symbol: √. So, √9 = 3.
Square roots can be of two types:
-
Perfect Squares: These are numbers that have whole number square roots. For instance, 9, 16, 25, and 36 are perfect squares because their square roots (3, 4, 5, and 6 respectively) are integers.
-
Non-Perfect Squares: These are numbers whose square roots are not whole numbers. For example, the square root of 2 is approximately 1.414, which is an irrational number (a number that cannot be expressed as a simple fraction).
Calculating the Square Root of 576
Now, let's tackle the central question: What is the square root of 576?
There are several ways to calculate this:
1. Prime Factorization Method
This method involves breaking down the number into its prime factors and then finding the square root.
-
Find the prime factorization of 576: 576 = 2 x 2 x 2 x 2 x 2 x 2 x 3 x 3 = 2<sup>6</sup> x 3<sup>2</sup>
-
Pair the factors: Notice that we have pairs of 2s and a pair of 3s.
-
Take one factor from each pair: This gives us 2 x 2 x 3 = 12.
-
Therefore, √576 = 24 because 24 * 24 = 576
2. Estimation and Iteration Method
This method involves making an educated guess and refining it through successive iterations.
-
Make an initial guess: Since 20 * 20 = 400 and 30 * 30 = 900, we know the square root of 576 lies between 20 and 30. Let's start with a guess of 25.
-
Divide the number by the guess: 576 / 25 = 23.04
-
Average the guess and the result: (25 + 23.04) / 2 = 24.02
-
Repeat steps 2 and 3: Using 24.02 as the new guess, we get a very close approximation to 24.
This method, while less precise for large numbers, demonstrates an iterative approach commonly used in numerical analysis.
3. Using a Calculator
The simplest method, especially for larger numbers, is to use a calculator. Simply input 576 and press the square root button (√). The calculator will quickly provide the answer: 24.
The Significance of Square Roots in Mathematics
The seemingly simple concept of square roots has profound implications across various areas of mathematics:
-
Geometry: Square roots are crucial in calculating distances, areas, and volumes of geometrical figures. For example, the Pythagorean theorem (a² + b² = c²) relies heavily on square roots to determine the length of the hypotenuse in a right-angled triangle.
-
Algebra: Solving quadratic equations frequently involves extracting square roots. Many real-world problems, from projectile motion to determining the optimal dimensions of a container, can be modeled using quadratic equations, making square roots essential tools for their solution.
-
Calculus: Derivatives and integrals, fundamental concepts in calculus, often involve manipulating expressions that include square roots. The study of curves and areas under curves frequently relies on understanding and applying square root operations.
-
Number Theory: Square roots delve into the properties of integers and their relationships. Perfect squares, for example, play a key role in various number theoretical concepts like modular arithmetic and Diophantine equations. The study of perfect squares and their distribution provides insights into the structure of numbers themselves.
-
Computer Science: Square roots are a fundamental part of many algorithms and calculations used in computer graphics, game development, and simulations. They are involved in determining distances, rotations, and other operations that require the manipulation of spatial information.
Beyond the Square Root: Higher-Order Roots
The concept of square roots extends to higher-order roots, such as cube roots (∛), fourth roots (∜), and so on. These represent values that, when multiplied by themselves a certain number of times, yield the original number. For instance, the cube root of 64 (∛64) is 4 because 4 * 4 * 4 = 64. These higher-order roots, just like square roots, appear frequently in advanced mathematical and scientific applications.
Applications of Square Roots in Real Life
Square roots aren't just confined to textbooks and classrooms. They have many practical applications in real-world scenarios:
-
Construction and Engineering: Calculating the length of diagonals, determining the area of land plots, and designing structures all involve using square roots.
-
Physics: Many physics formulas, especially those dealing with motion, forces, and energy, incorporate square roots. Calculating the velocity of an object or the energy of a particle often requires square root calculations.
-
Finance: Compound interest calculations, essential for understanding investment growth, rely on square roots.
-
Statistics: Standard deviation, a critical measure of data dispersion, uses square roots in its calculation. Understanding the spread of data in various fields like economics, biology, and social sciences relies on the application of square roots.
-
Computer Graphics: Generating realistic images and animations in computer games and simulations heavily uses square root calculations to handle 3D coordinates and transformations.
Conclusion
Finding the square root of 576, while seemingly straightforward, reveals a deeper understanding of fundamental mathematical concepts. It's not just about obtaining the answer (24); it's about grasping the underlying principles, exploring different calculation methods, and appreciating the wide-ranging applications of square roots in various fields. This exploration touches upon aspects of number theory, geometry, algebra, calculus, and even computer science. Understanding square roots is more than just a mathematical skill; it's a key to unlocking a deeper appreciation of the world around us, as it underpins many scientific and technological advancements. So, the next time you encounter a square root, remember that it's a gateway to a world of mathematical wonder.
Latest Posts
Latest Posts
-
What Is The Source Of Oxygen Released During Photosynthesis
May 09, 2025
-
How Many Centimeters Is 13 Inches
May 09, 2025
-
Find The Number Of Edges On This Solid
May 09, 2025
-
Are Hydrogen Bonds Weaker Than Covalent Bonds
May 09, 2025
-
Which Is A Point Mutation And Not A Frameshift Mutation
May 09, 2025
Related Post
Thank you for visiting our website which covers about What's The Square Root Of 576 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.