What Percentage Is 6 Of 8
Juapaving
Mar 20, 2025 · 4 min read
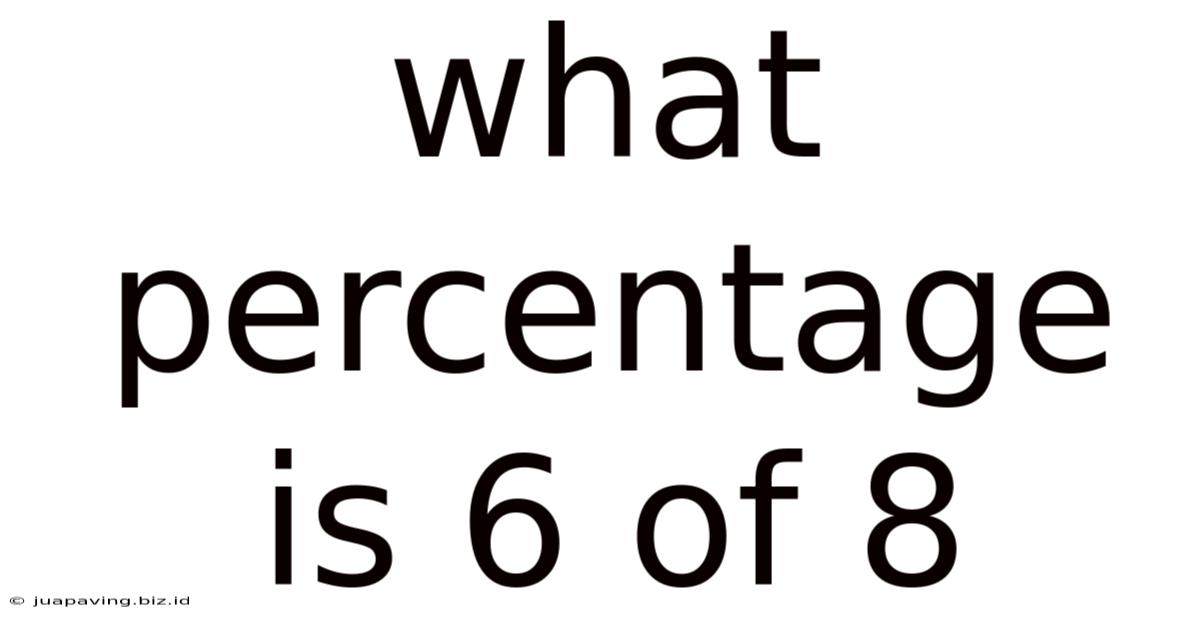
Table of Contents
What Percentage is 6 of 8? A Deep Dive into Percentage Calculations
Understanding percentages is a fundamental skill applicable across numerous areas of life, from calculating discounts and taxes to analyzing data and understanding statistics. This article will explore the seemingly simple question, "What percentage is 6 of 8?", delving far beyond the basic calculation to provide a comprehensive understanding of percentages and their applications.
Calculating the Percentage: The Basic Approach
The most straightforward way to calculate what percentage 6 is of 8 is through a simple formula:
(Part / Whole) x 100% = Percentage
In this case:
(6 / 8) x 100% = 75%
Therefore, 6 is 75% of 8. This is the core answer to the initial question. However, let's delve deeper into the underlying concepts and explore various ways to approach this calculation, enriching your understanding of percentages.
Understanding the Components: Part, Whole, and Percentage
Before moving further, let's define the key elements involved:
- Part: This represents the specific portion of the whole that we are interested in. In our example, the part is 6.
- Whole: This represents the total or complete amount. In our example, the whole is 8.
- Percentage: This expresses the part as a fraction of the whole, expressed as a number out of 100.
Clearly understanding these components is crucial for accurately calculating percentages in any context.
Alternative Calculation Methods
While the primary formula is efficient, several alternative methods can be employed, particularly helpful for mental calculations or situations with less straightforward numbers:
Method 1: Decimal Conversion
Convert the fraction (6/8) to a decimal by performing the division: 6 ÷ 8 = 0.75. Then, multiply the decimal by 100% to obtain the percentage: 0.75 x 100% = 75%.
This method is particularly useful when dealing with calculators or situations where direct percentage calculation isn't immediately possible.
Method 2: Simplification of Fractions
Before performing the division, simplify the fraction 6/8 by finding the greatest common divisor (GCD), which is 2. Simplifying the fraction yields 3/4. This makes the subsequent calculation easier: (3/4) x 100% = 75%.
This method streamlines the calculation process, particularly beneficial when dealing with larger numbers.
Method 3: Using Proportions
This method involves setting up a proportion to solve for the unknown percentage (x):
6/8 = x/100
Cross-multiplying gives:
8x = 600
Solving for x:
x = 600/8 = 75
Therefore, x = 75%, confirming our previous calculations. This approach is valuable for understanding the proportional relationship between the part and the whole.
Practical Applications of Percentage Calculations
The ability to calculate percentages is vital in numerous real-world scenarios:
1. Financial Calculations:
- Discounts: Determining the final price after a percentage discount. For example, a 25% discount on an $80 item.
- Taxes: Calculating the amount of sales tax or income tax owed.
- Interest Rates: Understanding interest accrued on savings accounts or loans.
- Investment Returns: Calculating the percentage return on investments.
- Profit Margins: Determining the percentage of profit made on sales.
2. Data Analysis and Statistics:
- Market Research: Analyzing survey results and expressing them as percentages.
- Scientific Studies: Representing experimental results and statistical significances as percentages.
- Data Visualization: Creating charts and graphs that visually represent data as percentages (pie charts, bar graphs).
3. Everyday Life:
- Tip Calculation: Determining the appropriate tip amount in restaurants.
- Recipe Scaling: Adjusting ingredient quantities in recipes based on percentage changes.
- Grading Systems: Understanding academic grades expressed as percentages.
Beyond the Basics: Advanced Percentage Concepts
While calculating "What percentage is 6 of 8?" provides a foundational understanding, further exploration into more complex percentage scenarios is beneficial:
1. Percentage Increase and Decrease:
These calculations involve determining the percentage change between two values. For instance, if a value increases from 8 to 10, the percentage increase is calculated as:
((New Value - Old Value) / Old Value) x 100% = ((10 - 8) / 8) x 100% = 25%
Similarly, a percentage decrease is calculated using the same formula.
2. Percentage Points:
It's important to differentiate between percentage change and percentage points. A percentage point represents the absolute difference between two percentages. For example, if the interest rate increases from 5% to 8%, the increase is 3 percentage points, not 60%.
3. Compound Interest:
Compound interest involves earning interest on both the principal amount and accumulated interest. Understanding compound interest is crucial for long-term financial planning.
Error Analysis and Avoiding Common Mistakes
Even simple percentage calculations can be prone to errors. Here are some common mistakes to avoid:
- Incorrect Formula Application: Using the wrong formula or misinterpreting the components (part and whole).
- Decimal Errors: Errors in decimal conversions and calculations.
- Unit Inconsistencies: Mixing percentages with other units without proper conversion.
- Misunderstanding Percentage Points: Confusing percentage change with percentage points.
Conclusion: Mastering Percentages for Real-World Success
Mastering percentage calculations isn't just about solving mathematical problems; it's about developing a crucial skillset applicable across various aspects of life. From navigating financial decisions to interpreting data, understanding percentages empowers you to make informed choices and thrive in a data-driven world. The seemingly simple question, "What percentage is 6 of 8?", serves as a springboard to explore a world of numerical applications and problem-solving skills. By understanding the underlying concepts, utilizing various calculation methods, and avoiding common pitfalls, you can confidently tackle any percentage-related challenge that comes your way. Remember to practice regularly to solidify your understanding and improve your computational fluency.
Latest Posts
Latest Posts
-
Are There More Metals Or Nonmetals On The Periodic Table
May 09, 2025
-
Lines Ab And Cd Are Parallel
May 09, 2025
-
Which Of The Following Is An Anterior Pituitary Hormone
May 09, 2025
-
Which Cell Organelle Is The Site For Photosynthesis
May 09, 2025
-
Use The Given Graph Determine The Period Of The Function
May 09, 2025
Related Post
Thank you for visiting our website which covers about What Percentage Is 6 Of 8 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.