What Percentage Is 100 Of 500
Juapaving
May 12, 2025 · 4 min read
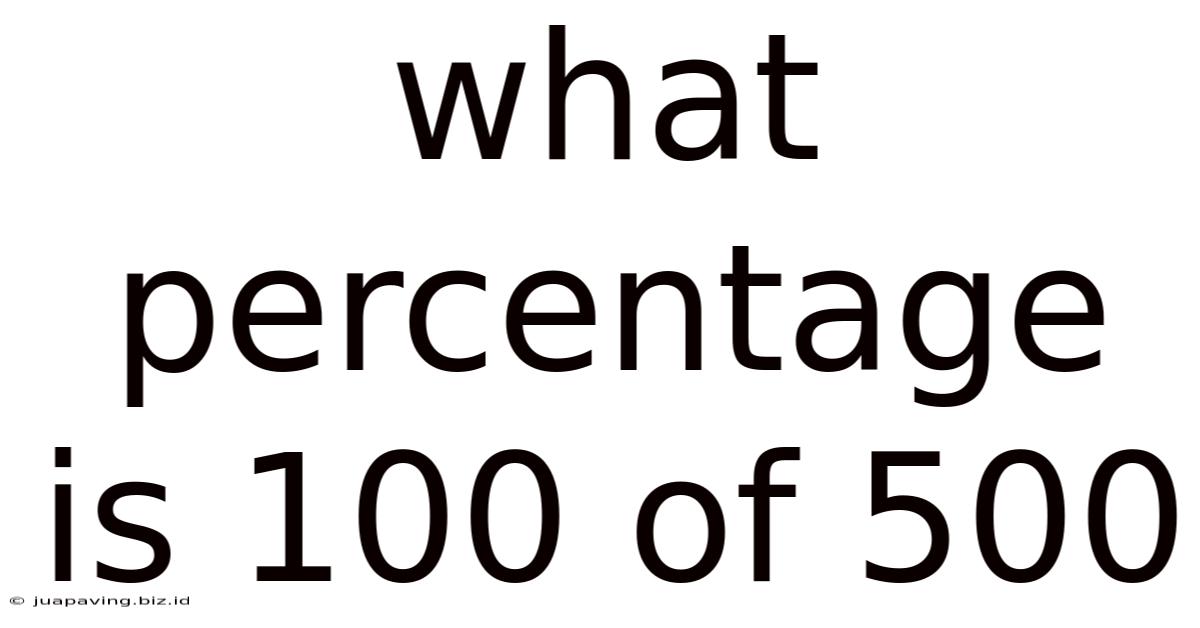
Table of Contents
What Percentage is 100 of 500? A Deep Dive into Percentage Calculations
Understanding percentages is a fundamental skill in various aspects of life, from calculating discounts and taxes to analyzing data and understanding financial reports. This article will thoroughly explore the question, "What percentage is 100 of 500?", providing not just the answer but a comprehensive understanding of the underlying principles and practical applications. We will cover various methods for calculating percentages, explore related concepts, and delve into real-world examples to solidify your understanding.
Calculating the Percentage: The Basic Method
The most straightforward way to determine what percentage 100 represents of 500 is using a simple formula:
(Part / Whole) x 100% = Percentage
In this case:
- Part: 100
- Whole: 500
Substituting these values into the formula:
(100 / 500) x 100% = 20%
Therefore, 100 is 20% of 500.
Understanding the Formula: A Step-by-Step Breakdown
Let's break down the formula to understand its logic:
-
Part / Whole: This fraction represents the proportion of the part (100) to the whole (500). It essentially asks, "What fraction of 500 is 100?" The result is 0.2.
-
x 100%: Multiplying by 100% converts the decimal (0.2) into a percentage. Multiplying by 100 moves the decimal point two places to the right.
This method is versatile and applicable to numerous percentage calculations.
Alternative Methods for Calculating Percentages
While the above method is the most common and generally preferred, alternative methods can be used, particularly when dealing with more complex scenarios or when using different tools.
Method 2: Using Proportions
Proportions offer a visual and intuitive approach. We can set up a proportion:
100/500 = x/100
Where 'x' represents the percentage we want to find. Solving for 'x':
500x = 10000 x = 20
Therefore, x = 20%.
Method 3: Using a Calculator
Most calculators have a percentage function. Simply enter "100 ÷ 500 x 100 =" to obtain the answer directly. This method is efficient for quick calculations.
Practical Applications: Real-World Examples
Understanding percentage calculations is crucial in various everyday situations:
-
Discounts: If a $500 item is discounted by $100, the discount is 20%. This helps consumers easily compare deals.
-
Taxes: If a $500 purchase incurs $100 in taxes, the tax rate is 20%.
-
Grade Calculations: If a student scores 100 points out of a possible 500 points on an exam, their score is 20%.
-
Financial Analysis: Understanding percentage changes in stock prices, profits, or expenses is vital for investment decisions.
-
Data Analysis: Percentages are used extensively to represent proportions and trends in data sets, making information easier to interpret.
-
Surveys and Polls: Results are often presented as percentages to show the proportion of respondents who chose a particular option.
Expanding the Concept: Beyond the Basics
While we've focused on a specific example, the principles extend to any percentage calculation. Let's consider some variations:
-
Finding the Part: If we know that 20% of a number is 100, we can find the whole number using the formula: (Part / Percentage) x 100 = Whole. This gives us (100 / 20) x 100 = 500.
-
Finding the Percentage Increase or Decrease: If a value increases from 500 to 600, the percentage increase is calculated as [(600-500)/500] x 100% = 20%. Similarly, a decrease can be calculated.
-
Compounding Percentages: This involves calculating percentages on percentages, often encountered in interest calculations. For example, calculating compound interest over multiple years involves applying percentage increases iteratively.
Advanced Applications and Related Concepts
-
Percentage Points: It's important to distinguish between percentage change and percentage points. A change from 20% to 30% is a 10 percentage point increase, but a 50% increase in the percentage itself.
-
Weighted Averages: In situations where different values hold varying weights, weighted averages are used. Understanding percentages is crucial for calculating and interpreting weighted averages.
-
Statistical Analysis: Percentages are fundamental in statistical analysis for expressing probabilities, frequencies, and confidence intervals.
Conclusion: Mastering Percentage Calculations
The question, "What percentage is 100 of 500?" provides a springboard for understanding a broader range of percentage calculations and their applications. Mastering these calculations is essential for navigating daily life, making informed decisions, and succeeding in academic and professional settings. By understanding the underlying formulas, applying different calculation methods, and exploring real-world examples, you can develop a solid foundation in this critical mathematical skill. Remember to practice regularly and explore various scenarios to solidify your understanding and build confidence in your ability to calculate and interpret percentages effectively. The more you work with percentages, the more intuitive they become, enabling you to easily tackle increasingly complex problems in various contexts. This foundational understanding opens doors to more advanced mathematical and analytical skills, offering significant advantages in numerous areas of life.
Latest Posts
Latest Posts
-
How To Convert Kwh To Joules
May 13, 2025
-
A Galvanometer Has A Resistance Of 20 Ohm
May 13, 2025
-
Differentiate Between Biotic And Abiotic Factors
May 13, 2025
-
What Percentage Is 1 Out Of 6
May 13, 2025
-
Consider The Circuit Shown In Figure
May 13, 2025
Related Post
Thank you for visiting our website which covers about What Percentage Is 100 Of 500 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.