What Percent Is 10 Of 50
Juapaving
May 14, 2025 · 4 min read
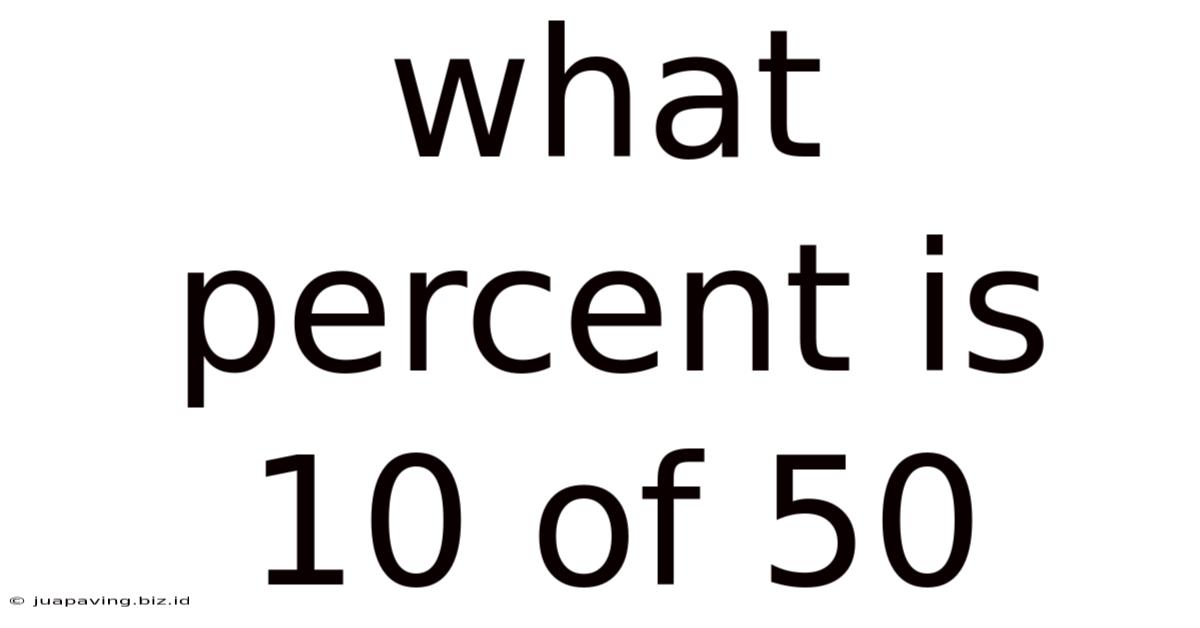
Table of Contents
What Percent is 10 of 50? A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill in many areas of life, from calculating discounts and tips to analyzing data and understanding financial reports. This article will delve into the question, "What percent is 10 of 50?", providing a step-by-step explanation, exploring different methods of calculation, and offering practical applications to solidify your understanding.
Calculating the Percentage: The Basic Method
The most straightforward method to determine what percent 10 is of 50 involves a simple formula:
(Part / Whole) * 100% = Percentage
In this case:
- Part: 10 (the value we want to express as a percentage)
- Whole: 50 (the total value)
Substituting these values into the formula:
(10 / 50) * 100% = 20%
Therefore, 10 is 20% of 50.
Understanding the Formula: A Deeper Dive
Let's break down the formula to understand its underlying logic:
-
Part / Whole: This fraction represents the proportion of the part to the whole. In our example, 10/50 signifies that 10 represents a certain portion of the total 50.
-
*** 100%:** Multiplying by 100% converts the proportion (which is a decimal or fraction) into a percentage. Percentages are simply fractions expressed out of 100.
This formula works for any percentage calculation, regardless of the numbers involved. You can adapt it to find the percentage of any part relative to its whole.
Alternative Methods for Percentage Calculation
While the primary formula is efficient, other methods can be used, particularly when dealing with more complex scenarios or when mental calculations are preferred:
Method 2: Using Proportions
This method involves setting up a proportion:
- x/100 = 10/50
Here, 'x' represents the percentage we're trying to find. Solving for 'x' involves cross-multiplication:
- 50x = 1000
- x = 1000 / 50
- x = 20
Therefore, x = 20%, confirming our previous result. This method is particularly useful for visualizing the relationship between the parts and the whole.
Method 3: Decimal Conversion
This method involves converting the fraction into a decimal first and then multiplying by 100%:
- 10/50 = 0.2
- 0.2 * 100% = 20%
This approach is especially helpful when using calculators, as it streamlines the calculation process.
Practical Applications of Percentage Calculations
Understanding percentage calculations is essential in numerous real-world applications. Here are some examples:
1. Retail Discounts:
Imagine a shirt originally priced at $50 is on sale for $10 off. Using the percentage formula, we determine that the discount is 20% ((10/50) * 100%). This information helps shoppers make informed purchasing decisions.
2. Grade Calculations:
Students frequently encounter percentage calculations when determining their final grades. If a student scores 10 points out of a possible 50 points on a test, their score is 20%. This helps gauge academic performance accurately.
3. Financial Analysis:
Investors and financial analysts use percentages extensively. For example, calculating the percentage change in stock prices, or the return on investment (ROI), involves similar percentage calculations. A 10-point increase in a stock valued at 50 points represents a 20% increase.
4. Data Analysis and Statistics:
In various fields, percentages are used to represent data. For instance, if a survey shows that 10 out of 50 respondents prefer a specific product, this translates to a 20% preference rate. This helps researchers understand trends and patterns.
5. Tip Calculations:
Restaurants often encourage tipping a certain percentage of the bill. If the bill is $50 and you wish to leave a 20% tip, the calculation is straightforward: (20/100) * $50 = $10.
Beyond the Basics: Handling More Complex Percentages
While the "What percent is 10 of 50?" question provides a simple starting point, the same principles apply to more complex percentage problems. Here are some examples:
-
Finding the whole: If 20% of a number is 10, what is the number? The formula can be rearranged to solve for the whole: (Whole = Part / (Percentage/100)).
-
Finding the part: If 30% of a number (50) is what number? The formula is applied directly: (Part = (Percentage/100) * Whole).
-
Percentage increase/decrease: Calculating percentage changes involves finding the difference between two values, dividing by the original value, and multiplying by 100%. For example, if a value increases from 50 to 60, the percentage increase is ((60-50)/50) * 100% = 20%.
Mastering Percentages: Tips and Tricks
-
Practice Regularly: The more you practice percentage calculations, the more comfortable you'll become with the formulas and techniques.
-
Use a Calculator: For more complex problems or when accuracy is paramount, a calculator can be a valuable tool.
-
Visual Aids: Consider using diagrams or charts to visualize the parts and the whole, especially when working with more complex scenarios.
-
Understand the Context: Always consider the context of the problem to correctly identify the "part" and the "whole".
Conclusion: The Power of Percentage Understanding
The seemingly simple question, "What percent is 10 of 50?", opens the door to a world of practical applications of percentage calculations. By understanding the underlying principles and employing the various methods explained in this article, you can confidently tackle any percentage problem and effectively use this fundamental mathematical skill in your daily life and professional endeavors. Remember that practice and understanding the context are key to mastering percentages.
Latest Posts
Latest Posts
-
Number In Words From 1 To 100
May 14, 2025
-
What Is 96 Inches In Feet
May 14, 2025
-
What Percentage Is 35 Out Of 40
May 14, 2025
-
Electricity Is Measured In What Unit
May 14, 2025
-
Is A Pencil A Conductor Or Insulator
May 14, 2025
Related Post
Thank you for visiting our website which covers about What Percent Is 10 Of 50 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.