What Is The Value Of X 75 37
Juapaving
May 11, 2025 · 5 min read
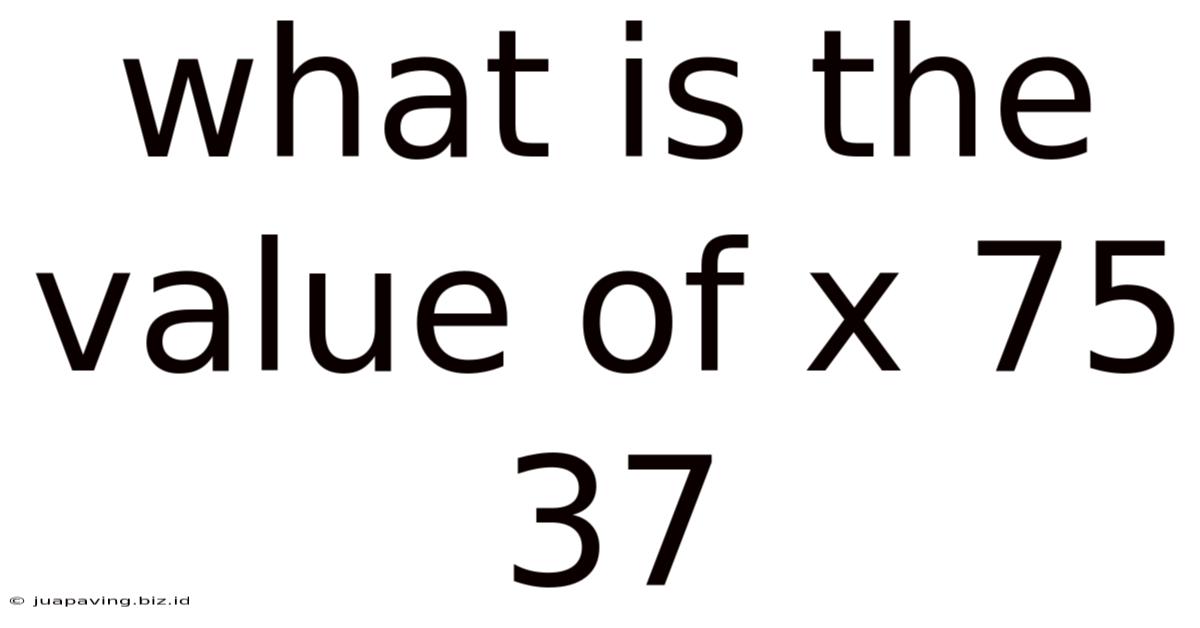
Table of Contents
What is the Value of x: Deconstructing the 75 37 Puzzle
This seemingly simple mathematical puzzle, "What is the value of x: 75 37," is deceptively complex. It's not a straightforward equation solvable with a single operation. Instead, it challenges us to think critically about mathematical relationships, pattern recognition, and the potential for multiple solutions depending on the underlying rules. This article will delve into various interpretations, explore different mathematical approaches, and ultimately demonstrate the importance of clearly defined rules in problem-solving.
Understanding the Ambiguity: Why There's No Single Answer
The core issue with "What is the value of x: 75 37" lies in the lack of an explicit operator or relationship between 75 and 37. Is it addition? Subtraction? Multiplication? Division? Or something more sophisticated? Without further information, we're left to speculate and explore possibilities. This ambiguity is precisely what makes this puzzle intriguing and challenging. It forces us to think beyond simple arithmetic and consider the broader context of mathematical logic.
The Importance of Context in Mathematics
Mathematics isn't just about numbers; it's about relationships between numbers. The context in which a problem is presented is crucial in determining its solution. In this case, the missing context is the key to unlocking the puzzle. It highlights the importance of precise mathematical notation and the dangers of relying on assumptions. A simple addition sign (+) or subtraction sign (-) would entirely change the nature of the problem, making it trivial.
Exploring Potential Solutions: A Journey Through Mathematical Possibilities
Let's explore several potential interpretations and see how different approaches can yield different answers. Keep in mind that without additional information, none of these solutions are definitively "correct" — they're all plausible interpretations given the lack of context.
1. Interpreting as a Simple Arithmetic Problem:
If we assume a simple arithmetic operation is intended, we could consider:
- Addition: 75 + 37 = 112. This is a straightforward solution, but it lacks sophistication and relies on a potentially unwarranted assumption.
- Subtraction: 75 - 37 = 38. Again, simple but equally reliant on an assumption without supporting evidence.
- Multiplication: 75 x 37 = 2775. A larger result, but still a possibility under the assumption of simple arithmetic.
- Division: 75 / 37 ≈ 2.027. This yields a decimal, which might be considered less elegant but still mathematically valid.
These simple arithmetic interpretations are the most obvious, yet they highlight the inherent ambiguity in the original question.
2. Exploring Number Properties:
We can delve deeper by examining the properties of the numbers 75 and 37:
- Prime Factorization: The prime factorization of 75 is 3 x 5 x 5, and 37 is a prime number. This doesn't immediately suggest a clear relationship, but it opens the door to exploring more advanced mathematical concepts.
- Digit Sum: The sum of digits of 75 is 12, and the sum of digits of 37 is 10. The difference is 2. Could this difference be relevant? Again, it's speculation without more context.
- Geometric Relationships: Is there a hidden geometric relationship? Perhaps the numbers represent sides of a shape, and 'x' represents an area or perimeter. This opens the door to significantly more complex interpretations.
These explorations demonstrate that even seemingly simple numbers possess intricate properties, making it crucial to consider various possibilities.
3. Advanced Mathematical Interpretations:
Let's consider more sophisticated mathematical avenues:
- Modular Arithmetic: This branch of mathematics deals with remainders. We could explore the remainders when 75 and 37 are divided by a certain number. For instance, 75 mod 10 = 5 and 37 mod 10 = 7. But without a specified modulus, this remains speculative.
- Continued Fractions: Representing the numbers as continued fractions could reveal hidden relationships, but this requires advanced mathematical knowledge and doesn't guarantee a clear solution without additional constraints.
- Number Sequences: Is 75 and 37 part of a larger sequence? Could there be a pattern that helps define the value of x? Without more numbers in the sequence, this remains purely speculative.
These interpretations showcase the potential for complex mathematical analyses; however, the absence of context prevents a definitive solution.
The Crucial Role of Context and Clear Problem Definition
The puzzle "What is the value of x: 75 37" serves as a powerful reminder of the importance of context and clear problem definition in mathematics. Without explicitly stating the relationship between 75 and 37, the problem remains inherently ambiguous. Multiple interpretations, each with potentially valid solutions, are possible.
Improving Problem Statement for Clarity:
To make the problem solvable, it needs to be significantly more precise. For example:
- "What is the value of x if x = 75 + 37?" This clearly defines the operation.
- "What is the value of x if x is the difference between 75 and 37?" This clearly defines the operation.
- "75 and 37 are consecutive terms in a Fibonacci-like sequence. What is the next term (x)?" This introduces a specific mathematical context.
Adding context transforms the puzzle into a solvable problem. This emphasis on clear communication is vital, not only in mathematics but across all fields.
Conclusion: Embracing Ambiguity and the Power of Critical Thinking
While "What is the value of x: 75 37" doesn't have a single, definitive answer, its ambiguity is valuable. It encourages critical thinking, problem-solving skills, and the exploration of various mathematical concepts. The exercise highlights the need for precision in mathematical notation and emphasizes the crucial role of context in determining solutions. This puzzle isn't about finding the answer; it's about understanding the process of formulating and solving mathematical problems, appreciating the nuances of mathematical relationships, and developing a deeper understanding of how context profoundly shapes meaning. This exploration has been a journey not just through numbers, but through the very essence of mathematical reasoning itself. It underscores that a seemingly simple problem can unravel into a rich tapestry of mathematical possibilities when the initial conditions are incomplete or poorly defined. The journey of exploration is often more valuable than the destination itself.
Latest Posts
Latest Posts
-
Which Of The Following Elements Has The Largest Atomic Radius
May 12, 2025
-
In Which Organelle Does Respiration Occur
May 12, 2025
-
Basic Functional Unit Of The Kidney
May 12, 2025
-
Which Of The Following Is Not A Characteristic Of Bonds
May 12, 2025
-
A Helicopter Lifts A 72 Kg Astronaut
May 12, 2025
Related Post
Thank you for visiting our website which covers about What Is The Value Of X 75 37 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.