What Is The Subset Of Real Numbers
Juapaving
Mar 30, 2025 · 5 min read
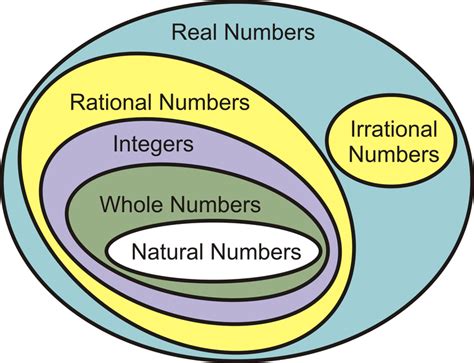
Table of Contents
What is the Subset of Real Numbers? A Comprehensive Guide
The real numbers encompass a vast landscape within mathematics, forming the foundation for countless applications in science, engineering, and everyday life. Understanding the subsets of real numbers is crucial for grasping their inherent structure and effectively employing them in various mathematical contexts. This comprehensive guide will delve into the various subsets, exploring their definitions, properties, and interrelationships.
Understanding the Real Number System
Before we dive into the subsets, let's briefly revisit the real number system. Real numbers include all rational and irrational numbers.
-
Rational Numbers: These can be expressed as a fraction p/q, where p and q are integers, and q is not zero. Examples include 1/2, -3, 0, and 7. Rational numbers can be further categorized into integers and fractions. Integers themselves are whole numbers including their negative counterparts (…,-2,-1,0,1,2,…).
-
Irrational Numbers: These cannot be expressed as a simple fraction. Their decimal representations are non-terminating and non-repeating. Famous examples include π (pi) and √2 (the square root of 2).
The real number system is complete, meaning it contains all its limit points. This completeness property is crucial for calculus and analysis. The real numbers are often represented visually on a number line, where each point corresponds to a unique real number.
Major Subsets of Real Numbers
The real numbers can be categorized into several important subsets based on their properties:
1. Natural Numbers (ℕ)
Also known as counting numbers, these are the positive integers starting from 1: {1, 2, 3, 4, ...}. They are used for counting and are the foundation for many mathematical concepts. Natural numbers are a subset of all other sets we'll discuss below.
2. Whole Numbers (W)
Whole numbers include zero and all positive integers: {0, 1, 2, 3, 4, ...}. They extend the natural numbers by including zero, crucial for representing quantities like absence or a starting point.
3. Integers (ℤ)
Integers comprise all positive and negative whole numbers, including zero: {..., -3, -2, -1, 0, 1, 2, 3, ...}. They are essential for representing quantities that can be both positive and negative, such as temperature or financial balances.
4. Rational Numbers (ℚ)
As mentioned earlier, rational numbers are numbers that can be expressed as a fraction p/q, where p and q are integers, and q ≠ 0. Examples include 1/2, -3/4, 0, and 7 (which can be written as 7/1). Their decimal representations either terminate (like 0.5) or repeat (like 0.333...).
5. Irrational Numbers (I)
Irrational numbers are real numbers that cannot be expressed as a fraction of two integers. Their decimal representations are non-terminating and non-repeating. Examples include π (approximately 3.14159...), √2 (approximately 1.41421...), and e (approximately 2.71828...).
6. Real Numbers (ℝ)
The real numbers encompass all rational and irrational numbers. They form a complete and ordered field, meaning they satisfy certain axioms that allow for arithmetic operations and comparisons. Every point on the number line corresponds to a unique real number.
Relationships Between Subsets
The subsets of real numbers are nested within each other, forming a hierarchical structure:
- ℕ ⊂ ℤ ⊂ ℚ ⊂ ℝ (Natural numbers are a subset of integers, which are a subset of rational numbers, which are a subset of real numbers)
- I ⊂ ℝ (Irrational numbers are a subset of real numbers)
- ℚ ∪ I = ℝ (The union of rational and irrational numbers makes up the entire set of real numbers)
Understanding these relationships is key to solving mathematical problems and working with various types of numbers.
Further Subsets and Classifications
Beyond these primary subsets, we can define even more specific classifications:
1. Even and Odd Numbers
These are subsets of integers.
- Even Numbers: Integers divisible by 2 (..., -4, -2, 0, 2, 4, ...).
- Odd Numbers: Integers not divisible by 2 (..., -3, -1, 1, 3, 5, ...).
2. Prime and Composite Numbers
These are subsets of natural numbers.
- Prime Numbers: Natural numbers greater than 1 that are only divisible by 1 and themselves (2, 3, 5, 7, 11, ...).
- Composite Numbers: Natural numbers greater than 1 that are not prime (4, 6, 8, 9, 10, ...).
3. Algebraic and Transcendental Numbers
These are subsets of real numbers based on their ability to be roots of polynomial equations.
- Algebraic Numbers: Real numbers that are roots of polynomial equations with integer coefficients. For instance, √2 is algebraic because it's a root of x² - 2 = 0. Rational numbers are a subset of algebraic numbers.
- Transcendental Numbers: Real numbers that are not algebraic. Examples include π and e.
4. Positive and Negative Numbers
These are broad classifications cutting across many subsets. Positive numbers are greater than zero, while negative numbers are less than zero. Zero itself is neither positive nor negative.
Applications of Subsets of Real Numbers
The understanding and application of these subsets are fundamental across various mathematical fields:
- Number Theory: Deals extensively with the properties of integers, prime numbers, and other subsets, exploring their distribution and relationships.
- Calculus: The concept of limits and continuity relies heavily on the properties of real numbers and their completeness.
- Linear Algebra: Vectors and matrices often involve operations on real numbers, their subsets, and their properties.
- Analysis: The study of continuous functions, sequences, and series relies heavily on the real number system and its subsets.
- Computer Science: Representing numbers in computers often involves understanding the limitations and properties of different subsets, particularly with regard to floating-point arithmetic and integer overflow.
Conclusion: The Importance of Understanding Subsets of Real Numbers
The various subsets of real numbers provide a rich framework for understanding the structure and properties of numbers. From the simple counting numbers to the complexities of irrational and transcendental numbers, each subset plays a crucial role in mathematical theory and its numerous applications. A strong grasp of these subsets is essential for anyone seeking a deeper understanding of mathematics and its role in the world around us. Mastering these concepts will unlock a world of advanced mathematical exploration and empower you to tackle complex problems across diverse fields. By understanding the relationships between these sets and their individual properties, you gain a powerful toolset for mathematical reasoning and problem-solving. The exploration of real numbers and their subsets is a journey of discovery that reveals the intricate beauty and profound utility of mathematics.
Latest Posts
Latest Posts
-
P Block Elements In Periodic Table
Apr 01, 2025
-
Adjective That Starts With L To Describe A Mom
Apr 01, 2025
-
Is 35 A Multiple Of 6
Apr 01, 2025
-
Exponents Worksheets Pdf With Answers 7th
Apr 01, 2025
-
Is Chlorine A Pure Substance Or Mixture
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about What Is The Subset Of Real Numbers . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
