What Is The Relationship Between Density Mass And Volume
Juapaving
Mar 29, 2025 · 7 min read
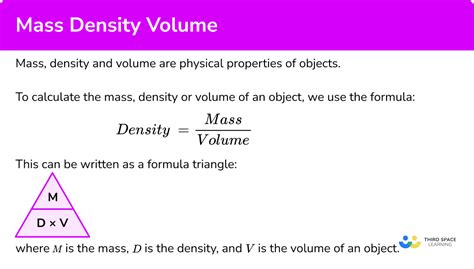
Table of Contents
What is the Relationship Between Density, Mass, and Volume?
Understanding the relationship between density, mass, and volume is fundamental to many areas of science and engineering. This seemingly simple concept underpins our understanding of everything from floating objects to the formation of stars. This comprehensive guide will explore this relationship in detail, providing clear explanations, real-world examples, and practical applications.
Defining the Key Terms
Before diving into the relationship, let's clearly define each term:
1. Mass: Mass is a measure of the amount of matter in an object. It's a scalar quantity, meaning it only has magnitude (size) and no direction. We typically measure mass in kilograms (kg), grams (g), or other related units. Think of it as the "stuff" that makes up an object. A heavier object contains more mass.
2. Volume: Volume is the amount of three-dimensional space occupied by an object or substance. It's also a scalar quantity. Common units for volume include cubic meters (m³), cubic centimeters (cm³), and liters (L). Imagine filling a container with water; the amount of water represents the volume of the container.
3. Density: Density is the measure of mass per unit volume. It describes how much mass is packed into a given volume. It's a derived quantity, meaning it's calculated from other measurements (mass and volume). Density is typically expressed in kilograms per cubic meter (kg/m³) or grams per cubic centimeter (g/cm³). A denser object has more mass packed into the same volume compared to a less dense object.
The Fundamental Relationship: The Density Formula
The relationship between density, mass, and volume is expressed by a simple yet powerful formula:
Density (ρ) = Mass (m) / Volume (V)
This formula states that density is directly proportional to mass and inversely proportional to volume. This means:
-
If mass increases and volume stays the same, density increases. Imagine compressing a sponge; its mass remains the same, but its volume decreases, leading to an increase in density.
-
If volume increases and mass stays the same, density decreases. Imagine stretching a piece of dough; its mass remains the same, but its volume increases, resulting in a decrease in density.
-
If both mass and volume increase proportionally, density remains constant. If you double both the mass and volume of an object, its density remains the same.
Understanding Density in Different States of Matter
Density varies significantly depending on the state of matter (solid, liquid, or gas):
1. Solids: Solids generally have the highest densities because their particles are tightly packed together. The strong intermolecular forces hold the particles in a fixed arrangement, resulting in a smaller volume for a given mass. Examples include metals (iron, gold), rocks, and wood.
2. Liquids: Liquids have intermediate densities compared to solids and gases. Their particles are closer together than in gases but more loosely packed than in solids. The particles can move around, giving liquids the ability to flow and take the shape of their container. Examples include water, oil, and mercury.
3. Gases: Gases have the lowest densities because their particles are far apart and move randomly. The weak intermolecular forces allow the particles to spread out, resulting in a large volume for a given mass. Examples include air, helium, and oxygen.
Practical Applications of Density
The concept of density is crucial in numerous applications across various fields:
1. Material Science and Engineering: Density is a critical factor in selecting materials for specific applications. For example, lightweight materials with low density are preferred in aerospace engineering to reduce fuel consumption, while high-density materials are essential in construction for strength and stability.
2. Oceanography and Meteorology: Density differences in ocean water (due to salinity and temperature) drive ocean currents, while density variations in the atmosphere influence weather patterns. Understanding density helps predict ocean circulation and weather phenomena.
3. Geology and Geophysics: The density of rocks and minerals provides valuable information about the Earth's structure and composition. Geophysicists use density measurements to interpret seismic data and explore for resources.
4. Medicine: Density measurements are used in various medical diagnostic techniques, such as bone densitometry (measuring bone density to detect osteoporosis) and blood tests (measuring blood density to assess hydration levels).
5. Archimedes' Principle and Buoyancy: Archimedes' principle states that an object immersed in a fluid experiences an upward buoyant force equal to the weight of the fluid displaced by the object. Whether an object floats or sinks depends on the comparison between its density and the density of the fluid. If an object's density is less than the fluid's density, it floats; otherwise, it sinks. This principle is fundamental in ship design, submarine technology, and understanding flotation devices.
Calculating Density: Examples and Worked Problems
Let's illustrate the density calculation with some examples:
Example 1: Calculating the density of a block of wood.
A block of wood has a mass of 500 grams and a volume of 625 cubic centimeters. What is its density?
Using the formula:
Density = Mass / Volume = 500 g / 625 cm³ = 0.8 g/cm³
Therefore, the density of the wood is 0.8 g/cm³.
Example 2: Calculating the mass of a liquid.
A container holds 2 liters (2000 cm³) of a liquid with a density of 1.2 g/cm³. What is the mass of the liquid?
Rearranging the density formula to solve for mass:
Mass = Density × Volume = 1.2 g/cm³ × 2000 cm³ = 2400 g = 2.4 kg
Therefore, the mass of the liquid is 2.4 kg.
Example 3: Calculating the volume of a solid.
A metal sphere has a mass of 1 kg (1000 g) and a density of 7.8 g/cm³. What is its volume?
Rearranging the density formula to solve for volume:
Volume = Mass / Density = 1000 g / 7.8 g/cm³ ≈ 128.2 cm³
Therefore, the volume of the metal sphere is approximately 128.2 cm³.
Relative Density and Specific Gravity
Related concepts often encountered alongside density include relative density and specific gravity:
Relative Density: Relative density compares the density of a substance to the density of a reference substance, usually water at 4°C. It's a dimensionless quantity (no units). A relative density of 1 indicates that the substance has the same density as water; a relative density greater than 1 indicates that the substance is denser than water, and a relative density less than 1 indicates that the substance is less dense than water.
Specific Gravity: Specific gravity is essentially the same as relative density. It's the ratio of the density of a substance to the density of a reference substance (usually water). Specific gravity is also a dimensionless quantity.
Both relative density and specific gravity are useful for comparing the densities of different substances without considering the units of measurement.
Advanced Concepts and Applications
The relationship between density, mass, and volume extends into more complex areas:
1. Density Gradient: Density gradients exist in many natural systems, such as the Earth's mantle and the ocean. These gradients drive processes like convection and mixing.
2. Density and Pressure: In fluids, pressure increases with depth due to the weight of the overlying fluid. This relationship is influenced by the fluid's density.
3. Density and Temperature: The density of most substances changes with temperature. Generally, density decreases as temperature increases (except for water between 0°C and 4°C).
4. Density and Phase Transitions: Density changes dramatically during phase transitions (e.g., melting, boiling). The density of ice is lower than that of liquid water, which is why ice floats.
5. Computational Fluid Dynamics (CFD): Density is a crucial parameter in CFD simulations used to model fluid flow and heat transfer in complex systems.
Conclusion
The relationship between density, mass, and volume is a fundamental principle in physics and has far-reaching applications in various fields. Understanding this relationship is crucial for solving problems related to material selection, fluid dynamics, and many other scientific and engineering disciplines. The simple formula, Density = Mass / Volume, serves as a foundation for understanding the behaviour of matter in various states and situations. From designing efficient aircraft to understanding ocean currents, the concept of density remains an indispensable tool in our quest to comprehend the world around us.
Latest Posts
Related Post
Thank you for visiting our website which covers about What Is The Relationship Between Density Mass And Volume . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
