What Is The Perimeter Of A Parallelogram
Juapaving
Apr 03, 2025 · 5 min read
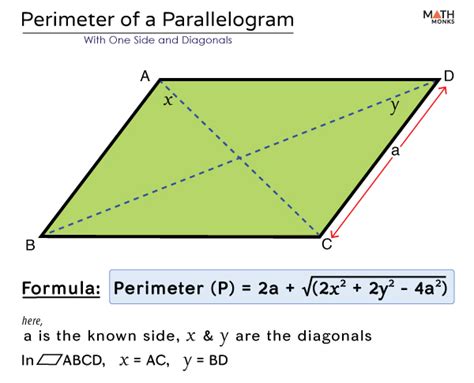
Table of Contents
What is the Perimeter of a Parallelogram? A Comprehensive Guide
Understanding the perimeter of a parallelogram is fundamental in geometry and has practical applications in various fields, from architecture and engineering to everyday problem-solving. This comprehensive guide will delve into the concept of a parallelogram, explain how to calculate its perimeter, explore different types of parallelograms, and provide numerous examples to solidify your understanding. We'll also touch upon related concepts and advanced applications.
Understanding Parallelograms
A parallelogram is a quadrilateral (a four-sided polygon) with two pairs of parallel sides. This unique property leads to several important characteristics:
- Opposite sides are equal in length: If we label the sides of a parallelogram as a and b, then the opposite sides are also a and b.
- Opposite angles are equal in measure: The angles opposite each other within the parallelogram are congruent.
- Consecutive angles are supplementary: This means that the sum of any two adjacent angles is 180 degrees.
Calculating the Perimeter of a Parallelogram
The perimeter of any polygon is simply the total distance around its exterior. For a parallelogram, since opposite sides are equal, the perimeter is calculated by adding the lengths of all four sides. This can be expressed concisely as:
Perimeter = 2(a + b)
Where:
- a represents the length of one side.
- b represents the length of an adjacent side.
This formula streamlines the calculation, eliminating the need to measure each side individually. Let's illustrate with some examples:
Example 1: A Simple Parallelogram
Imagine a parallelogram with sides of length 5 cm and 8 cm. Using the formula:
Perimeter = 2(5 cm + 8 cm) = 2(13 cm) = 26 cm
The perimeter of this parallelogram is 26 cm.
Example 2: Real-World Application - Fencing a Field
A farmer needs to fence a rectangular field (a rectangle is a special type of parallelogram). The field measures 20 meters in length and 15 meters in width. What length of fencing is required?
Here, 'a' = 20 meters and 'b' = 15 meters.
Perimeter = 2(20 meters + 15 meters) = 2(35 meters) = 70 meters
The farmer needs 70 meters of fencing.
Example 3: A Parallelogram with Decimal Values
Let's consider a parallelogram with sides measuring 3.5 inches and 6.2 inches.
Perimeter = 2(3.5 inches + 6.2 inches) = 2(9.7 inches) = 19.4 inches
The perimeter is 19.4 inches.
Different Types of Parallelograms
Several special types of parallelograms exist, each with its unique properties:
Rectangle
A rectangle is a parallelogram where all four angles are right angles (90 degrees). The perimeter calculation remains the same: 2(a + b), where 'a' and 'b' are the lengths of adjacent sides (often referred to as length and width).
Square
A square is a special case of a rectangle (and therefore a parallelogram) where all four sides are equal in length. The perimeter calculation simplifies to:
Perimeter = 4a
Where 'a' is the length of one side.
Rhombus
A rhombus is a parallelogram with all four sides equal in length. While its angles are not necessarily right angles, the perimeter calculation is similar to a square:
Perimeter = 4a
Where 'a' is the length of one side.
Rhomboid
A rhomboid is a parallelogram that is neither a rectangle nor a rhombus. It has two pairs of equal, parallel sides, but the sides are not all equal in length, and the angles are not all right angles. The perimeter calculation remains the same as the general parallelogram formula: 2(a + b).
Advanced Concepts and Applications
The concept of the parallelogram's perimeter extends beyond simple calculations. It plays a vital role in:
-
Area Calculation: While the perimeter gives the distance around the parallelogram, the area provides the space enclosed within it. The area of a parallelogram is calculated as: Area = base * height, where the base is the length of one side and the height is the perpendicular distance between that side and its opposite side.
-
Vector Geometry: Parallelograms are fundamental in vector algebra. The sides of a parallelogram can represent vectors, and the perimeter relates to the magnitudes of these vectors.
-
Coordinate Geometry: Using coordinate systems, you can determine the lengths of the sides of a parallelogram plotted on a graph and hence calculate its perimeter using the distance formula.
-
Calculus: Parallelograms appear in calculus problems involving double integrals and area calculations of more complex shapes.
-
Engineering and Architecture: Parallelograms are common shapes in various engineering and architectural designs, where perimeter calculations are crucial for material estimation, cost calculations, and structural integrity.
Solving More Complex Problems
Let's tackle a more complex problem that integrates multiple geometric concepts:
Problem: A parallelogram has one side measuring 12 cm and an adjacent angle of 60 degrees. The height corresponding to the 12cm side is 10 cm. Calculate the perimeter of the parallelogram.
Solution:
-
Find the length of the adjacent side (b): We can use trigonometry (specifically, the sine function) since we have an angle and the height. The height creates a right-angled triangle with the 12cm side as the hypotenuse and the height as the opposite side to the 60-degree angle. Sin(60°) = height/12cm. Solving for height gives approximately 10.39 cm. This is the adjacent side, b.
-
Calculate the perimeter: Now we have a = 12 cm and b = 10.39 cm (approximately).
Perimeter = 2(12cm + 10.39cm) = 2(22.39cm) = 44.78 cm (approximately).
Conclusion
Understanding the perimeter of a parallelogram is a fundamental skill in geometry with practical applications in diverse fields. Mastering the basic formula and its application to different types of parallelograms provides a solid foundation for tackling more complex geometric problems and real-world applications. Remember to always consider the specific characteristics of the parallelogram and use the appropriate formula for accurate calculations. By combining the conceptual understanding with practical problem-solving, you can unlock a deeper appreciation of the versatile parallelogram and its role in mathematics and beyond.
Latest Posts
Latest Posts
-
Five Letter Words With Two A
Apr 04, 2025
-
Label The Parts Of The Animal Cell
Apr 04, 2025
-
Land Surrounded By Water On 3 Sides
Apr 04, 2025
-
Compare And Contrast Mixtures And Solutions
Apr 04, 2025
-
Darkest Part Of The Moons Shadow
Apr 04, 2025
Related Post
Thank you for visiting our website which covers about What Is The Perimeter Of A Parallelogram . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
