What Is The Length Of A Line Segment
Juapaving
Apr 02, 2025 · 6 min read
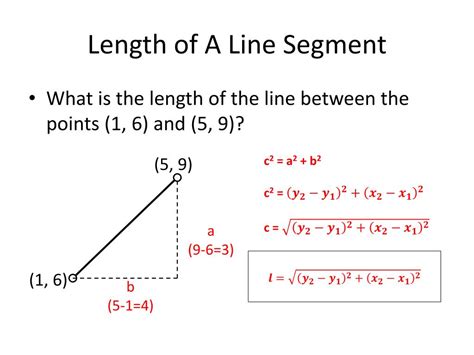
Table of Contents
- What Is The Length Of A Line Segment
- Table of Contents
- What is the Length of a Line Segment? A Comprehensive Guide
- Defining a Line Segment
- Distinguishing a Line Segment from Other Geometric Entities
- Measuring the Length of a Line Segment
- Using Rulers and Measuring Tapes
- Coordinate Geometry Approach
- Extending to Three Dimensions
- Properties of Line Segment Length
- Applications of Line Segment Length
- Geometry and Trigonometry
- Computer Graphics and Computer-Aided Design (CAD)
- Physics and Engineering
- Cartography and Geographic Information Systems (GIS)
- Real-world Applications
- Advanced Concepts Related to Line Segment Length
- Arc Length
- Fractal Geometry
- Vector Spaces and Linear Algebra
- Conclusion
- Latest Posts
- Latest Posts
- Related Post
What is the Length of a Line Segment? A Comprehensive Guide
The seemingly simple question, "What is the length of a line segment?", opens a door to a fascinating exploration of geometry, measurement, and the fundamental concepts that underpin our understanding of space. While intuitively we grasp the idea of a line segment's length, delving deeper reveals subtleties and nuances that are crucial for various applications, from basic arithmetic to advanced calculus. This comprehensive guide will unravel the intricacies of line segment length, covering its definition, measurement, properties, and applications in diverse fields.
Defining a Line Segment
Before we dive into the measurement, let's precisely define what a line segment is. A line segment is a part of a line that is bounded by two distinct endpoints. It represents the shortest distance between these two points. Unlike a line which extends infinitely in both directions, a line segment has a definite beginning and end. This finite nature is crucial when determining its length. We can represent a line segment using notation such as AB, where A and B represent the endpoints.
Distinguishing a Line Segment from Other Geometric Entities
It's essential to differentiate a line segment from other related geometric entities:
- Line: A line is a straight path that extends infinitely in both directions. It has no endpoints.
- Ray: A ray is a part of a line that starts at a point and extends infinitely in one direction. It has one endpoint.
- Curve: A curve is a continuous path that is not necessarily straight.
Understanding these distinctions is vital for accurate geometrical reasoning and calculations. The length is a property only applicable to line segments and curves (where we define arc length).
Measuring the Length of a Line Segment
The measurement of a line segment's length hinges on the concept of distance. The length represents the distance between the two endpoints. The method of measurement depends on the context and the tools available:
Using Rulers and Measuring Tapes
For practical measurements in everyday scenarios, rulers and measuring tapes are the most common tools. The process involves aligning one endpoint of the segment with the zero mark of the ruler and observing the mark corresponding to the other endpoint. The difference between these two marks represents the length of the line segment. The units of measurement, typically centimeters, inches, or meters, depend on the scale of the ruler or measuring tape.
Coordinate Geometry Approach
In coordinate geometry, we can determine the length of a line segment using the distance formula. If we have the coordinates of the two endpoints, (x₁, y₁) and (x₂, y₂), the distance (and therefore the length) is calculated using the following formula:
d = √[(x₂ - x₁)² + (y₂ - y₁)²]
This formula is derived from the Pythagorean theorem, which relates the lengths of the sides of a right-angled triangle. The distance formula extends this concept to find the distance between any two points in a Cartesian coordinate system. This method is particularly useful for calculations in two-dimensional spaces.
Extending to Three Dimensions
The distance formula can be extended to three-dimensional space. If we have the coordinates (x₁, y₁, z₁) and (x₂, y₂, z₂) of the endpoints in a three-dimensional Cartesian coordinate system, the distance is:
d = √[(x₂ - x₁)² + (y₂ - y₁)² + (z₂ - z₁)²]
This expansion allows for calculating the length of line segments in three-dimensional spaces, crucial for applications in fields like physics and engineering.
Properties of Line Segment Length
The length of a line segment possesses several important properties:
- Non-negativity: The length of a line segment is always non-negative. It cannot be a negative value.
- Uniqueness: A line segment has only one length.
- Additivity: If a point C lies on the line segment AB, then the length of AC plus the length of CB equals the length of AB. This is a crucial property for many geometric proofs and constructions.
- Invariance under Rigid Transformations: The length of a line segment remains unchanged under rigid transformations such as translations, rotations, and reflections. This property is fundamental in geometry and ensures that the length is a consistent measure regardless of the segment's position or orientation.
Applications of Line Segment Length
The concept of line segment length is foundational across many disciplines:
Geometry and Trigonometry
Line segment lengths are the building blocks of many geometric theorems and constructions. Calculating the lengths of sides and diagonals in polygons, determining the altitudes of triangles, and solving problems involving similar triangles all rely heavily on the concept of line segment length. Trigonometry utilizes line segment lengths to relate angles and sides of triangles.
Computer Graphics and Computer-Aided Design (CAD)
In computer graphics and CAD software, line segments are fundamental primitives. The precise calculation and manipulation of line segment lengths are essential for creating and rendering two-dimensional and three-dimensional models. Algorithms for drawing lines, calculating distances between objects, and performing geometric operations all rely on accurate length calculations.
Physics and Engineering
In physics and engineering, line segments are used to represent distances and vectors. Calculating distances between points, determining the magnitudes of forces, and analyzing motion all involve determining line segment lengths. For example, the calculation of work done by a force involves the displacement vector, which is essentially a line segment.
Cartography and Geographic Information Systems (GIS)
In cartography and GIS, line segments are used to represent roads, rivers, and boundaries. The length of these line segments represents distances on the Earth's surface. Accurate measurement of these lengths is critical for mapmaking, navigation, and geographic analysis.
Real-world Applications
The applications extend to everyday tasks:
- Construction: Measuring distances for building foundations, laying out roads, or determining the dimensions of structures.
- Surveying: Determining the distances between points in the landscape for land measurement and mapping.
- Navigation: Calculating distances and routes using GPS systems and map applications.
Advanced Concepts Related to Line Segment Length
The concept of line segment length opens the door to more advanced mathematical concepts:
Arc Length
While the discussion has focused on straight line segments, the concept of length extends to curved lines. The arc length of a curve is the distance along the curve between two points. Calculating arc length requires techniques from calculus, involving integration.
Fractal Geometry
Fractal geometry explores shapes with infinite detail at arbitrarily small scales. Measuring the length of a fractal curve requires careful consideration of the scale of measurement, as the length can change depending on the level of detail considered.
Vector Spaces and Linear Algebra
In linear algebra, vectors are often represented by line segments. The length of a vector, its magnitude, is a crucial concept for vector operations and calculations.
Conclusion
The length of a line segment, although seemingly simple, is a cornerstone concept in mathematics and its applications. From basic measurements with a ruler to sophisticated calculations in coordinate geometry and calculus, the ability to determine and understand the length of a line segment is paramount. This fundamental concept underpins a wide range of disciplines, enabling the precise modeling and analysis of the physical world and its representations in computational systems. The ongoing exploration and development of these concepts continue to enrich our understanding of space, measurement, and the universe around us.
Latest Posts
Latest Posts
-
Who Is The Father Of Bio
Apr 06, 2025
-
What Are Vertices In A Triangle
Apr 06, 2025
-
Descriptive Words That Start With F
Apr 06, 2025
-
When A Sperm And Egg Combine It Is Called
Apr 06, 2025
-
How Many Centimeters Is 13 In
Apr 06, 2025
Related Post
Thank you for visiting our website which covers about What Is The Length Of A Line Segment . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
