What Is The Greatest Common Factor Of 8 And 20
Juapaving
May 10, 2025 · 5 min read
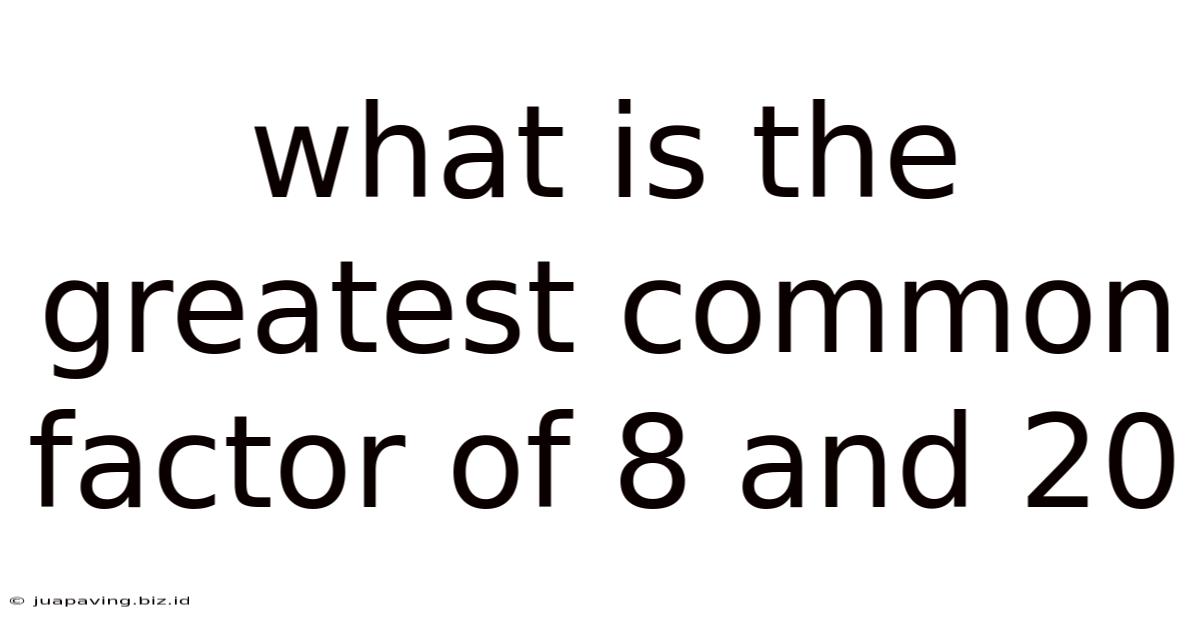
Table of Contents
What is the Greatest Common Factor of 8 and 20? A Deep Dive into Number Theory
Finding the greatest common factor (GCF) of two numbers might seem like a simple task, especially for smaller numbers like 8 and 20. However, understanding the underlying concepts and different methods for calculating the GCF is crucial for grasping fundamental number theory and its applications in various fields, from cryptography to computer science. This article delves into the world of GCF, exploring multiple approaches to determine the GCF of 8 and 20, and then extending the concepts to tackle more complex scenarios.
Understanding the Greatest Common Factor (GCF)
The greatest common factor (GCF), also known as the greatest common divisor (GCD), of two or more integers is the largest positive integer that divides each of the integers without leaving a remainder. In simpler terms, it's the biggest number that perfectly divides both numbers. For instance, the factors of 8 are 1, 2, 4, and 8, while the factors of 20 are 1, 2, 4, 5, 10, and 20. The common factors are 1, 2, and 4. The greatest of these common factors is 4. Therefore, the GCF of 8 and 20 is 4.
Why is the GCF Important?
The GCF is a fundamental concept in mathematics with far-reaching applications. Here are a few examples:
-
Simplifying Fractions: The GCF is used to simplify fractions to their lowest terms. For example, the fraction 20/8 can be simplified to 5/2 by dividing both the numerator and denominator by their GCF (4).
-
Solving Algebraic Equations: The GCF plays a role in factoring algebraic expressions, which is essential for solving many algebraic equations.
-
Geometry and Measurement: The GCF helps in solving problems related to area, volume, and measurement where we need to find the largest possible common unit.
-
Computer Science and Cryptography: GCF calculations are fundamental in certain cryptographic algorithms and computer science applications, particularly those related to modular arithmetic.
Methods for Finding the GCF
Several methods exist for determining the GCF of two numbers. Let's explore three common approaches:
1. Listing Factors
This is the most straightforward method, especially for smaller numbers. We list all the factors of each number and then identify the largest factor that is common to both.
Factors of 8: 1, 2, 4, 8 Factors of 20: 1, 2, 4, 5, 10, 20
Common Factors: 1, 2, 4 Greatest Common Factor (GCF): 4
This method becomes less efficient when dealing with larger numbers, as listing all factors can be time-consuming.
2. Prime Factorization
This method utilizes the prime factorization of each number. The prime factorization is the expression of a number as the product of its prime factors.
- Prime Factorization of 8: 2 x 2 x 2 = 2³
- Prime Factorization of 20: 2 x 2 x 5 = 2² x 5
To find the GCF using prime factorization, identify the common prime factors and multiply them together. In this case, both 8 and 20 share two factors of 2. Therefore:
GCF (8, 20) = 2 x 2 = 4
This method is more efficient than listing factors, especially for larger numbers, because it reduces the need to list all factors.
3. Euclidean Algorithm
The Euclidean algorithm is a highly efficient method for finding the GCF of two numbers, particularly useful for larger numbers. It's based on the principle that the GCF of two numbers does not change if the larger number is replaced by its difference with the smaller number. This process is repeated until the two numbers are equal.
Let's apply the Euclidean algorithm to find the GCF of 8 and 20:
- Divide the larger number (20) by the smaller number (8): 20 ÷ 8 = 2 with a remainder of 4.
- Replace the larger number with the remainder: Now we find the GCF of 8 and 4.
- Divide the larger number (8) by the smaller number (4): 8 ÷ 4 = 2 with a remainder of 0.
- Since the remainder is 0, the GCF is the last non-zero remainder, which is 4.
Therefore, the GCF of 8 and 20 using the Euclidean algorithm is 4. This method is significantly more efficient than the previous two methods for larger numbers.
Extending the Concepts: GCF of More Than Two Numbers
The methods described above can be extended to find the GCF of more than two numbers. For example, let's find the GCF of 8, 20, and 24.
Prime Factorization Method:
- Prime Factorization of 8: 2³
- Prime Factorization of 20: 2² x 5
- Prime Factorization of 24: 2³ x 3
The common prime factor is 2, and the lowest power of 2 present in all three factorizations is 2². Therefore, the GCF(8, 20, 24) = 2² = 4.
Euclidean Algorithm Extension:
The Euclidean algorithm can be extended to more than two numbers by finding the GCF of the first two numbers, and then finding the GCF of the result and the next number, and so on. For example:
- GCF(8, 20) = 4 (using the Euclidean algorithm as shown previously)
- GCF(4, 24) = 4 (using the Euclidean algorithm again)
Therefore, the GCF(8, 20, 24) = 4.
Real-World Applications of GCF
The GCF is not just a mathematical curiosity; it has practical applications in various fields:
-
Construction and Engineering: Determining the optimal dimensions for building materials often involves finding the GCF to ensure efficient cutting and minimal waste.
-
Music Theory: The GCF plays a role in understanding musical intervals and harmonies.
-
Data Compression: In computer science, the GCF is used in some data compression algorithms.
-
Scheduling and Planning: Finding the GCF can be helpful in scheduling tasks or events that need to occur at regular intervals.
-
Cryptography: The Euclidean algorithm, which is used to calculate the GCF, is a core component of several cryptographic techniques.
Conclusion
Finding the greatest common factor is a fundamental skill in mathematics with widespread applications. While simple for small numbers, understanding the underlying principles and different methods, such as listing factors, prime factorization, and the Euclidean algorithm, allows for efficient calculation even with larger numbers and multiple inputs. The versatility of the GCF makes it a valuable tool in various fields, highlighting its significance beyond the classroom. The GCF of 8 and 20, as demonstrated throughout this article, is definitively 4, and understanding how to arrive at this answer unlocks a deeper appreciation for the elegance and practicality of number theory.
Latest Posts
Latest Posts
-
9 Centimeters Is How Many Millimeters
May 10, 2025
-
The Change From A Gas To A Liquid Is Called
May 10, 2025
-
Compare And Contrast Tendons And Ligaments
May 10, 2025
-
Correct Sequence Of Events In Phagocytosis
May 10, 2025
-
Which Type Of Biomolecule Is Atp
May 10, 2025
Related Post
Thank you for visiting our website which covers about What Is The Greatest Common Factor Of 8 And 20 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.