What Is The Fraction For 40 Percent
Juapaving
May 11, 2025 · 4 min read
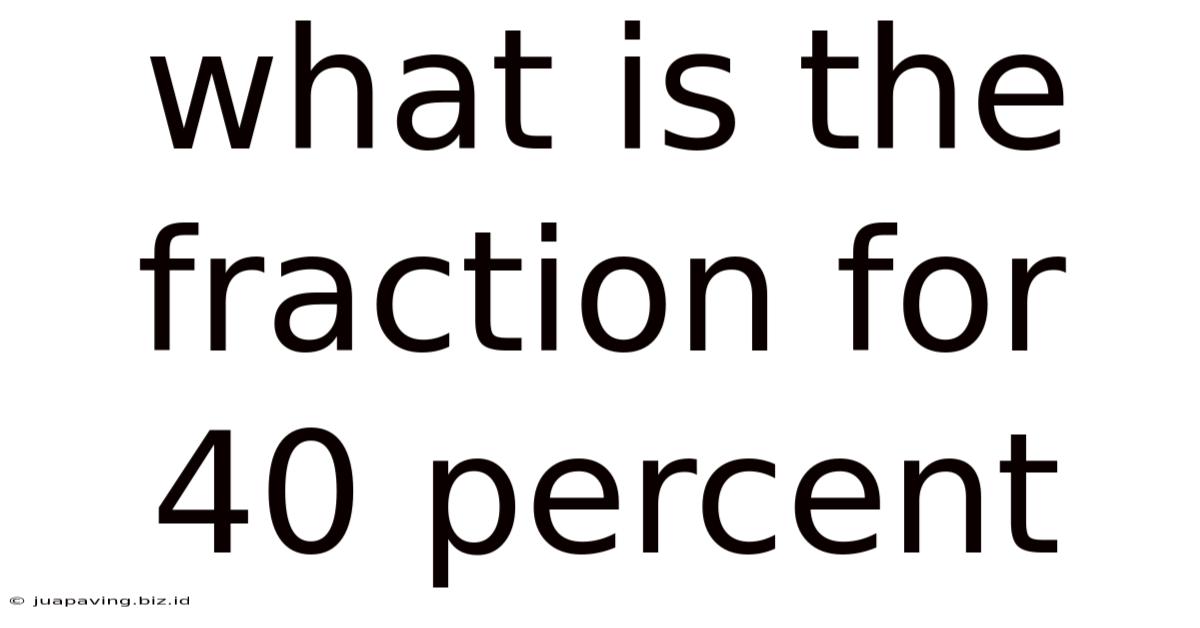
Table of Contents
What is the Fraction for 40 Percent? A Comprehensive Guide
Understanding percentages and their fractional equivalents is a fundamental skill in mathematics with widespread applications in everyday life, from calculating discounts and taxes to understanding statistics and financial data. This comprehensive guide delves into the question: What is the fraction for 40 percent? We will not only answer this directly but also explore the broader concepts of converting percentages to fractions and simplifying fractions to their lowest terms. We’ll also look at practical examples and common mistakes to avoid.
Understanding Percentages and Fractions
Before we tackle the specific problem, let's establish a clear understanding of percentages and fractions.
What is a Percentage?
A percentage represents a fraction of 100. The word "percent" literally means "per hundred." So, 40 percent means 40 out of 100. This can be written as 40/100.
What is a Fraction?
A fraction represents a part of a whole. It consists of a numerator (the top number) and a denominator (the bottom number). The numerator indicates how many parts are being considered, while the denominator shows the total number of equal parts the whole is divided into.
Converting Percentages to Fractions: A Step-by-Step Guide
The process of converting a percentage to a fraction is straightforward:
-
Write the percentage as a fraction with a denominator of 100. For example, 40% becomes 40/100.
-
Simplify the fraction (if possible). This involves finding the greatest common divisor (GCD) of the numerator and the denominator and dividing both by it.
Let's apply this to our problem:
What is the fraction for 40 percent?
-
Write as a fraction: 40/100
-
Simplify: Both 40 and 100 are divisible by 10 (and by 20, which is the GCD). Dividing both the numerator and the denominator by 20 gives us:
40 ÷ 20 = 2 100 ÷ 20 = 5
Therefore, the simplified fraction for 40 percent is 2/5.
Working with Different Percentages: Examples
Let's explore a few more examples to solidify the conversion process:
Example 1: Converting 75% to a fraction
-
Write as a fraction: 75/100
-
Simplify: Both 75 and 100 are divisible by 25. 75 ÷ 25 = 3 and 100 ÷ 25 = 4.
Therefore, 75% = 3/4
Example 2: Converting 15% to a fraction
-
Write as a fraction: 15/100
-
Simplify: Both 15 and 100 are divisible by 5. 15 ÷ 5 = 3 and 100 ÷ 5 = 20.
Therefore, 15% = 3/20
Example 3: Converting 2% to a fraction
-
Write as a fraction: 2/100
-
Simplify: Both 2 and 100 are divisible by 2. 2 ÷ 2 = 1 and 100 ÷ 2 = 50.
Therefore, 2% = 1/50
Common Mistakes to Avoid When Converting Percentages to Fractions
While the conversion process is relatively simple, some common mistakes can lead to incorrect results. Here are a few to watch out for:
-
Forgetting to simplify: Leaving the fraction as 40/100 is technically correct, but it's not the most simplified form, making it less useful for calculations and comparisons. Always simplify to the lowest terms.
-
Incorrect simplification: Ensure you divide both the numerator and denominator by the greatest common divisor to fully simplify the fraction. Dividing by a smaller common factor will result in a fraction that is not fully simplified.
-
Misunderstanding percentage meaning: Remember that a percentage represents a part of 100. This understanding is crucial for setting up the initial fraction correctly.
Practical Applications of Percentage to Fraction Conversion
The ability to convert percentages to fractions is vital in numerous real-world scenarios:
-
Cooking and Baking: Recipes often use percentages for ingredient ratios. Converting these percentages to fractions allows for easier measurement and scaling of recipes.
-
Finance: Calculating interest rates, discounts, and tax amounts often involves converting percentages to fractions for easier computation.
-
Statistics: Understanding data represented in percentages often requires converting them to fractions for deeper analysis and comparisons.
-
Probability: Many probability problems involve percentages which can be converted to fractions for calculation of probabilities.
Beyond 40%: Expanding your Understanding
While we've focused on 40%, the principles discussed apply to any percentage. By mastering the conversion process and understanding fraction simplification, you can confidently tackle any percentage-to-fraction conversion problem. Remember the key steps:
- Write the percentage as a fraction over 100.
- Find the greatest common divisor (GCD) of the numerator and denominator.
- Divide both the numerator and denominator by the GCD to simplify.
Conclusion: Mastering Percentage to Fraction Conversions
Converting 40% to its fractional equivalent of 2/5 is a straightforward process once you understand the underlying principles of percentages and fractions. This skill is invaluable across many disciplines and daily life situations. By avoiding common mistakes and practicing regularly, you'll become proficient in converting percentages to fractions, unlocking a deeper understanding of mathematical concepts and problem-solving abilities. Remember to always simplify your fractions to their lowest terms for clarity and efficiency.
Latest Posts
Latest Posts
-
Simplify The Square Root Of 112
May 11, 2025
-
10 Letter Words That Start With C
May 11, 2025
-
How Many Centimeters Are Equal To 35 Kilometers
May 11, 2025
-
Is 101 A Prime Or Composite Number
May 11, 2025
-
1 To The Power Of 8
May 11, 2025
Related Post
Thank you for visiting our website which covers about What Is The Fraction For 40 Percent . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.