What Is The Derivative Of 5x
Juapaving
May 12, 2025 · 6 min read
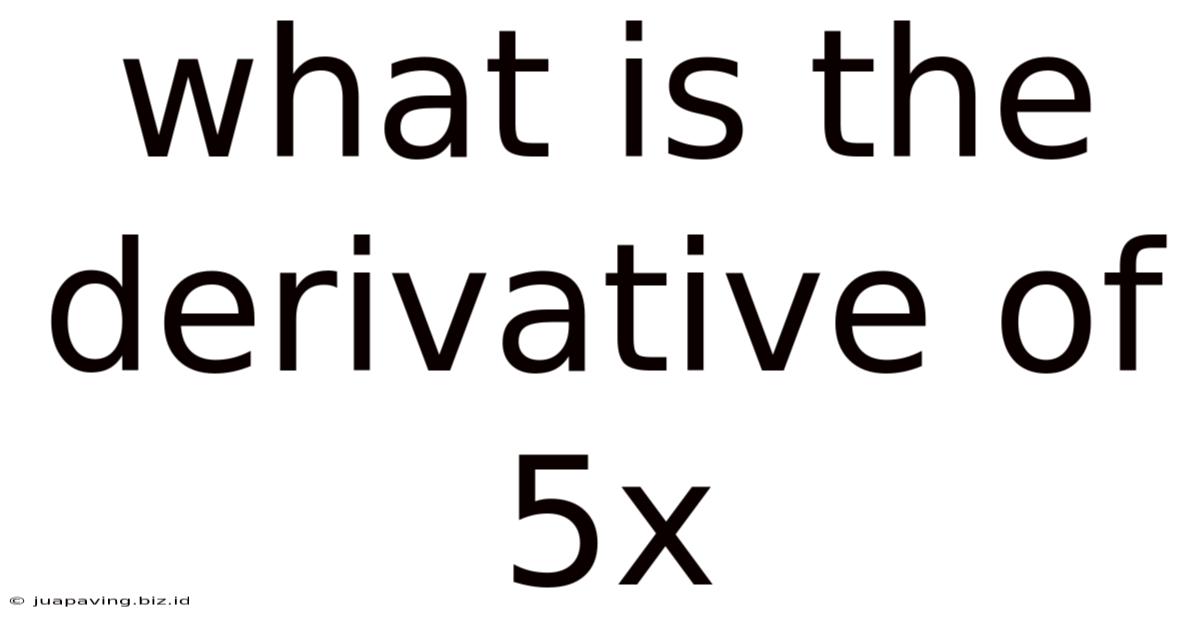
Table of Contents
What is the Derivative of 5x? A Comprehensive Guide
Finding the derivative of a function is a fundamental concept in calculus. It represents the instantaneous rate of change of the function at any given point. Understanding derivatives is crucial for various applications, from optimizing business processes to modeling physical phenomena. This article delves into the derivative of the simple function 5x, explaining the process step-by-step and exploring the broader implications of this calculation.
Understanding Derivatives: A Quick Refresher
Before we tackle the derivative of 5x, let's briefly review the core concept of derivatives. The derivative of a function, often denoted as f'(x) or dy/dx, measures the slope of the tangent line to the function's graph at a specific point. Geometrically, it represents the instantaneous rate at which the function's value is changing.
The process of finding a derivative is called differentiation. There are several rules and techniques used in differentiation, and the specific method applied depends on the complexity of the function. For simple functions like 5x, we can apply basic differentiation rules.
The Power Rule: The Key to Differentiating 5x
The simplest and most commonly used rule in differentiation is the power rule. This rule states that the derivative of x<sup>n</sup> is nx<sup>n-1</sup>, where 'n' is a constant.
Let's break down this rule:
- x<sup>n</sup>: This represents a variable raised to a power.
- n: This is the exponent, a constant number.
- nx<sup>n-1</sup>: This is the derivative. We multiply the original function by the exponent and then reduce the exponent by 1.
Applying the Power Rule to 5x
Now, let's apply the power rule to find the derivative of 5x. We can rewrite 5x as 5x<sup>1</sup>. Here, '5' is a constant coefficient, and 'x' is raised to the power of 1.
Following the power rule:
-
Multiply by the exponent: The exponent is 1, so we multiply 5x<sup>1</sup> by 1: 1 * 5x<sup>1</sup> = 5x<sup>1</sup>.
-
Reduce the exponent by 1: The exponent is 1, and 1 - 1 = 0. This gives us 5x<sup>0</sup>.
-
Simplify: Remember that any number raised to the power of 0 (except 0<sup>0</sup> which is undefined) is equal to 1. Therefore, 5x<sup>0</sup> simplifies to 5 * 1 = 5.
Therefore, the derivative of 5x is 5.
What Does the Derivative of 5x Tell Us?
The derivative of 5x being 5 signifies that the function 5x has a constant slope of 5. This means that the graph of y = 5x is a straight line with a slope of 5. No matter where you are on the line, the instantaneous rate of change will always be 5.
This is a significant characteristic of linear functions. Linear functions exhibit a constant rate of change, unlike non-linear functions whose rate of change varies along their curves.
Visualizing the Derivative: The Tangent Line
To visualize this, imagine the graph of y = 5x. It's a straight line passing through the origin (0,0) with a slope of 5. If we draw a tangent line at any point on this line, the tangent line will coincide with the line itself. The slope of this tangent line, representing the instantaneous rate of change at that point, will always be 5 – the value of the derivative.
The Constant Multiple Rule: An Alternative Approach
Another helpful rule in differentiation is the constant multiple rule. This rule states that the derivative of a constant times a function is equal to the constant times the derivative of the function. In other words, if we have a function cf(x), where 'c' is a constant, then the derivative is c * f'(x).
Using this rule on 5x:
-
Identify the constant and the function: The constant is 5, and the function is x.
-
Find the derivative of the function: The derivative of x (which is x<sup>1</sup>) is 1 (using the power rule).
-
Multiply the constant by the derivative: 5 * 1 = 5.
Therefore, applying the constant multiple rule also confirms that the derivative of 5x is 5.
Higher-Order Derivatives: Exploring Beyond the First Derivative
While we've focused on the first derivative of 5x, it's important to know that we can find higher-order derivatives. The second derivative (denoted as f''(x) or d²y/dx²) represents the rate of change of the first derivative. In the case of 5x, the first derivative is 5, which is a constant. The derivative of a constant is always 0. Therefore:
- First derivative of 5x: 5
- Second derivative of 5x: 0
- Third derivative of 5x: 0
- and so on...
This means that the rate of change of the slope of the line y = 5x is 0, which is consistent with the fact that it's a straight line with a constant slope.
Applications of Derivatives: Real-World Examples
Understanding derivatives extends far beyond theoretical calculations. They have practical applications in numerous fields:
-
Physics: Derivatives are used extensively to describe velocity (the derivative of position) and acceleration (the derivative of velocity). Understanding these concepts is vital in mechanics, kinematics, and other branches of physics.
-
Economics: Derivatives are used in marginal analysis to determine the optimal production levels for maximizing profits or minimizing costs. They also help in analyzing rates of change in economic variables like supply and demand.
-
Engineering: Derivatives are crucial in designing optimal structures, analyzing stress and strain on materials, and modeling various engineering systems.
-
Machine Learning: Many machine learning algorithms rely on optimization techniques that use derivatives to find the minimum or maximum of a function. This is vital for training models and finding optimal parameters.
-
Computer Graphics: Derivatives are used in computer graphics for tasks like creating smooth curves and surfaces, simulating realistic lighting effects, and generating realistic textures.
Conclusion: Mastering the Fundamentals of Differentiation
The derivative of 5x, which is simply 5, may seem like a straightforward calculation. However, it illustrates fundamental concepts of calculus that are crucial for understanding more complex derivatives and their widespread applications. Mastering the basic differentiation rules, such as the power rule and constant multiple rule, lays a strong foundation for tackling more intricate mathematical problems in various fields. The simplicity of this example allows us to focus on the core concepts, providing a solid basis for further exploration of differential calculus. Understanding this seemingly simple derivative unlocks a world of possibilities in mathematics and its numerous applications in the real world.
Latest Posts
Latest Posts
-
What Is The Most Abundant Substance In The Human Body
May 12, 2025
-
What Are The Monomers Of Protein Polymers
May 12, 2025
-
List Of Verbs Ending In Ing
May 12, 2025
-
What Can 37 Be Divided By
May 12, 2025
-
What Is The Difference Between Alcoholic And Lactic Acid Fermentation
May 12, 2025
Related Post
Thank you for visiting our website which covers about What Is The Derivative Of 5x . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.