What Is Greater 1 2 Or 2 3
Juapaving
May 10, 2025 · 4 min read
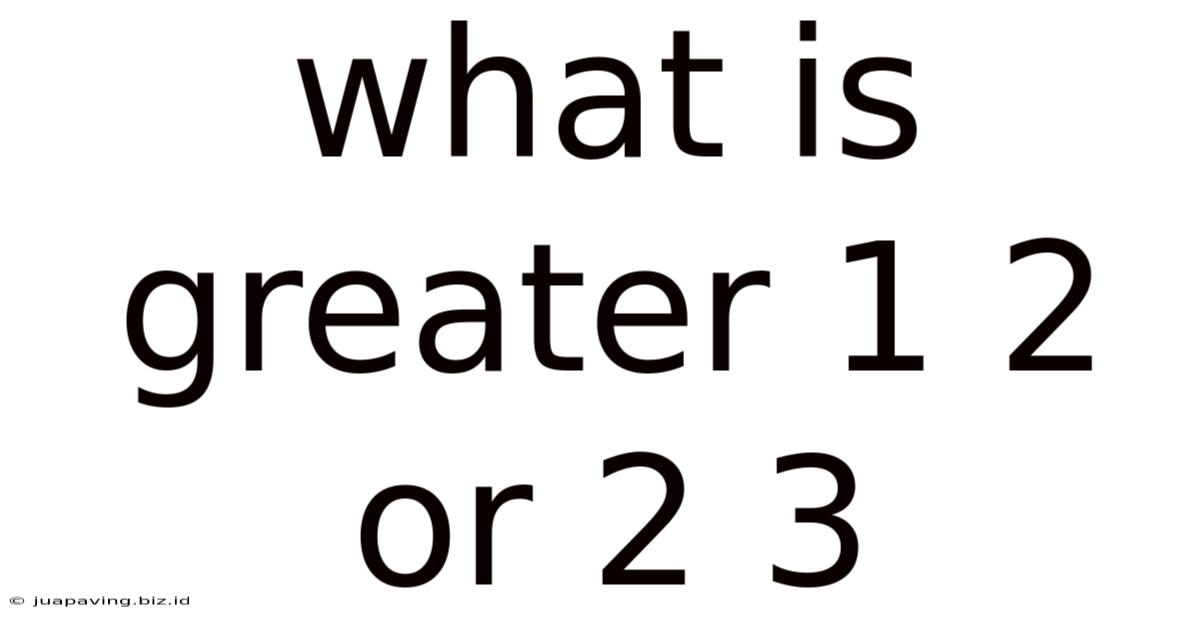
Table of Contents
What's Greater: 1/2 or 2/3? A Deep Dive into Fractions and Comparative Analysis
Determining which fraction is greater, 1/2 or 2/3, might seem trivial at first glance. However, a deeper exploration of this seemingly simple comparison provides a valuable opportunity to revisit fundamental mathematical concepts and sharpen our analytical skills. This article will not only answer the question directly but also explore various methods for comparing fractions, delving into the underlying principles and offering practical applications.
Understanding Fractions: A Quick Recap
Before jumping into the comparison, let's briefly refresh our understanding of fractions. A fraction represents a part of a whole. It consists of two numbers:
- Numerator: The top number, indicating the number of parts we have.
- Denominator: The bottom number, indicating the total number of equal parts the whole is divided into.
For example, in the fraction 1/2, the numerator (1) represents one part, and the denominator (2) signifies that the whole is divided into two equal parts.
Method 1: Finding a Common Denominator
This is arguably the most common and straightforward method for comparing fractions. The process involves finding a common denominator—a number that is a multiple of both denominators. Once we have a common denominator, we can directly compare the numerators.
Let's apply this to our fractions: 1/2 and 2/3.
-
Finding the Least Common Multiple (LCM): The LCM of 2 and 3 is 6. This will be our common denominator.
-
Converting the Fractions:
- 1/2 becomes 3/6 (multiply both numerator and denominator by 3)
- 2/3 becomes 4/6 (multiply both numerator and denominator by 2)
-
Comparing the Numerators: Now we have 3/6 and 4/6. Since 4 > 3, we conclude that 2/3 > 1/2.
Method 2: Visual Representation
Visual aids can be incredibly helpful in understanding fractions, especially when dealing with comparisons. We can represent these fractions using diagrams, such as circles or rectangles, divided into equal parts.
Imagine two circles:
- Circle 1: Divided into two equal parts, with one part shaded (representing 1/2).
- Circle 2: Divided into three equal parts, with two parts shaded (representing 2/3).
By visually inspecting the shaded areas, it's apparent that the shaded area in Circle 2 (2/3) is larger than the shaded area in Circle 1 (1/2). This confirms that 2/3 > 1/2.
Method 3: Converting to Decimals
Another effective method is to convert the fractions into decimal equivalents. This is particularly useful when comparing fractions with larger or more complex denominators.
- Converting 1/2 to a decimal: 1 ÷ 2 = 0.5
- Converting 2/3 to a decimal: 2 ÷ 3 ≈ 0.666... (a repeating decimal)
Since 0.666... > 0.5, we again conclude that 2/3 > 1/2.
Method 4: Cross-Multiplication
This method provides a quick and efficient way to compare fractions without finding a common denominator. It involves cross-multiplying the numerators and denominators:
- Multiply the numerator of the first fraction by the denominator of the second fraction: 1 x 3 = 3
- Multiply the numerator of the second fraction by the denominator of the first fraction: 2 x 2 = 4
Compare the results: 4 > 3. Since the result of the second multiplication is greater, the second fraction (2/3) is greater. Therefore, 2/3 > 1/2.
Beyond the Comparison: Real-World Applications
The ability to compare fractions is fundamental to various aspects of life, including:
- Cooking and Baking: Following recipes often requires understanding and comparing fractions of ingredients.
- Construction and Engineering: Precise measurements and calculations frequently involve fractions.
- Finance and Budgeting: Managing finances and understanding proportions necessitate fractional comprehension.
- Data Analysis: Interpreting data often involves working with percentages and proportions, which rely on fractional understanding.
Extending the Concept: Comparing More Than Two Fractions
The methods discussed above can be extended to compare more than two fractions. For example, consider comparing 1/2, 2/3, and 3/4. The most efficient approach would be to find the least common multiple of all the denominators (in this case, 12) and convert all fractions to have this common denominator before comparing numerators.
Addressing Common Misconceptions
A common misconception is that simply comparing numerators or denominators individually can determine which fraction is larger. This is incorrect. The relationship between the numerator and the denominator is crucial, and only through methods like finding a common denominator or cross-multiplication can we accurately determine the relative size of fractions.
Conclusion: Mastering Fraction Comparison
Comparing fractions, while seemingly basic, is a foundational skill with far-reaching applications. By understanding and employing different methods—finding a common denominator, visual representation, decimal conversion, and cross-multiplication—we can confidently and accurately compare fractions of any size or complexity. This skill is essential for success in various fields and contributes significantly to a stronger mathematical foundation. Mastering this skill empowers you to tackle more complex mathematical problems and confidently navigate various real-world scenarios involving proportions and ratios. Remember, the key is to choose the method most comfortable and efficient for you, but always double-check your work to ensure accuracy. The seemingly simple question of whether 1/2 or 2/3 is larger opens a door to a world of mathematical understanding and application.
Latest Posts
Latest Posts
-
The Central Dogma States That Information Flows From
May 10, 2025
-
Is Diamond A Element Compound Or Mixture
May 10, 2025
-
1 Billion Is Equal To How Many Crores
May 10, 2025
-
Is Rubber An Insulator Or A Conductor
May 10, 2025
-
An Ion With A Positive Charge Is Called
May 10, 2025
Related Post
Thank you for visiting our website which covers about What Is Greater 1 2 Or 2 3 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.