What Is Cube Root Of 27
Juapaving
May 13, 2025 · 5 min read
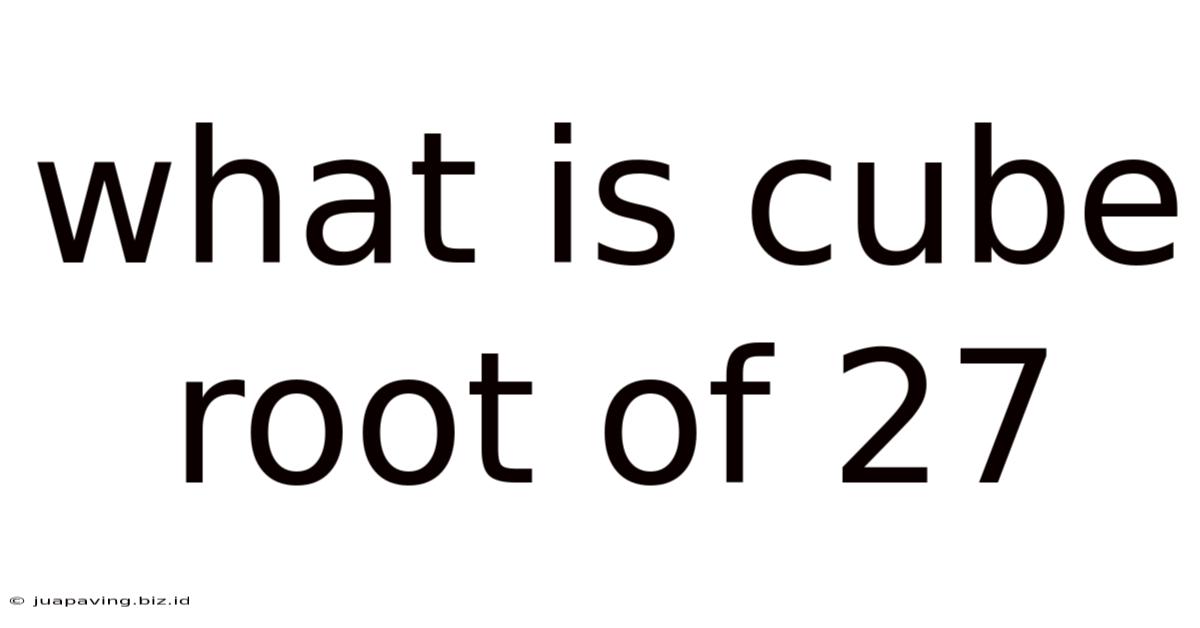
Table of Contents
What is the Cube Root of 27? A Deep Dive into Cube Roots and Their Applications
The seemingly simple question, "What is the cube root of 27?" opens a door to a fascinating world of mathematics, encompassing fundamental concepts, practical applications, and even surprising connections to other areas of study. This article will not only answer this specific question but also explore the broader topic of cube roots, delving into their properties, calculations, and relevance in various fields.
Understanding Cube Roots: The Basics
Before we tackle the cube root of 27, let's establish a solid understanding of what a cube root actually is. In mathematics, the cube root of a number is a value that, when multiplied by itself three times (cubed), equals the original number. This is represented symbolically as ³√x, where 'x' is the number for which we're finding the cube root.
For example, the cube root of 8 is 2 because 2 x 2 x 2 = 8. Similarly, the cube root of 64 is 4 because 4 x 4 x 4 = 64.
Key Concepts:
- Cubing a number: This is the process of multiplying a number by itself three times. For instance, cubing 3 is 3 x 3 x 3 = 27.
- Inverse operation: The cube root is the inverse operation of cubing a number. Just as addition and subtraction are inverse operations, and multiplication and division are inverse operations, cubing and finding the cube root are inverse operations.
- Principal cube root: Every real number has only one real cube root. This is known as the principal cube root. However, in the realm of complex numbers, a number has three cube roots. We'll focus primarily on real cube roots in this article.
Calculating the Cube Root of 27
Now, let's address the original question: What is the cube root of 27? The answer is 3. This is because 3 x 3 x 3 = 27.
While this specific calculation might seem straightforward, understanding how to find cube roots of other numbers is crucial. There are several methods to achieve this:
1. Memorization:
For commonly used numbers like 8, 27, 64, and 125, memorizing their cube roots can significantly speed up calculations. This is a particularly useful technique for quick problem-solving in various contexts.
2. Prime Factorization:
This method is effective for numbers that can be easily broken down into prime factors. Let's consider the number 216. We can express 216 as 2 x 2 x 2 x 3 x 3 x 3. Grouping the factors into sets of three, we get (2 x 2 x 2) x (3 x 3 x 3) = 8 x 27. Therefore, the cube root of 216 is the cube root of 8 multiplied by the cube root of 27, which equals 2 x 3 = 6.
3. Estimation and Trial and Error:
For numbers without easily identifiable prime factors, estimation and trial and error can be useful. This involves making educated guesses and refining them based on the results obtained by cubing the guesses. This method becomes more efficient with practice.
4. Calculators and Software:
Modern calculators and mathematical software packages have built-in functions to calculate cube roots directly. This method is extremely convenient, especially when dealing with larger numbers or more complex calculations.
Applications of Cube Roots in Various Fields
Cube roots are not merely abstract mathematical concepts; they have numerous practical applications across various fields:
1. Geometry and Volume Calculations:
Cube roots are fundamental to calculating the side length of a cube given its volume. If the volume of a cube is known, its side length can be found by taking the cube root of the volume. This has applications in numerous fields, including engineering, architecture, and construction.
2. Physics and Engineering:
Cube roots appear in various physics and engineering equations, particularly those dealing with volume, capacity, and scaling problems. For example, they are crucial in calculating the radius of a sphere given its volume.
3. Chemistry and Molecular Structures:
In chemistry, understanding the volume and arrangement of atoms and molecules often involves cube roots. Analyzing crystal structures and calculating unit cell dimensions often relies on this mathematical concept.
4. Finance and Investments:
Compound interest calculations sometimes involve cube roots. In scenarios dealing with the time it takes for investments to triple in value, the cube root is needed to solve the equation and find the number of compounding periods.
5. Statistics and Data Analysis:
Cube roots are applied in various statistical calculations and data analysis techniques, often used to normalize data or transform variables. While not as frequently used as square roots, they have a significant role in specialized statistical methods.
6. Computer Graphics and 3D Modeling:
In computer graphics and 3D modeling, cube roots play a role in creating and manipulating three-dimensional objects. They are used in transformations, scaling, and algorithms that determine relationships in three-dimensional space.
Beyond the Real Numbers: Complex Cube Roots
As mentioned earlier, in the realm of complex numbers, every number (excluding zero) has three cube roots. Understanding complex cube roots requires a familiarity with complex numbers and their representation in polar form. The three cube roots are equally spaced around a circle in the complex plane, centered at the origin. This fascinating aspect extends the concept of cube roots beyond the familiar real number system.
Solving Problems Involving Cube Roots
Let's consider a practical example:
Problem: A cubic water tank has a volume of 1728 cubic meters. What is the length of one side of the tank?
Solution: Since the tank is a cube, all its sides are equal in length. The volume of a cube is calculated as side x side x side (side³). Therefore, to find the side length, we need to take the cube root of the volume:
³√1728 = 12 meters
The length of one side of the tank is 12 meters.
Conclusion
The cube root of 27, while a seemingly simple concept, serves as a gateway to a rich understanding of mathematics, particularly the concept of roots and their applications. From fundamental geometry problems to advanced scientific calculations, the cube root and the broader concept of nth roots play an essential role in our understanding and modeling of the world around us. Understanding how to calculate cube roots and appreciate their various applications is a valuable skill across various disciplines. The ability to perform these calculations accurately is fundamental in many fields, and mastering this concept opens doors to more complex mathematical and scientific endeavors.
Latest Posts
Latest Posts
-
Words That End With I N G
May 14, 2025
-
How Many Legs Does And Ant Have
May 14, 2025
-
What Is A Group Of Fish Called
May 14, 2025
-
Do Ionic Compounds Dissolve In Water
May 14, 2025
-
5 Letter Words That Start With Ak
May 14, 2025
Related Post
Thank you for visiting our website which covers about What Is Cube Root Of 27 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.