What Is All The Factors Of 49
Juapaving
Mar 28, 2025 · 5 min read
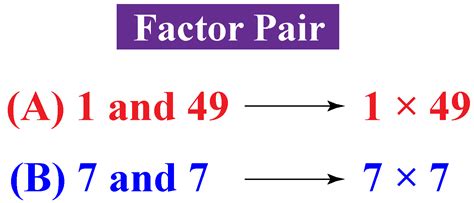
Table of Contents
What are All the Factors of 49? A Deep Dive into Number Theory
The seemingly simple question, "What are all the factors of 49?" opens a door to a fascinating world of number theory. While the immediate answer might seem obvious, exploring this question allows us to delve into concepts like prime factorization, divisibility rules, and the broader implications of factors in mathematics and beyond. This comprehensive guide will not only answer the question but also provide a solid foundation in understanding factors and their significance.
Understanding Factors and Divisibility
Before we tackle the factors of 49, let's establish a clear definition. A factor (also known as a divisor) of a number is any integer that divides that number evenly, leaving no remainder. In other words, if 'a' is a factor of 'b', then b/a results in a whole number.
Divisibility is the key concept here. We can determine if a number is a factor using various methods, including:
- Direct division: The simplest method; simply divide the number by the potential factor. If the result is a whole number, it's a factor.
- Prime factorization: Breaking down the number into its prime components provides a complete list of factors. This is especially useful for larger numbers.
- Divisibility rules: Rules exist for specific numbers (like 2, 3, 5, etc.) to quickly check for divisibility without performing division.
Finding the Factors of 49: A Step-by-Step Approach
Now, let's systematically find all the factors of 49.
Method 1: Direct Division
We'll start by testing integers starting from 1:
- 1 divides 49 evenly (49/1 = 49).
- 2 does not divide 49 evenly.
- 3 does not divide 49 evenly.
- 4 does not divide 49 evenly.
- 5 does not divide 49 evenly.
- 6 does not divide 49 evenly.
- 7 divides 49 evenly (49/7 = 7).
- 8 does not divide 49 evenly.
Notice something? Once we reach 7, we've found a factor pair (7 and 7). Any subsequent number we test will already have been covered in the reverse pair. This leads us to the conclusion that we only need to test numbers up to the square root of the given number.
Method 2: Prime Factorization
49 can be expressed as 7 x 7. Since 7 is a prime number (a number divisible only by 1 and itself), the prime factorization of 49 is 7².
This immediately tells us the factors: 1, 7, and 49.
Method 3: Listing Factor Pairs
We can systematically list the factor pairs:
- 1 x 49
- 7 x 7
This approach highlights that the factors of 49 are 1, 7, and 49.
The Factors of 49: A Complete List
Therefore, the complete list of factors for 49 is: 1, 7, and 49.
Beyond 49: Expanding our Understanding of Factors
The seemingly simple case of 49 provides a solid foundation for understanding factors. Let's explore some broader concepts related to factors:
1. Prime Numbers and Factorization
Prime numbers are building blocks of all other numbers. They are numbers greater than 1 that are only divisible by 1 and themselves (e.g., 2, 3, 5, 7, 11, etc.). Prime factorization is the process of expressing a number as a product of its prime factors. The Fundamental Theorem of Arithmetic states that every integer greater than 1 can be expressed uniquely as a product of prime numbers. This theorem is fundamental to number theory and is crucial in various areas of mathematics.
2. The Number of Factors
The number of factors a number has depends on its prime factorization. For instance, if the prime factorization of a number is p<sup>a</sup> * q<sup>b</sup> (where p and q are prime numbers), the total number of factors is (a+1)(b+1). For 49 (7²), the number of factors is (2+1) = 3.
3. Factors and Divisibility Rules
Divisibility rules provide shortcuts for determining if a number is divisible by certain integers without performing long division. These rules are particularly helpful when dealing with larger numbers. For example:
- Divisibility by 2: A number is divisible by 2 if its last digit is even (0, 2, 4, 6, 8).
- Divisibility by 3: A number is divisible by 3 if the sum of its digits is divisible by 3.
- Divisibility by 5: A number is divisible by 5 if its last digit is 0 or 5.
- Divisibility by 7: The divisibility rule for 7 is more complex. It involves repeatedly subtracting twice the last digit from the remaining digits. If the result is divisible by 7, then the original number is as well.
4. Factors in Real-World Applications
Factors have practical applications in various fields:
- Computer Science: Factorization is crucial in cryptography, especially in RSA encryption, which relies on the difficulty of factoring large numbers.
- Engineering: Factors are used in calculating dimensions, ratios, and proportions in engineering designs.
- Music Theory: Musical intervals and chord progressions often involve ratios and relationships related to factors.
- Scheduling and Logistics: Optimal scheduling and resource allocation frequently involve problems related to factor analysis and divisibility.
Conclusion: The Significance of Understanding Factors
While finding the factors of 49 might seem trivial, this exploration provides a gateway to a deeper understanding of number theory and its broader implications. By mastering concepts like prime factorization, divisibility rules, and the relationship between factors and prime numbers, we gain valuable tools for solving more complex mathematical problems and appreciating the underlying structure of numbers. The simplicity of this particular problem allows for a focused examination of these concepts, solidifying our understanding of these fundamental building blocks of mathematics. This deeper understanding then extends to more advanced topics and real-world applications, highlighting the importance of understanding the seemingly simple concepts within the mathematical world.
Latest Posts
Latest Posts
-
How Many Cm Is 36 Inches
Mar 31, 2025
-
Is Oxygen A Metal Or Nonmetal
Mar 31, 2025
-
72 As Product Of Prime Factors
Mar 31, 2025
-
How Many Valence Electrons Does S2 Have
Mar 31, 2025
-
What Is 17 25 As A Decimal
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about What Is All The Factors Of 49 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
