What Is 3 25 As A Percent
Juapaving
May 10, 2025 · 4 min read
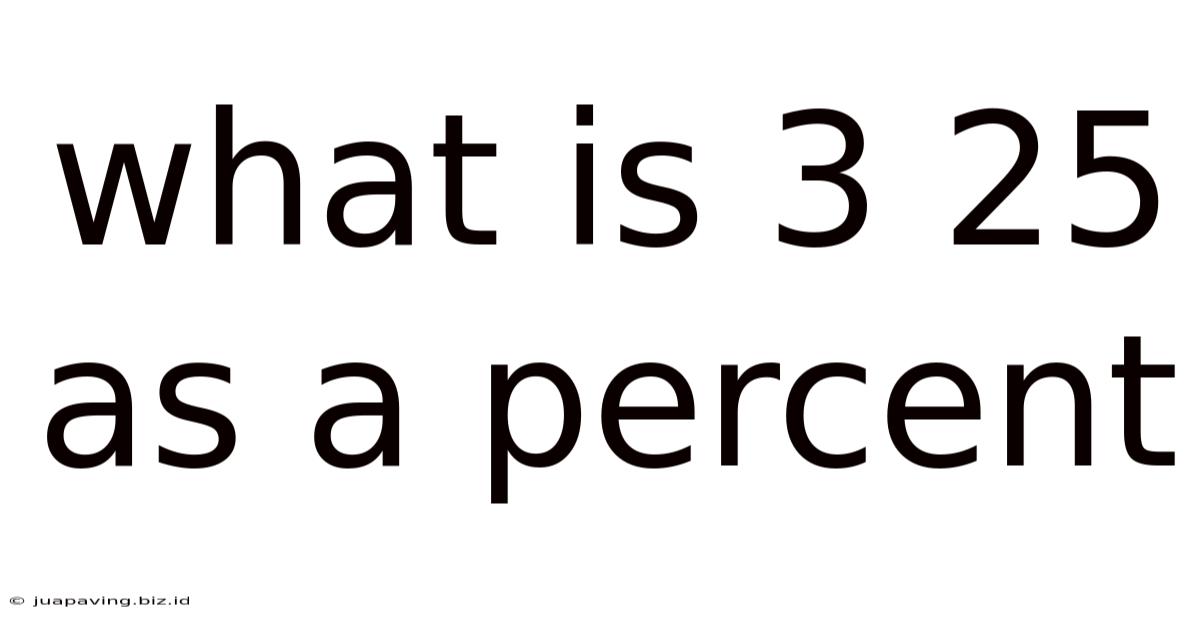
Table of Contents
What is 3/25 as a Percent? A Comprehensive Guide to Fraction-to-Percentage Conversions
Converting fractions to percentages is a fundamental skill in mathematics with wide-ranging applications in various fields, from finance and statistics to everyday calculations. Understanding this process empowers you to easily interpret data, compare proportions, and solve problems involving ratios. This article will comprehensively explain how to convert the fraction 3/25 into a percentage, and delve deeper into the underlying concepts and methods involved in fraction-to-percentage conversions. We'll also explore different approaches and provide practical examples to solidify your understanding.
Understanding Fractions and Percentages
Before diving into the conversion, let's briefly revisit the concepts of fractions and percentages.
-
Fractions: A fraction represents a part of a whole. It's expressed as a ratio of two numbers: the numerator (top number) and the denominator (bottom number). The numerator indicates the number of parts you have, while the denominator indicates the total number of parts in the whole. For example, in the fraction 3/25, 3 is the numerator and 25 is the denominator.
-
Percentages: A percentage represents a fraction of 100. It indicates how many parts out of 100 represent a given proportion. The symbol "%" is used to denote percentages. For instance, 50% means 50 parts out of 100, which is equivalent to the fraction 50/100 or 1/2.
Converting 3/25 to a Percentage: The Direct Method
The most straightforward way to convert a fraction to a percentage is to first convert the fraction to a decimal and then multiply by 100.
Step 1: Convert the fraction to a decimal.
To convert 3/25 to a decimal, we divide the numerator (3) by the denominator (25):
3 ÷ 25 = 0.12
Step 2: Multiply the decimal by 100 to express it as a percentage.
0.12 × 100 = 12%
Therefore, 3/25 is equal to 12%.
Alternative Methods for Fraction-to-Percentage Conversion
While the direct method is efficient, understanding alternative methods broadens your mathematical toolkit and helps in solving various percentage problems.
Method 1: Finding an Equivalent Fraction with a Denominator of 100
This method leverages the fact that percentages are always expressed out of 100. If we can find an equivalent fraction of 3/25 with a denominator of 100, the numerator directly represents the percentage.
To achieve this, we need to determine what number, when multiplied by 25, gives 100. This number is 4 (25 x 4 = 100). Since we multiply the denominator by 4, we must also multiply the numerator by 4 to maintain the fraction's value:
(3 x 4) / (25 x 4) = 12/100
Since 12/100 means 12 out of 100, the percentage is 12%.
Method 2: Using Proportions
Proportions provide a powerful way to solve percentage problems. We can set up a proportion to solve for the percentage:
3/25 = x/100
To solve for 'x' (the percentage), we cross-multiply:
25x = 300
x = 300/25
x = 12
Therefore, x = 12%, confirming our previous results.
Practical Applications and Real-World Examples
Understanding fraction-to-percentage conversions is crucial in various real-world scenarios:
-
Finance: Calculating interest rates, discounts, and profit margins often involves converting fractions to percentages. For example, a 3/25 discount on a product translates to a 12% discount.
-
Statistics: Representing data in percentage form helps in easy interpretation and comparison. If 3 out of 25 students passed an exam, this represents a 12% pass rate.
-
Everyday Life: Understanding percentages is essential for calculating tips, sales tax, and understanding proportions in recipes or measurements.
Advanced Concepts and Further Exploration
While the conversion of 3/25 to a percentage is relatively straightforward, expanding your knowledge of percentage calculations opens doors to more complex problems.
-
Calculating Percentages of Quantities: Once you know the percentage, you can apply it to calculate a percentage of a larger quantity. For example, finding 12% of $500 involves multiplying 0.12 by 500, resulting in $60.
-
Working Backwards from Percentages: You can also work backward from a percentage to find the original fraction or quantity. For example, if you know that 12% of a number is 60, you can set up an equation to solve for the original number.
-
Compound Percentages: Understanding compound percentages is important for calculations involving repeated percentage increases or decreases, such as compound interest calculations.
-
Percentage Change: Calculating percentage change is vital for analyzing trends and growth rates. This involves finding the difference between two values and expressing this difference as a percentage of the original value.
Conclusion: Mastering Percentage Conversions
Converting fractions to percentages is a fundamental skill with numerous applications in various fields. The direct method, along with alternative methods like finding equivalent fractions or using proportions, provide different approaches to achieve the conversion. Understanding these methods not only allows you to solve simple problems like converting 3/25 to 12% but also lays a solid foundation for tackling more complex percentage calculations in real-world situations. By mastering this skill, you significantly enhance your mathematical proficiency and ability to interpret and analyze data effectively. Remember to practice regularly to solidify your understanding and build confidence in handling percentage problems. Further exploration of advanced percentage concepts will further refine your mathematical skills and provide you with a comprehensive understanding of this essential area of mathematics.
Latest Posts
Latest Posts
-
How Do You Find The Degree Of A Monomial
May 10, 2025
-
What Is The Shape Of The Dna Molecule Called
May 10, 2025
-
Which Of These Is Not Considered Connective Tissue
May 10, 2025
-
13 Rounded To The Nearest Tenth
May 10, 2025
-
What Are The Two Kinds Of Friction
May 10, 2025
Related Post
Thank you for visiting our website which covers about What Is 3 25 As A Percent . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.