What Is 20 Percent In A Fraction
Juapaving
May 11, 2025 · 5 min read
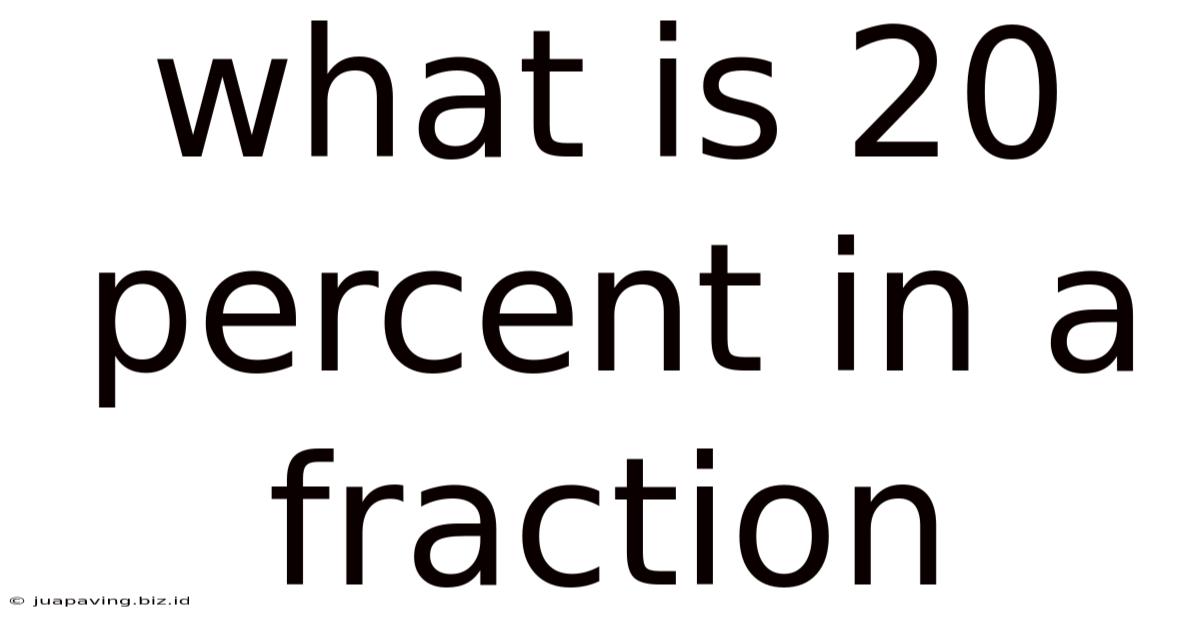
Table of Contents
What is 20 Percent as a Fraction? A Comprehensive Guide
Understanding percentages, fractions, and decimals is fundamental to many areas of life, from calculating discounts in a store to understanding financial reports. This comprehensive guide will delve deep into the conversion of percentages to fractions, specifically focusing on how to express 20 percent as a fraction and exploring the broader concepts involved.
Understanding Percentages
A percentage is a way of expressing a number as a fraction of 100. The word "percent" itself comes from the Latin "per centum," meaning "out of a hundred." Therefore, 20 percent means 20 out of 100. This understanding forms the basis for converting percentages to fractions.
Key Concepts:
- Numerator: The top number in a fraction, representing the part.
- Denominator: The bottom number in a fraction, representing the whole.
- Percent Sign (%): The symbol used to denote a percentage.
Converting 20 Percent to a Fraction: Step-by-Step
The conversion process is straightforward:
-
Write the percentage as a fraction with 100 as the denominator: 20% becomes 20/100.
-
Simplify the fraction: This involves finding the greatest common divisor (GCD) of the numerator and denominator and dividing both by it. The GCD of 20 and 100 is 20.
-
Divide both the numerator and the denominator by the GCD: 20 ÷ 20 = 1 and 100 ÷ 20 = 5.
-
The simplified fraction: This gives us the final answer: 1/5.
Therefore, 20 percent as a fraction is 1/5.
Understanding Fraction Simplification
Simplifying fractions is crucial for representing them in their most concise form. A simplified fraction is one where the numerator and denominator have no common factors other than 1. This makes the fraction easier to understand and work with in calculations.
Methods for Simplifying Fractions:
-
Finding the Greatest Common Divisor (GCD): The GCD is the largest number that divides both the numerator and denominator without leaving a remainder. You can find the GCD using various methods, including prime factorization or the Euclidean algorithm.
-
Dividing by Common Factors: If you can spot a common factor between the numerator and denominator, you can divide both by that factor repeatedly until no further simplification is possible.
Example: Let's simplify the fraction 36/48:
-
Find the GCD: The GCD of 36 and 48 is 12.
-
Divide: 36 ÷ 12 = 3 and 48 ÷ 12 = 4.
-
Simplified fraction: 3/4.
Other Percentage to Fraction Conversions
Understanding the process for 20% allows you to easily convert other percentages to fractions. Here are a few examples:
- 50%: 50/100 simplifies to 1/2.
- 75%: 75/100 simplifies to 3/4.
- 10%: 10/100 simplifies to 1/10.
- 25%: 25/100 simplifies to 1/4.
- 1%: 1/100 (This fraction is already simplified).
Practical Applications of Percentage to Fraction Conversions
The ability to convert percentages to fractions is invaluable in many real-world scenarios:
-
Calculating Discounts: If a store offers a 20% discount, you can easily calculate the discount amount by multiplying the original price by 1/5 (the fraction equivalent of 20%).
-
Understanding Financial Statements: Financial reports often use percentages to represent various ratios and proportions. Converting these percentages to fractions can help you understand the underlying relationships more clearly.
-
Solving Mathematical Problems: Many mathematical problems involving percentages are easier to solve if you convert the percentages to fractions first.
-
Cooking and Baking: Recipes often use percentages to indicate the proportion of ingredients. Understanding fraction equivalents allows for easier scaling of recipes.
-
Data Analysis: In data analysis, percentages are frequently used to represent proportions of a dataset. Converting to fractions can simplify further calculations and comparisons.
Working with Improper Fractions
Sometimes, when converting a percentage greater than 100%, you'll end up with an improper fraction – a fraction where the numerator is larger than the denominator. For example, 150% becomes 150/100, which simplifies to 3/2.
Improper fractions can be converted to mixed numbers (a whole number and a proper fraction). In the case of 3/2, this would be 1 ½.
Converting Decimals to Fractions and Percentages
The relationship between percentages, fractions, and decimals is interconnected. You can easily convert between these forms:
-
Decimal to Fraction: Write the decimal as a fraction with a denominator of a power of 10 (10, 100, 1000, etc.), then simplify. For example, 0.25 is 25/100, which simplifies to 1/4.
-
Decimal to Percentage: Multiply the decimal by 100 and add the percent sign. For example, 0.25 becomes 25%.
-
Fraction to Decimal: Divide the numerator by the denominator. For example, 1/4 is 0.25.
-
Fraction to Percentage: Convert the fraction to a decimal, then multiply by 100 and add the percent sign.
Advanced Applications and Further Exploration
The principles discussed here form a foundation for more advanced mathematical concepts:
-
Ratio and Proportion: Percentages and fractions are closely related to ratios and proportions. Understanding these relationships is crucial in many scientific and engineering applications.
-
Algebra: Many algebraic equations involve percentages and fractions. The ability to convert between these forms is essential for solving these equations.
-
Statistics and Probability: Percentages and fractions are fundamental to statistics and probability, used to represent probabilities, frequencies, and proportions within datasets.
-
Finance: Interest rates, discounts, and investment returns are all expressed using percentages. A solid grasp of percentage to fraction conversion is necessary for understanding financial concepts.
Conclusion: Mastering Percentage to Fraction Conversions
Converting percentages to fractions is a fundamental skill with wide-ranging applications. By understanding the core concepts, the step-by-step process, and the various methods of simplification, you can confidently handle percentage calculations in numerous contexts. This ability enhances your problem-solving skills and provides a deeper understanding of numerical relationships. Remember to practice regularly to solidify your understanding and build your proficiency. With consistent effort, you'll become adept at converting percentages to fractions and applying this knowledge to real-world situations.
Latest Posts
Latest Posts
-
These Are Organelles That Digest Macromolecules
May 11, 2025
-
A Bundle Of Muscle Fibers Is Known As
May 11, 2025
-
The Development Of A New Species Is Called
May 11, 2025
-
How Many Atoms Are In Nitrogen
May 11, 2025
-
Surface Area Of A Hexagonal Pyramid
May 11, 2025
Related Post
Thank you for visiting our website which covers about What Is 20 Percent In A Fraction . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.