What Is 15/25 As A Percent
Juapaving
May 12, 2025 · 4 min read
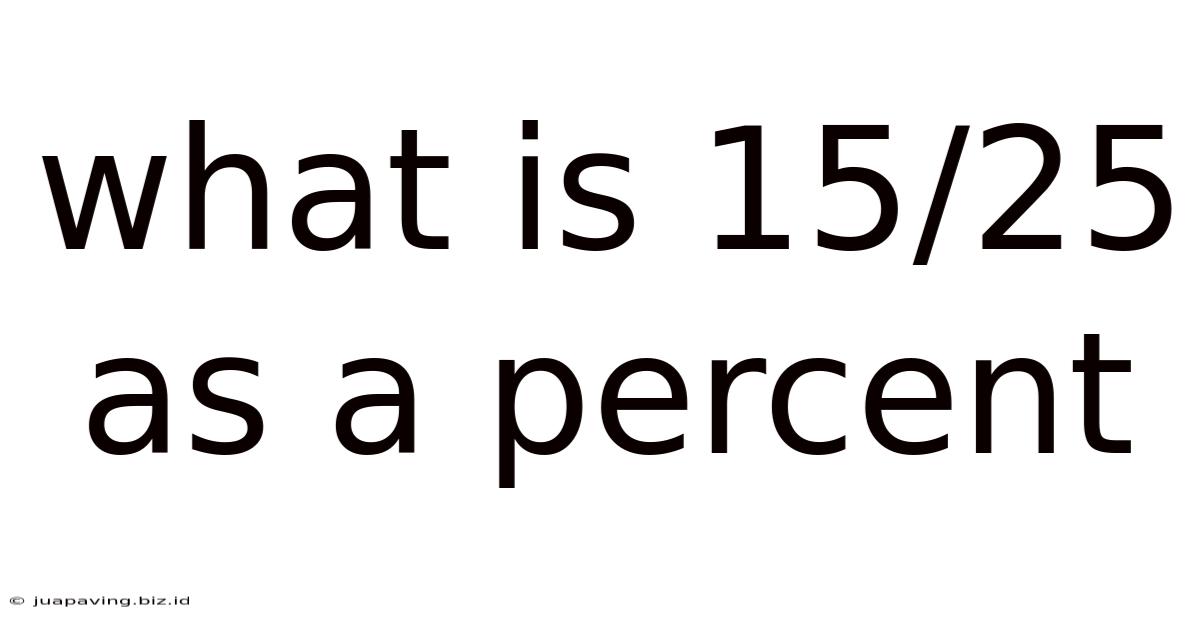
Table of Contents
What is 15/25 as a Percent? A Comprehensive Guide
Converting fractions to percentages is a fundamental skill in mathematics with widespread applications in everyday life, from calculating discounts in shopping to understanding statistics in news reports. This comprehensive guide will not only answer the question "What is 15/25 as a percent?" but also delve into the underlying concepts, provide multiple methods for solving similar problems, and explore real-world examples.
Understanding Fractions and Percentages
Before tackling the conversion, let's clarify the basics. A fraction represents a part of a whole. It consists of a numerator (the top number) and a denominator (the bottom number). The numerator indicates how many parts we have, and the denominator indicates how many equal parts make up the whole.
A percentage, denoted by the symbol %, represents a fraction out of 100. It's a way to express a proportion or a ratio as a part of a hundred. For example, 50% means 50 out of 100, which is equivalent to the fraction 50/100 or the decimal 0.5.
Method 1: Simplifying the Fraction
The simplest approach to converting 15/25 to a percentage involves simplifying the fraction first. We look for the greatest common divisor (GCD) of the numerator (15) and the denominator (25). The GCD of 15 and 25 is 5.
Dividing both the numerator and the denominator by 5, we get:
15 ÷ 5 = 3 25 ÷ 5 = 5
Therefore, 15/25 simplifies to 3/5.
Now, to convert 3/5 to a percentage, we need to express it as a fraction with a denominator of 100. We can achieve this by multiplying both the numerator and the denominator by 20:
(3 × 20) / (5 × 20) = 60/100
60/100 represents 60 out of 100, which is equivalent to 60%.
Therefore, 15/25 as a percent is 60%.
Method 2: Direct Conversion using Division
Another method involves directly converting the fraction to a decimal and then multiplying by 100.
-
Divide the numerator by the denominator: 15 ÷ 25 = 0.6
-
Multiply the decimal by 100: 0.6 × 100 = 60
Therefore, 15/25 is equal to 60%.
This method is particularly useful when dealing with fractions that are not easily simplified.
Method 3: Using Proportions
We can also use proportions to solve this problem. We set up a proportion where x represents the percentage we want to find:
15/25 = x/100
To solve for x, we can cross-multiply:
15 × 100 = 25 × x 1500 = 25x x = 1500 ÷ 25 x = 60
Therefore, 15/25 is equal to 60%.
Real-World Applications of Percentage Conversions
The ability to convert fractions to percentages is crucial in various real-world scenarios:
1. Retail Discounts:
Imagine a store offers a 25% discount on an item originally priced at $100. To calculate the discount amount, you would convert 25% to a fraction (25/100 or 1/4) and multiply it by the original price: ($100) x (1/4) = $25. The discounted price would then be $100 - $25 = $75. Understanding percentage conversions is key to easily calculating the final price after discounts.
2. Calculating Grades:
Students often encounter fractions when calculating their grades. For example, if a student answers 15 out of 25 questions correctly on a test, their score is 15/25, which we've already established is 60%. This allows for easy comparison of performance across different assessments.
3. Financial Calculations:
In finance, percentages are used extensively. Calculating interest rates, profit margins, tax rates, and investment returns all rely on converting fractions or decimals to percentages for easier comprehension and comparison. Understanding these conversions is critical for making informed financial decisions.
4. Data Analysis and Statistics:
Data analysis often involves working with proportions and ratios, frequently expressed as percentages. For example, analyzing survey results, market research data, or scientific experiments often requires converting fractions representing proportions into percentages to make the data more understandable and visually appealing in charts and graphs.
5. Everyday Life:
Beyond specific fields, understanding percentages is valuable in everyday life. Tips at restaurants, calculating sales tax, determining the nutritional content of food, and interpreting weather forecasts all involve working with percentages.
Beyond 15/25: Mastering Fraction-to-Percentage Conversions
The methods described above can be applied to any fraction. Remember these key steps:
-
Simplify the fraction: Reduce the fraction to its simplest form by finding the greatest common divisor (GCD) of the numerator and denominator and dividing both by it.
-
Convert to a decimal: Divide the numerator by the denominator.
-
Multiply by 100: Multiply the resulting decimal by 100 to express it as a percentage.
-
Add the percent symbol: Add the "%" symbol to indicate that the result is a percentage.
By mastering these steps, you can confidently convert any fraction into a percentage, making it easier to understand and use this crucial mathematical concept in your daily life and professional endeavors. This skill will enhance your problem-solving abilities and enable you to interpret information more effectively across various fields. Practice consistently, and you’ll soon find that converting fractions to percentages becomes second nature.
Latest Posts
Latest Posts
-
How Many Times We Breathe In A Day
May 14, 2025
-
Five Letter Words Ending In E N
May 14, 2025
-
What Is The Square Root Of 40
May 14, 2025
-
How Many Inches Is 17 Cm
May 14, 2025
-
How Many Players In Cricket Team
May 14, 2025
Related Post
Thank you for visiting our website which covers about What Is 15/25 As A Percent . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.