What Fraction Is Equivalent To 1 2
Juapaving
May 12, 2025 · 5 min read
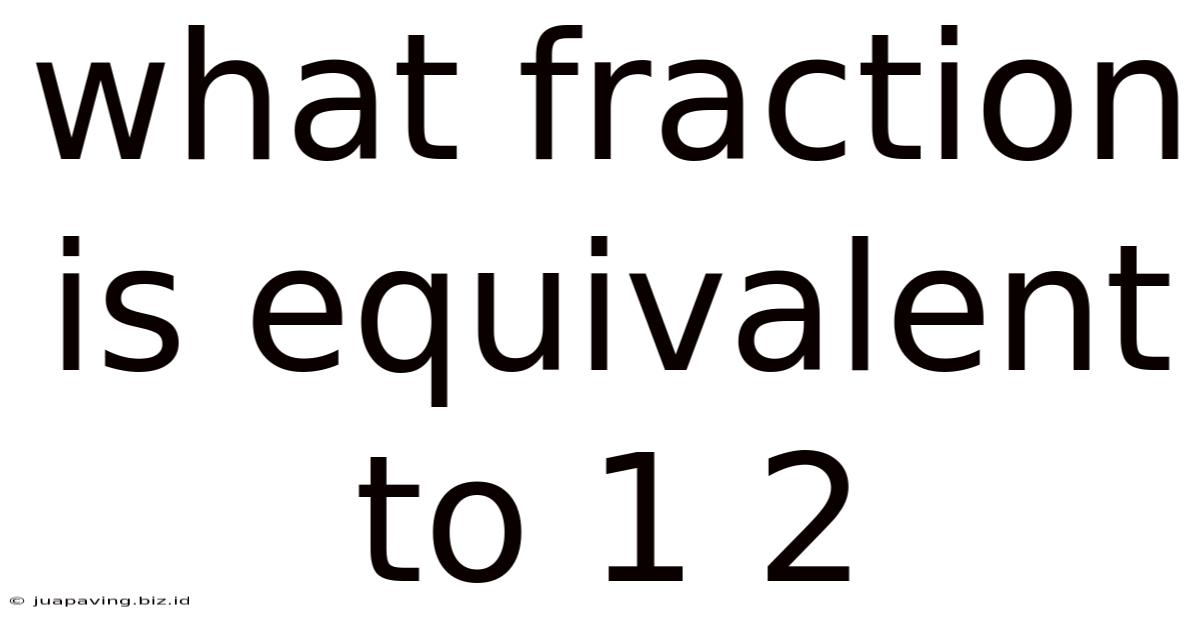
Table of Contents
What Fraction is Equivalent to 1 ½? Understanding Equivalent Fractions and Mixed Numbers
The question, "What fraction is equivalent to 1 ½?" might seem simple at first glance, but it opens the door to a deeper understanding of fractions, mixed numbers, and the crucial concept of equivalent fractions. This comprehensive guide will not only answer this question but also equip you with the knowledge to confidently tackle similar problems and build a strong foundation in fractional arithmetic.
Understanding Fractions and Mixed Numbers
Before diving into the equivalence, let's review the basics. A fraction represents a part of a whole. It consists of two parts:
- Numerator: The top number, indicating the number of parts you have.
- Denominator: The bottom number, indicating the total number of equal parts the whole is divided into.
A mixed number combines a whole number and a proper fraction (a fraction where the numerator is smaller than the denominator). For example, 1 ½ is a mixed number: one whole and one-half.
Converting Mixed Numbers to Improper Fractions
To find an equivalent fraction to 1 ½, we first need to convert the mixed number into an improper fraction. An improper fraction has a numerator that is greater than or equal to its denominator. Here's how to convert 1 ½:
- Multiply the whole number by the denominator: 1 x 2 = 2
- Add the numerator: 2 + 1 = 3
- Keep the same denominator: The denominator remains 2.
Therefore, 1 ½ is equivalent to the improper fraction 3/2.
Equivalent Fractions: The Core Concept
Equivalent fractions represent the same value, even though they look different. They are created by multiplying or dividing both the numerator and denominator by the same non-zero number. Think of it like slicing a pizza: ½ a pizza is the same as 2/4, 3/6, 4/8, and so on. Each fraction represents the same amount of pizza.
Finding More Equivalent Fractions to 1 ½ (or 3/2)
Now that we have the improper fraction 3/2, we can easily find more equivalent fractions by multiplying both the numerator and the denominator by the same number. For example:
- Multiplying by 2: (3 x 2) / (2 x 2) = 6/4
- Multiplying by 3: (3 x 3) / (2 x 3) = 9/6
- Multiplying by 4: (3 x 4) / (2 x 4) = 12/8
- Multiplying by 5: (3 x 5) / (2 x 5) = 15/10
And so on. All these fractions – 6/4, 9/6, 12/8, 15/10, and infinitely many more – are equivalent to 1 ½ and 3/2.
Simplifying Fractions: Finding the Simplest Form
While there are infinitely many equivalent fractions, it's often helpful to find the simplest form of a fraction. This is also known as reducing a fraction to its lowest terms. A fraction is in its simplest form when the greatest common divisor (GCD) of the numerator and the denominator is 1.
In the case of 3/2, the GCD of 3 and 2 is 1, meaning it's already in its simplest form. However, if we consider one of the equivalent fractions, such as 6/4, we can simplify it:
- Find the GCD of the numerator and denominator: The GCD of 6 and 4 is 2.
- Divide both the numerator and denominator by the GCD: 6 ÷ 2 = 3 and 4 ÷ 2 = 2.
This simplifies 6/4 back to 3/2, confirming that it is indeed an equivalent fraction.
Applications of Equivalent Fractions
Understanding equivalent fractions is crucial in various mathematical contexts:
- Adding and Subtracting Fractions: You need to find common denominators before adding or subtracting fractions. This often involves finding equivalent fractions.
- Comparing Fractions: Determining which fraction is larger or smaller requires finding equivalent fractions with a common denominator.
- Solving Equations: Equivalent fractions play a role in simplifying equations and finding solutions.
- Real-world Applications: Equivalent fractions are used extensively in everyday life, from cooking and baking (measuring ingredients) to construction and engineering (calculating proportions).
Beyond the Basics: Visualizing Equivalent Fractions
One of the most effective ways to grasp the concept of equivalent fractions is through visualization. Imagine a rectangle representing a whole.
- 1/2: Divide the rectangle into two equal parts and shade one.
- 2/4: Divide the same rectangle into four equal parts and shade two.
- 3/6: Divide the rectangle into six equal parts and shade three.
Visually, you can see that the shaded areas in each case represent the same amount, even though the fractions look different. This visual representation solidifies the understanding of equivalence.
Practical Examples and Problem-Solving
Let's explore some practical examples to reinforce our understanding:
Example 1: A recipe calls for 1 ½ cups of flour. Express this amount as an improper fraction.
Solution: Following the conversion steps, 1 ½ cups is equivalent to 3/2 cups.
Example 2: John ate 2/8 of a pizza, and Mary ate 3/12 of the same pizza. Did they eat the same amount?
Solution: Simplify both fractions. 2/8 simplifies to 1/4, and 3/12 simplifies to 1/4. Yes, they ate the same amount of pizza.
Example 3: Compare the fractions 5/6 and 7/9. Which is larger?
Solution: Find a common denominator. The least common multiple of 6 and 9 is 18. Convert both fractions: 5/6 = 15/18 and 7/9 = 14/18. Since 15/18 > 14/18, 5/6 is larger.
Conclusion: Mastering Equivalent Fractions
Understanding equivalent fractions is fundamental to mastering fractional arithmetic and its broader applications. By learning how to convert between mixed numbers and improper fractions, find equivalent fractions, simplify fractions, and visualize these concepts, you'll develop a solid foundation for more advanced mathematical concepts. The simple question of what fraction is equivalent to 1 ½ opens a door to a richer understanding of the world of fractions and their diverse applications in various fields. Remember to practice regularly to build confidence and fluency in working with fractions.
Latest Posts
Latest Posts
-
How Many Feet Is 168 Cm
May 14, 2025
-
What Is A Medium In Physics
May 14, 2025
-
5 Letter Words That Start With Hel
May 14, 2025
-
18 Cm Is How Many Inches
May 14, 2025
-
How Many Feet Is 100 Cm
May 14, 2025
Related Post
Thank you for visiting our website which covers about What Fraction Is Equivalent To 1 2 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.