What Are The Real Fourth Roots Of 256
Juapaving
May 10, 2025 · 4 min read
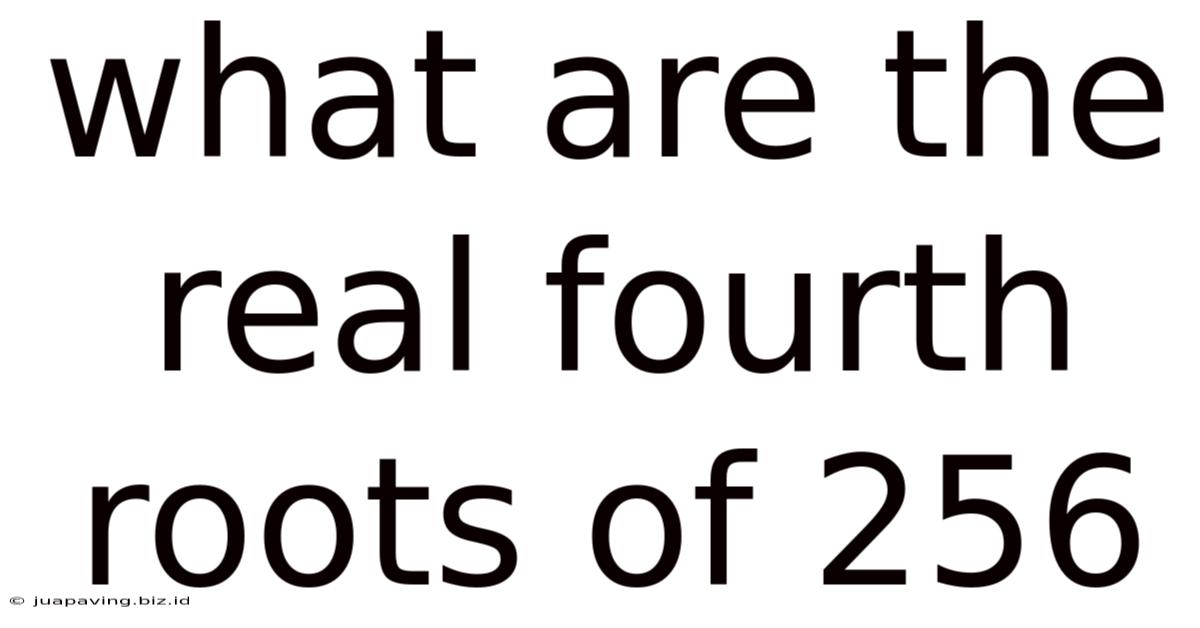
Table of Contents
What Are the Real Fourth Roots of 256? A Comprehensive Exploration
Finding the fourth root of a number involves determining which number, when multiplied by itself four times, yields the original number. While calculators can readily provide one answer, a deeper understanding requires exploring the concept mathematically and acknowledging the existence of multiple solutions, especially within the complex number system. This article delves into the intricacies of finding the real fourth roots of 256, providing a comprehensive explanation suitable for various levels of mathematical understanding.
Understanding Roots and Radicals
Before diving into the specifics of finding the fourth root of 256, let's revisit the fundamental concept of roots. The nth root of a number x, denoted as √ⁿx or x^(1/n), is a number that, when multiplied by itself n times, equals x. For example:
- The square root (n=2) of 9 is 3 because 3 * 3 = 9.
- The cube root (n=3) of 8 is 2 because 2 * 2 * 2 = 8.
- The fourth root (n=4) of 16 is 2 because 2 * 2 * 2 * 2 = 16.
In our case, we're interested in the fourth root of 256, meaning we need to find a number that, when multiplied by itself four times, equals 256.
Finding the Principal Fourth Root of 256
The most straightforward approach to finding the fourth root of 256 is to use a calculator or a computational tool. This typically provides the principal fourth root, which is the positive real number solution. In this case, the principal fourth root of 256 is 4 because 4 * 4 * 4 * 4 = 256.
However, limiting ourselves to the principal root ignores a crucial aspect of mathematics: many equations have multiple solutions.
Exploring Multiple Solutions: Beyond the Principal Root
While 4 is the principal fourth root of 256, there are other solutions, especially when considering the complex number system. The fundamental theorem of algebra states that a polynomial equation of degree n has exactly n roots (counting multiplicity) in the complex numbers. Since the equation x⁴ - 256 = 0 is a polynomial equation of degree 4, it must have four roots. Let's explore these solutions.
The Real Fourth Roots
We've already identified the positive real root, 4. Consider the equation x⁴ = 256. We can also consider the negative fourth root. Because (-4) * (-4) * (-4) * (-4) = 256, -4 is also a real fourth root of 256. Therefore, the two real fourth roots of 256 are 4 and -4.
The Complex Fourth Roots
To find the remaining two roots, we need to venture into the realm of complex numbers. Complex numbers are numbers of the form a + bi, where 'a' and 'b' are real numbers and 'i' is the imaginary unit (√-1). To find the complex roots, we can use De Moivre's theorem, which provides a method for finding the roots of complex numbers. However, for this particular problem, a simpler approach is possible.
We can express 256 in polar form as 256(cos 0 + i sin 0). Using De Moivre's theorem, the fourth roots are given by:
√⁴(256) * [cos((0 + 2kπ)/4) + i sin((0 + 2kπ)/4)]
where k = 0, 1, 2, 3.
This formula provides four distinct roots:
- k = 0: 4(cos 0 + i sin 0) = 4
- k = 1: 4(cos π/2 + i sin π/2) = 4i
- k = 2: 4(cos π + i sin π) = -4
- k = 3: 4(cos 3π/2 + i sin 3π/2) = -4i
Thus, the four fourth roots of 256 are 4, -4, 4i, and -4i.
Understanding the Significance of Multiple Roots
The existence of multiple roots highlights the importance of understanding the underlying mathematical principles. While a calculator might only provide the principal root, a complete understanding requires recognizing all possible solutions. This is crucial in various applications, such as solving polynomial equations in engineering, physics, and other scientific fields. Ignoring multiple roots can lead to incomplete or inaccurate solutions.
Applications of Fourth Roots
The concept of fourth roots, and roots in general, finds applications in numerous fields:
- Geometry: Calculating the side length of a hypercube (four-dimensional cube) given its hypervolume.
- Physics: Solving equations related to oscillations, waves, and other phenomena where powers of four are involved.
- Engineering: Designing structures and systems where the fourth power of a variable is a significant factor.
- Computer Science: Algorithms and data structures can utilize concepts of roots for efficient processing and data manipulation.
- Financial Mathematics: Calculating compound interest over a period of four years involves the fourth root to find the annual equivalent rate.
Conclusion: A Deeper Dive into the Fourth Roots of 256
While a quick calculation might yield only the principal fourth root of 256 (4), a thorough exploration reveals a richer mathematical landscape. We’ve shown that there are actually four fourth roots: two real numbers (4 and -4) and two complex numbers (4i and -4i). Understanding these multiple solutions is not just an academic exercise; it's a fundamental concept with practical applications across various scientific and engineering disciplines. This exploration highlights the importance of grasping the full scope of mathematical concepts, moving beyond simple calculations to comprehend the underlying theory and its implications. The journey from finding the simple answer to uncovering the full set of solutions underscores the beauty and power of mathematics.
Latest Posts
Latest Posts
-
Part Of The Brain That Controls Heartbeat
May 10, 2025
-
The Most Abundant Element In The Air
May 10, 2025
-
According To Mendels Law Of Independent Assortment
May 10, 2025
-
Chemical Element With Atomic Number 1
May 10, 2025
-
What Does Reticular Tissue Look Like
May 10, 2025
Related Post
Thank you for visiting our website which covers about What Are The Real Fourth Roots Of 256 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.