What Are The Prime Factors Of 735
Juapaving
May 10, 2025 · 4 min read
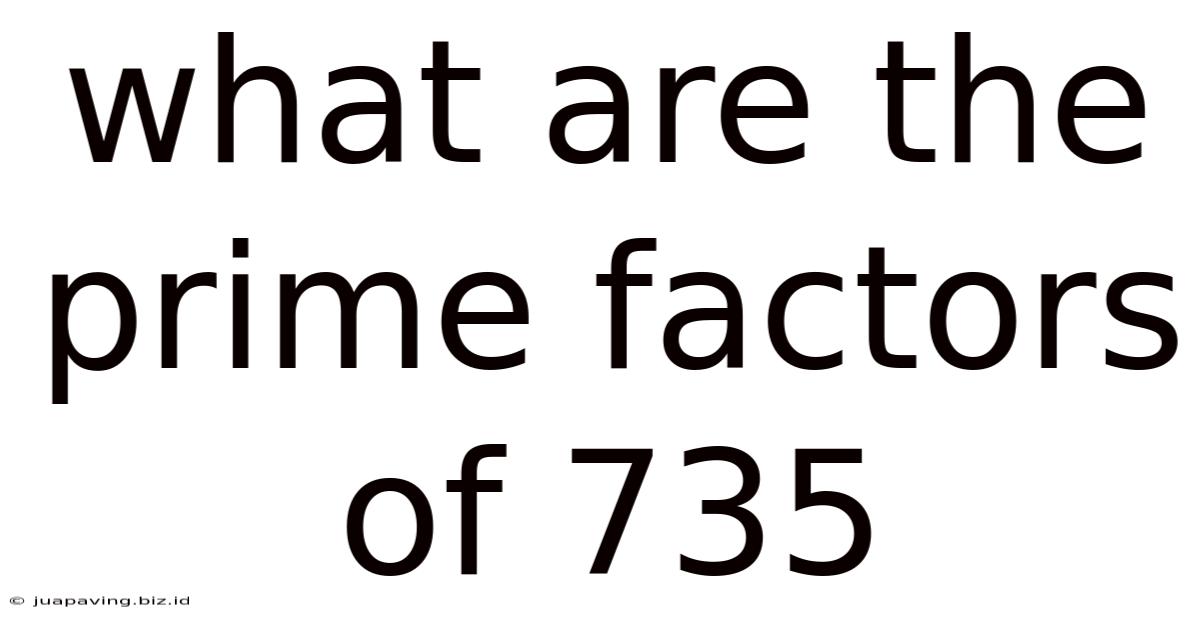
Table of Contents
What Are the Prime Factors of 735? A Deep Dive into Prime Factorization
Finding the prime factors of a number might seem like a simple mathematical exercise, but understanding the process reveals fundamental concepts in number theory and has practical applications in various fields, from cryptography to computer science. This article will not only uncover the prime factors of 735 but also explore the underlying theory and methods involved, offering a comprehensive understanding of prime factorization.
Understanding Prime Numbers and Prime Factorization
Before diving into the prime factors of 735, let's establish a solid foundation. A prime number is a whole number greater than 1 that has only two divisors: 1 and itself. The first few prime numbers are 2, 3, 5, 7, 11, 13, and so on. A number that is not prime is called a composite number.
Prime factorization is the process of expressing a composite number as a product of its prime factors. This representation is unique for every composite number; meaning there is only one way to express a composite number as a product of prime numbers (ignoring the order of the factors). This uniqueness is a cornerstone of number theory and has significant implications in various mathematical fields.
Finding the Prime Factors of 735: A Step-by-Step Approach
There are several methods to find the prime factors of a number. Let's explore two common approaches to determine the prime factorization of 735:
Method 1: Successive Division by Prime Numbers
This method involves systematically dividing the number by the smallest prime numbers, starting with 2, then 3, 5, 7, and so on, until the result is 1.
-
Divide by 2: 735 is not divisible by 2 (it's an odd number).
-
Divide by 3: 735 / 3 = 245. We find that 3 is a prime factor.
-
Divide by 3 again: 245 is not divisible by 3 (the sum of its digits, 2+4+5=11, is not divisible by 3).
-
Divide by 5: 245 / 5 = 49. We find that 5 is a prime factor.
-
Divide by 5 again: 49 is not divisible by 5.
-
Divide by 7: 49 / 7 = 7. We find that 7 is a prime factor.
-
Divide by 7 again: 7 / 7 = 1. We've reached 1, indicating we've found all the prime factors.
Therefore, the prime factorization of 735 is 3 x 5 x 7 x 7, or 3 x 5 x 7<sup>2</sup>.
Method 2: Factor Tree
A factor tree is a visual representation of the prime factorization process. It's particularly helpful for visualizing the steps involved, especially for larger numbers.
735
/ \
3 245
/ \
5 49
/ \
7 7
We start with 735 at the top. We find the smallest prime factor (3), and then divide 735 by 3 to get 245. We continue this process, branching out until we only have prime numbers at the bottom. The prime factors are the numbers at the end of each branch: 3, 5, 7, and 7. Again, the prime factorization is 3 x 5 x 7<sup>2</sup>.
Significance of Prime Factorization
Understanding prime factorization is crucial in numerous areas:
1. Cryptography:
Prime numbers are fundamental to many encryption algorithms. The difficulty of factoring large composite numbers into their prime factors is the basis of the security for systems like RSA encryption, widely used to secure online transactions.
2. Number Theory:
Prime factorization is a core concept in number theory, forming the basis for many theorems and proofs related to divisibility, modular arithmetic, and other important number-theoretic properties.
3. Computer Science:
Prime numbers are used in various algorithms and data structures in computer science, including hash tables, random number generation, and primality testing. Efficient algorithms for prime factorization are crucial for various computational tasks.
4. Mathematics Education:
Understanding prime factorization strengthens foundational mathematical skills, including division, multiplication, and number sense. It lays the groundwork for more advanced mathematical concepts.
Beyond 735: Exploring Other Factorization Techniques
While the methods above are effective for relatively small numbers like 735, larger numbers require more sophisticated techniques. These include:
-
Trial Division: A systematic approach of trying prime divisors until a factor is found. However, this becomes computationally expensive for very large numbers.
-
Pollard's Rho Algorithm: A probabilistic algorithm that is efficient for finding small prime factors of large composite numbers.
-
General Number Field Sieve (GNFS): Currently the most efficient known algorithm for factoring very large numbers, used to factor numbers with hundreds of digits.
Conclusion: The Power of Prime Factorization
The seemingly simple task of finding the prime factors of 735 – resulting in 3 x 5 x 7² – unveils a vast landscape of mathematical concepts and practical applications. From its role in securing online transactions to its fundamental importance in number theory and computer science, prime factorization is a cornerstone of modern mathematics and technology. Understanding the techniques and their significance allows for a deeper appreciation of the elegance and power of prime numbers and their unique properties. The exploration of prime factorization, even starting with a relatively small number like 735, provides a glimpse into the profound depths of mathematical inquiry. Further exploration into the more advanced factorization techniques will unlock even greater insights into the world of prime numbers and their pivotal role in numerous fields.
Latest Posts
Latest Posts
-
Find Area Of Triangle With Fractions
May 10, 2025
-
Green Plants That Make Their Own Food Are Called
May 10, 2025
-
Manuel Ate 1 3 Of The Crackers On A Plate
May 10, 2025
-
What Is The Difference Between An Antibiotic And An Antiseptic
May 10, 2025
-
What Is 2 3 4 5
May 10, 2025
Related Post
Thank you for visiting our website which covers about What Are The Prime Factors Of 735 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.