What Are The Common Factors Of 6 And 15
Juapaving
May 12, 2025 · 5 min read
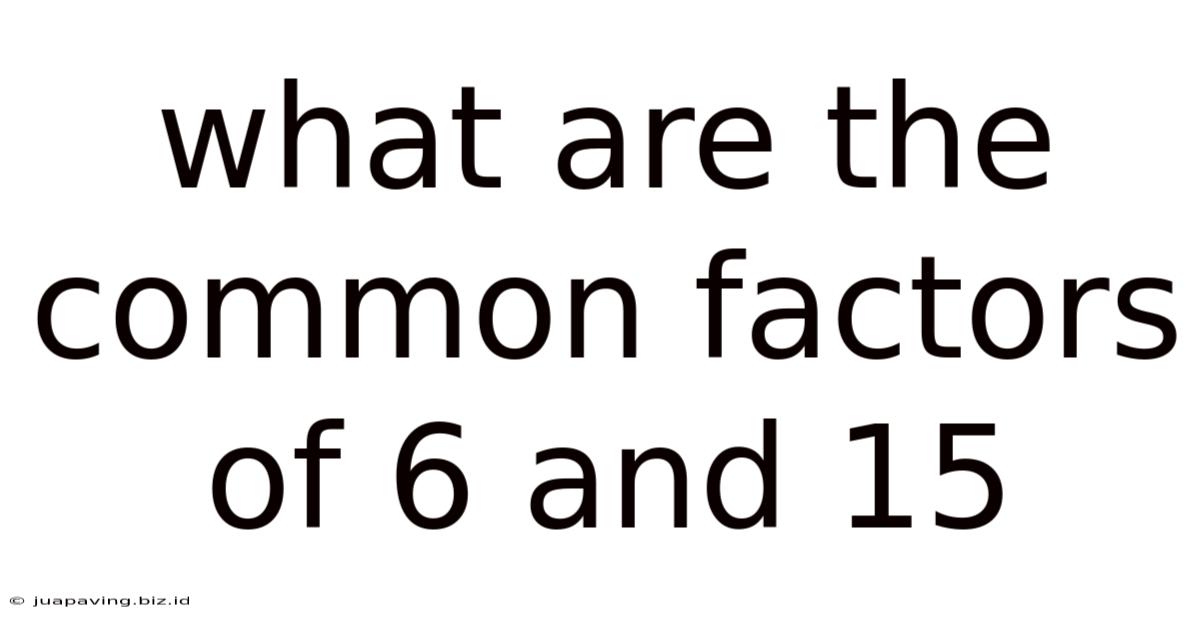
Table of Contents
What Are the Common Factors of 6 and 15? A Deep Dive into Number Theory
Finding the common factors of two numbers might seem like a simple arithmetic exercise, but it's a fundamental concept in number theory with applications across various fields, from cryptography to computer science. This article delves into the process of determining the common factors of 6 and 15, explaining the underlying concepts and providing a broader perspective on factor analysis. We'll explore different methods, discuss the significance of greatest common factors (GCFs), and touch upon related mathematical ideas.
Understanding Factors
Before we dive into finding the common factors of 6 and 15, let's clarify the term "factor." A factor of a number is any integer that divides that number evenly without leaving a remainder. For instance, the factors of 6 are 1, 2, 3, and 6 because each of these numbers divides 6 without leaving a remainder. Similarly, the factors of 15 are 1, 3, 5, and 15.
Listing the Factors of 6 and 15
Let's systematically list all the factors of 6 and 15:
Factors of 6: 1, 2, 3, 6
Factors of 15: 1, 3, 5, 15
Now, we can visually compare these lists to identify the numbers that appear in both. These shared numbers are the common factors.
Identifying Common Factors
Looking at the lists above, we can easily see that the numbers 1 and 3 are present in both the list of factors for 6 and the list of factors for 15.
Therefore, the common factors of 6 and 15 are 1 and 3.
The Greatest Common Factor (GCF)
Among the common factors, there's always one that's the largest – the greatest common factor (GCF), also known as the highest common factor (HCF). In the case of 6 and 15, the GCF is 3. The GCF is a crucial concept in various mathematical operations, particularly in simplifying fractions and solving algebraic equations.
Methods for Finding the GCF
While listing factors works well for smaller numbers, it becomes less efficient for larger numbers. Let's explore more systematic methods for determining the GCF:
1. Prime Factorization
Prime factorization involves breaking down a number into its prime factors – numbers divisible only by 1 and themselves. Let's apply this to 6 and 15:
- Prime factorization of 6: 2 x 3
- Prime factorization of 15: 3 x 5
By comparing the prime factorizations, we see that the only common prime factor is 3. Therefore, the GCF is 3. This method is particularly useful for larger numbers, as it provides a structured approach to finding common factors.
2. Euclidean Algorithm
The Euclidean algorithm is an efficient method for finding the GCF of two numbers, particularly useful for larger numbers where prime factorization might be cumbersome. It's based on the principle that the GCF of two numbers doesn't change if the larger number is replaced by its difference with the smaller number. This process is repeated until the two numbers are equal, which represents the GCF.
Let's illustrate with 6 and 15:
- 15 - 6 = 9 (Now we find the GCF of 6 and 9)
- 9 - 6 = 3 (Now we find the GCF of 6 and 3)
- 6 - 3 = 3 (Now we find the GCF of 3 and 3)
Since both numbers are now 3, the GCF of 6 and 15 is 3. The Euclidean algorithm's efficiency becomes more pronounced when dealing with much larger numbers.
Significance of Common Factors and GCF
The concepts of common factors and the GCF have significant applications in various areas:
-
Simplifying Fractions: The GCF is used to simplify fractions to their lowest terms. For example, the fraction 6/15 can be simplified to 2/5 by dividing both the numerator (6) and the denominator (15) by their GCF, which is 3.
-
Solving Equations: GCF plays a vital role in solving certain types of algebraic equations, particularly those involving polynomial expressions.
-
Cryptography: Number theory, including concepts like GCF, forms the foundation of many modern cryptographic algorithms used to secure online communications.
-
Computer Science: Algorithms related to GCF finding are used in various computer science applications, such as optimizing data structures and solving computational problems.
-
Modular Arithmetic: The concept of GCF is fundamental in understanding modular arithmetic, a branch of number theory with applications in cryptography and computer science. The GCF helps determine if two numbers are relatively prime (their GCF is 1), a key property in many cryptographic systems.
Beyond the Basics: Exploring Related Concepts
Understanding common factors opens doors to exploring several related and more advanced number theory concepts:
-
Least Common Multiple (LCM): The least common multiple (LCM) of two numbers is the smallest number that is a multiple of both numbers. Finding the LCM is closely related to finding the GCF, and there are formulas that connect the two.
-
Relatively Prime Numbers: Two numbers are considered relatively prime if their GCF is 1. This concept is crucial in number theory and has significant applications in cryptography.
-
Diophantine Equations: These are algebraic equations where only integer solutions are sought. Finding GCFs often plays a key role in solving certain types of Diophantine equations.
-
Modular Inverses: In modular arithmetic, the modular inverse of a number exists if and only if the number and the modulus are relatively prime (their GCF is 1). This is a crucial concept in cryptography.
Conclusion: The Importance of Foundational Math
While finding the common factors of 6 and 15 might seem elementary, the underlying principles are fundamental to a deeper understanding of number theory and its applications in various fields. Mastering these concepts provides a strong foundation for tackling more complex mathematical problems and appreciating the elegance and power of mathematical structures. The seemingly simple task of finding common factors serves as a gateway to a vast and fascinating world of mathematical exploration. From simplifying fractions to securing online communications, the impact of these fundamental concepts is far-reaching and undeniable. So, the next time you encounter a problem involving factors, remember the power and versatility of this fundamental mathematical idea.
Latest Posts
Latest Posts
-
Formula To Find Perimeter Of Square
May 12, 2025
-
Are Ribosomes In Eukaryotes Or Prokaryotes
May 12, 2025
-
Which Of The Following Statements About Diabetes Is True
May 12, 2025
-
Bacteria Reproduce By A Process Called
May 12, 2025
-
What Is The Square Root Of 3 2
May 12, 2025
Related Post
Thank you for visiting our website which covers about What Are The Common Factors Of 6 And 15 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.