Moment Of Inertia Of A Wheel
Juapaving
Mar 26, 2025 · 7 min read
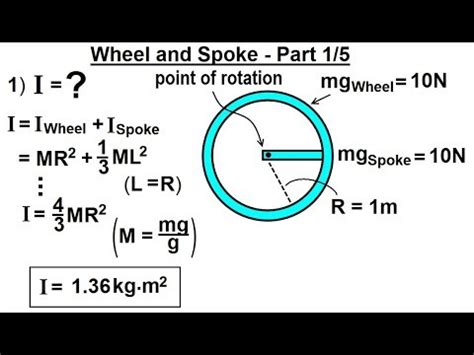
Table of Contents
Moment of Inertia of a Wheel: A Deep Dive into Rotational Inertia
The moment of inertia, a crucial concept in physics and engineering, describes an object's resistance to changes in its rotational motion. Think of it as the rotational equivalent of mass in linear motion. A heavier object resists linear acceleration more than a lighter one; similarly, an object with a higher moment of inertia resists changes in its rotational speed more than one with a lower moment of inertia. This article delves into the fascinating world of the moment of inertia, focusing specifically on the wheel – a ubiquitous object whose rotational properties are fundamental to countless applications.
Understanding Moment of Inertia
Before we delve into the specifics of a wheel, let's establish a firm grasp of the fundamental principles of moment of inertia. It's denoted by the symbol I and is calculated differently depending on the object's shape and the axis of rotation. The general formula is:
I = Σ mᵢrᵢ²
Where:
- I is the moment of inertia
- mᵢ is the mass of each individual particle making up the object
- rᵢ is the perpendicular distance of each particle from the axis of rotation
This formula essentially sums up the contribution of each tiny mass element to the object's overall resistance to rotational acceleration. The further the mass is distributed from the axis of rotation, the greater its contribution to the moment of inertia, and thus the harder it is to change the object's rotation.
This concept becomes particularly relevant when considering rotating objects like wheels, flywheels, and gears, which are integral components in various machines and vehicles.
The Moment of Inertia of a Simple Wheel
Let's consider a simplified model of a wheel: a thin, uniform ring or hoop rotating about an axis perpendicular to its plane and passing through its center. In this case, all the mass is concentrated at a distance R (the radius of the hoop) from the axis of rotation. The formula simplifies significantly:
I = MR²
Where:
- M is the total mass of the hoop
- R is the radius of the hoop
This equation clearly demonstrates the direct proportionality between the moment of inertia, mass, and the square of the radius. Doubling the mass doubles the moment of inertia; doubling the radius quadruples it. This highlights the significant influence of the radial distribution of mass on the rotational inertia.
Moment of Inertia of a More Realistic Wheel
Real-world wheels aren't typically thin hoops. They have a significant thickness and mass distributed throughout the structure. To calculate the moment of inertia for a more realistic wheel, we need to consider a solid cylinder or disk. The formula for a solid cylinder rotating about its central axis is:
I = (1/2)MR²
Notice the crucial difference: the moment of inertia of a solid cylinder is half that of a thin hoop with the same mass and radius. This is because the mass in a solid cylinder is distributed closer to the axis of rotation on average compared to a hoop where all the mass is at the maximum distance.
Factors Affecting a Wheel's Moment of Inertia
Several factors significantly influence a wheel's moment of inertia:
1. Mass:
As indicated by the formulas, a wheel's mass is directly proportional to its moment of inertia. A heavier wheel will have a greater resistance to changes in rotational motion. This is intuitively clear; it requires more effort to accelerate or decelerate a heavier wheel.
2. Radius:
The radius plays a more significant role, as it's squared in the formula. Increasing the radius dramatically increases the moment of inertia. This is because the mass is distributed further from the axis of rotation, requiring more torque to change the rotation. This is why larger diameter wheels tend to resist changes in their rotational speed more than smaller ones.
3. Mass Distribution:
The distribution of mass within the wheel is critical. A wheel with mass concentrated near the rim (like a hoop) has a higher moment of inertia than a wheel with mass distributed more evenly towards the center (like a solid disk). This explains why spoked wheels, with their mass concentrated towards the rim, have a larger moment of inertia compared to solid wheels of the same mass and radius.
4. Wheel Material:
The density of the wheel's material also influences its moment of inertia. A denser material, for the same mass and radius, will generally result in a smaller wheel and therefore a smaller moment of inertia.
The Significance of Moment of Inertia in Wheel Applications
The moment of inertia is a crucial parameter in various applications involving wheels:
1. Vehicle Dynamics:
In automobiles, the moment of inertia of the wheels significantly impacts acceleration, braking, and cornering performance. Wheels with lower moment of inertia respond more quickly to changes in speed and direction, leading to improved handling and maneuverability. This is why lightweight wheels are often preferred in performance vehicles.
2. Flywheels:
Flywheels, which are essentially high-inertia wheels, are used in energy storage systems. Their high moment of inertia allows them to store significant rotational kinetic energy, which can be released later to power machinery or smooth out fluctuations in power supply.
3. Robotics:
In robotics, the moment of inertia of wheels is critical for precise control of robot movement. Precise knowledge of the wheel's moment of inertia is essential for accurate modeling and control algorithms.
4. Rotating Machinery:
In various rotating machines, from generators to turbines, the moment of inertia of the rotating components is crucial for designing robust and efficient systems. Understanding the moment of inertia allows engineers to predict the system's response to changes in torque and speed, ensuring optimal performance and stability.
Calculating Moment of Inertia for Complex Wheel Geometries
Calculating the moment of inertia for complex wheel designs, such as those with intricate spokes or varying thicknesses, requires more advanced techniques. These often involve integral calculus, breaking down the wheel into smaller, simpler shapes, and then summing the individual moments of inertia. Numerical methods and computer simulations are often employed to tackle the complexities of these calculations. Software packages specifically designed for engineering analysis provide powerful tools for efficiently determining the moment of inertia of such complex geometries.
Practical Applications and Considerations
Understanding the moment of inertia of a wheel is not merely a theoretical exercise. It has far-reaching practical implications across various engineering disciplines. Consider these examples:
-
Automotive Engineering: Designing lightweight, high-performance wheels requires precise calculations of moment of inertia to optimize handling and fuel efficiency. Minimizing the moment of inertia allows for quicker acceleration and deceleration, enhancing the overall driving experience.
-
Aerospace Engineering: In aircraft and spacecraft, the moment of inertia of rotating components like wheels, gyroscopes, and turbines is critical for stability and control. Accurate calculation is vital for ensuring safe and reliable operation.
-
Mechanical Engineering: The design of gears, pulleys, and other rotating machine elements necessitates careful consideration of moment of inertia to optimize performance and reduce wear and tear.
Conclusion: Mastering the Moment of Inertia
The moment of inertia of a wheel is a fundamental concept with extensive practical implications. Understanding how mass distribution, radius, and material properties influence rotational inertia is crucial for engineers and physicists alike. From designing high-performance vehicles to developing efficient energy storage systems, mastering the principles of moment of inertia unlocks possibilities for innovation and technological advancement. This article provides a comprehensive overview, from basic principles to advanced considerations, equipping you with a deeper understanding of this essential concept. Further exploration into the intricacies of rotational dynamics will undoubtedly reveal even more fascinating aspects of this critical physical property. Remember to always consider the specific geometry and mass distribution of the wheel when performing calculations to ensure accuracy and relevance to your application.
Latest Posts
Latest Posts
-
How Many Factors Does 10 Have
Mar 29, 2025
-
Lcm Of 6 5 And 3
Mar 29, 2025
-
How Many Meters Are In 7 Feet
Mar 29, 2025
-
In Photosynthesis Light Energy Is Converted To
Mar 29, 2025
-
1000 M Is Equal To How Many Km
Mar 29, 2025
Related Post
Thank you for visiting our website which covers about Moment Of Inertia Of A Wheel . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
