Mass Of An Electron In Mev
Juapaving
Mar 31, 2025 · 5 min read
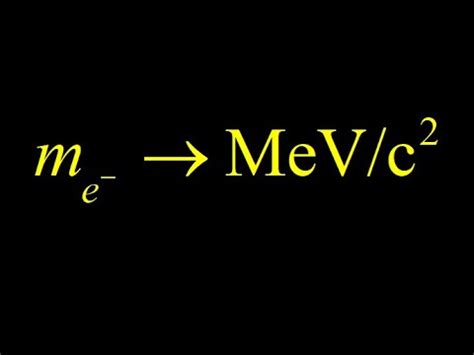
Table of Contents
Mass of an Electron in MeV: A Deep Dive into Particle Physics
The mass of an electron, a fundamental particle of matter, is a cornerstone concept in physics. While often expressed in kilograms (kg), understanding its equivalent in mega-electronvolts (MeV) provides crucial insights into particle interactions and energy conversions within the realm of particle physics and nuclear physics. This article delves deep into the meaning, calculation, significance, and applications of the electron's mass in MeV.
Understanding the Electron's Mass
The electron, a lepton with a negative elementary electric charge, is a fundamental constituent of atoms. Its mass, a measure of its inertia and gravitational interaction, is incredibly small. In kilograms, the electron's mass is approximately 9.109 x 10⁻³¹ kg. However, in the world of particle physics, expressing this mass in MeV provides a more intuitive and practical understanding of its energy equivalence.
From Kilograms to MeV: Einstein's Equation
The connection between mass and energy is elegantly captured by Einstein's famous equation, E=mc², where:
- E represents energy
- m represents mass
- c represents the speed of light in a vacuum (approximately 3 x 10⁸ m/s)
This equation reveals the inherent energy contained within any mass. A small mass, when converted entirely to energy, can release a tremendous amount of energy. This principle is fundamental to nuclear reactions and particle physics processes.
Calculating the Electron Mass in MeV
To convert the electron's mass from kilograms to MeV, we use Einstein's equation and appropriate unit conversions. Here's the breakdown:
-
Start with the electron's mass in kilograms: 9.109 x 10⁻³¹ kg
-
Multiply by the square of the speed of light: (3 x 10⁸ m/s)²
-
Convert joules to electronvolts: 1 joule (J) is equal to 6.242 x 10¹⁸ electronvolts (eV).
-
Convert electronvolts to mega-electronvolts: 1 MeV = 10⁶ eV.
The complete calculation yields a value of approximately 0.511 MeV. This means that the rest mass energy of an electron is equivalent to 0.511 MeV. This is a remarkably useful value in particle physics calculations.
Significance of the Electron Mass in MeV
The electron's mass in MeV has profound implications across various domains of physics:
1. Particle Physics Interactions:
The 0.511 MeV value plays a crucial role in calculating the energy thresholds for various particle interactions. For instance, in electron-positron annihilation, where an electron and its antiparticle (positron) collide and annihilate, the minimum energy required for this process is twice the electron's rest mass energy – 1.022 MeV. This threshold energy is directly derived from the electron's mass in MeV. Understanding this threshold is essential in designing and interpreting experiments involving electron-positron collisions.
2. Nuclear Physics and Beta Decay:
Beta decay, a type of radioactive decay, involves the emission of electrons (beta particles) from an unstable atomic nucleus. The energy spectrum of these emitted electrons is directly related to the electron's mass and the energy released during the decay process. The 0.511 MeV value helps in predicting and analyzing the energy distribution of beta particles.
3. Astrophysics and Cosmology:
The mass of the electron has significant implications in astrophysical processes. For instance, the electron's mass influences the stability of stars and the behavior of plasmas in stellar interiors. Accurate knowledge of the electron mass is crucial for developing and validating astrophysical models.
4. Quantum Electrodynamics (QED):
QED, a quantum field theory describing the interaction of light and matter, relies heavily on precise measurements of the electron's mass and charge. These fundamental constants are input parameters in QED calculations, and their accuracy dictates the precision of theoretical predictions.
Advanced Concepts and Applications
The electron's mass in MeV opens doors to several advanced concepts and applications:
1. Pair Production:
High-energy photons (gamma rays) can convert into electron-positron pairs under specific conditions. This process, known as pair production, requires a minimum photon energy equal to 1.022 MeV (twice the electron's rest mass energy). This threshold highlights the mass-energy equivalence in a dramatic manner.
2. Compton Scattering:
Compton scattering is the inelastic scattering of a photon by a charged particle, typically an electron. The change in the photon's wavelength depends on the electron's mass, and precise measurements of this scattering can be used to determine the electron's mass with high accuracy.
3. Electron-Muon Mass Ratio:
The ratio of the electron's mass to the muon's mass (another lepton) is a subject of intense study in particle physics. This ratio is not precisely predicted by the Standard Model of particle physics and its precise measurement may shed light on physics beyond the Standard Model.
Experimental Determination of Electron Mass
The electron's mass is not a theoretical constant derived from first principles but rather a precisely measured quantity. Several experimental methods have been employed to determine this fundamental constant, including:
- Spectroscopy: Precise measurements of atomic energy levels, particularly those sensitive to the electron's mass, are used to infer its value.
- Cyclotron resonance: The electron's cyclotron frequency in a magnetic field depends on its mass-to-charge ratio, which enables a highly precise determination of its mass.
- Quantum Hall effect: This quantum phenomenon provides a way to determine fundamental constants, including the electron's mass, with exceptional accuracy.
These experiments have yielded increasingly accurate values for the electron's mass, confirming its stability and providing crucial input for various theoretical models.
Conclusion: The Ubiquitous Role of the Electron Mass
The electron's mass, whether expressed in kilograms or MeV, is a fundamental constant with far-reaching implications in various branches of physics. Its equivalent in MeV, 0.511 MeV, is particularly useful in particle physics, providing a crucial link between mass and energy. Understanding this value is essential for comprehending a wide range of physical phenomena, from elementary particle interactions to astrophysical processes. The ongoing efforts to refine the measurement of the electron's mass reflect its fundamental importance in our quest to understand the universe at its most basic level. Future research promises further refinement of this value and its role in exploring the frontiers of physics. The seemingly small mass of the electron, expressed eloquently in MeV, holds a key to unlocking many of nature's most profound secrets.
Latest Posts
Latest Posts
-
What Is The Only Movable Bone Of The Skull
Apr 02, 2025
-
How Many Inches Are In One Meter
Apr 02, 2025
-
What Is The Freezing Point Of Water In Kelvin Scale
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about Mass Of An Electron In Mev . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
