Least Common Multiple Of 48 And 16
Juapaving
Apr 03, 2025 · 5 min read
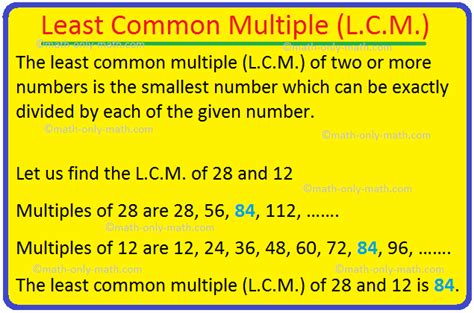
Table of Contents
Finding the Least Common Multiple (LCM) of 48 and 16: A Comprehensive Guide
The least common multiple (LCM) is a fundamental concept in arithmetic and number theory. It represents the smallest positive integer that is a multiple of two or more integers. Understanding how to find the LCM is crucial in various mathematical applications, from simplifying fractions to solving problems in algebra and beyond. This comprehensive guide will delve into the methods for calculating the LCM of 48 and 16, providing a detailed explanation and exploring various approaches. We'll also examine the broader context of LCMs and their significance in mathematics.
Understanding Least Common Multiples
Before we jump into calculating the LCM of 48 and 16, let's solidify our understanding of what an LCM actually is. The LCM of two or more integers is the smallest positive integer that is divisible by all the given integers without leaving a remainder. For example, the LCM of 2 and 3 is 6 because 6 is the smallest positive integer that is divisible by both 2 and 3.
Key Characteristics of LCMs:
- Positive Integer: The LCM is always a positive whole number.
- Divisibility: The LCM is divisible by all the numbers whose LCM is being calculated.
- Smallest Multiple: It's the smallest number that satisfies the divisibility condition.
The concept of LCM is closely related to the greatest common divisor (GCD). The GCD is the largest positive integer that divides each of the given integers without leaving a remainder. There's a crucial relationship between the LCM and GCD: For any two integers 'a' and 'b', the product of their LCM and GCD is equal to the product of the two integers themselves. This relationship provides an alternative method for calculating the LCM. This is formally expressed as:
LCM(a, b) * GCD(a, b) = a * b
Methods for Calculating the LCM of 48 and 16
Now, let's apply our understanding to find the LCM of 48 and 16. We'll explore three common methods: the listing method, the prime factorization method, and the method using the GCD.
1. The Listing Method
This method involves listing the multiples of each number until we find the smallest common multiple.
Multiples of 48: 48, 96, 144, 192, 240, 288, 336, 384, 432, 480...
Multiples of 16: 16, 32, 48, 64, 80, 96, 112, 128, 144, 160...
By comparing the lists, we can see that the smallest common multiple is 48. Therefore, LCM(48, 16) = 48.
While this method is straightforward for smaller numbers, it becomes less efficient as the numbers get larger.
2. The Prime Factorization Method
This is a more efficient and systematic method, particularly for larger numbers. It involves finding the prime factorization of each number and then constructing the LCM using the highest powers of each prime factor present in the factorizations.
Prime factorization of 48:
48 = 2 x 24 = 2 x 2 x 12 = 2 x 2 x 2 x 6 = 2 x 2 x 2 x 2 x 3 = 2<sup>4</sup> x 3<sup>1</sup>
Prime factorization of 16:
16 = 2 x 8 = 2 x 2 x 4 = 2 x 2 x 2 x 2 = 2<sup>4</sup>
To find the LCM, we take the highest power of each prime factor present in either factorization:
- The highest power of 2 is 2<sup>4</sup> = 16
- The highest power of 3 is 3<sup>1</sup> = 3
Therefore, LCM(48, 16) = 2<sup>4</sup> x 3<sup>1</sup> = 16 x 3 = 48
3. The GCD Method
This method leverages the relationship between the LCM and GCD. First, we need to find the GCD of 48 and 16. We can use the Euclidean algorithm for this:
- Divide 48 by 16: 48 = 16 x 3 + 0
- The remainder is 0, so the GCD is 16.
Now, we can use the formula:
LCM(a, b) = (a * b) / GCD(a, b)
LCM(48, 16) = (48 * 16) / 16 = 48
Applications of LCMs
The concept of LCM extends far beyond simple arithmetic calculations. It finds applications in various areas, including:
-
Fraction Addition and Subtraction: Finding the LCM of the denominators is crucial when adding or subtracting fractions with different denominators. This allows us to find a common denominator, enabling us to perform the operation.
-
Scheduling Problems: LCMs are used to solve problems involving recurring events. For example, if two buses leave a station at different intervals, the LCM of their intervals will tell us when they will depart at the same time again.
-
Modular Arithmetic: In modular arithmetic, the LCM plays a vital role in solving congruence problems.
-
Music Theory: LCMs are used to calculate the least common period of musical notes with different frequencies.
-
Computer Science: LCM is utilized in algorithms related to synchronization and scheduling in parallel processing.
Further Exploration of LCMs and Related Concepts
To deepen your understanding of LCMs, consider exploring these related concepts:
-
Greatest Common Divisor (GCD): As discussed earlier, the GCD is intimately related to the LCM. Mastering the calculation of GCDs significantly enhances your ability to compute LCMs.
-
Euclidean Algorithm: This efficient algorithm is commonly used for finding the GCD of two integers.
-
Prime Factorization: Understanding prime factorization is fundamental to effectively calculating LCMs, especially for larger numbers.
-
Modular Arithmetic and Congruences: Explore how LCMs are used to solve problems involving congruences and modular arithmetic.
-
Least Common Multiple of More Than Two Numbers: The methods described above can be extended to find the LCM of more than two numbers. The prime factorization method is particularly useful in this case.
Conclusion
The calculation of the least common multiple, while seemingly simple, is a crucial concept with wide-ranging applications across various fields of mathematics and beyond. Understanding the different methods for calculating the LCM, particularly the prime factorization method, empowers you to efficiently solve problems involving multiples and divisibility. By grasping the relationship between the LCM and GCD, and by exploring related concepts, you build a solid foundation for tackling more advanced mathematical problems. The example of finding the LCM of 48 and 16, as demonstrated through different approaches, provides a clear illustration of these principles and their practical application. Remember, the more you practice, the more comfortable and proficient you'll become in calculating LCMs and utilizing them in problem-solving scenarios.
Latest Posts
Latest Posts
-
Land Surrounded By Water On 3 Sides
Apr 04, 2025
-
Compare And Contrast Mixtures And Solutions
Apr 04, 2025
-
Darkest Part Of The Moons Shadow
Apr 04, 2025
-
How Much 50 Cm In Inches
Apr 04, 2025
-
Find The Sum Of The Infinite Geometric Series Calculator
Apr 04, 2025
Related Post
Thank you for visiting our website which covers about Least Common Multiple Of 48 And 16 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
