Least Common Denominator Of 4 And 8
Juapaving
Apr 03, 2025 · 5 min read
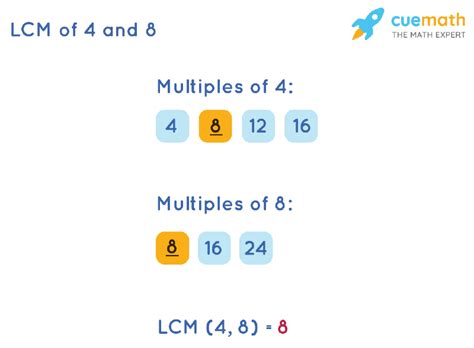
Table of Contents
Finding the Least Common Denominator (LCD) of 4 and 8: A Comprehensive Guide
Finding the least common denominator (LCD) is a fundamental concept in mathematics, particularly crucial when adding or subtracting fractions. Understanding how to determine the LCD allows for efficient and accurate calculations. This article will delve into the process of finding the LCD of 4 and 8, exploring various methods and providing a comprehensive understanding of the underlying principles. We'll move beyond simply stating the answer and explore the why behind the process, making this concept clear for students and anyone looking to refresh their math skills.
What is a Least Common Denominator (LCD)?
The least common denominator (LCD), also known as the least common multiple (LCM) of the denominators, is the smallest number that is a multiple of all the denominators in a set of fractions. When adding or subtracting fractions, having a common denominator is essential. The LCD simplifies the process, ensuring accurate results. Instead of working with disparate fractional parts, we unify them under a single, shared denominator.
Why is the LCD important?
Imagine trying to add 1/4 and 3/8 without finding a common denominator. You can't directly add the numerators because the fractional parts represent different sizes. The LCD provides the common "unit" allowing for a direct comparison and combination of the fractions.
Methods for Finding the LCD of 4 and 8
Several methods can determine the LCD of 4 and 8. We'll explore three common approaches:
Method 1: Listing Multiples
This is a straightforward method, especially useful when dealing with smaller numbers. We simply list the multiples of each number until we find the smallest common multiple.
- Multiples of 4: 4, 8, 12, 16, 20, 24...
- Multiples of 8: 8, 16, 24, 32...
By comparing the lists, we observe that the smallest number appearing in both lists is 8. Therefore, the LCD of 4 and 8 is 8.
Method 2: Prime Factorization
Prime factorization is a more robust method, particularly useful for larger numbers or when dealing with multiple denominators. It involves breaking down each number into its prime factors.
- Prime factorization of 4: 2 x 2 = 2²
- Prime factorization of 8: 2 x 2 x 2 = 2³
To find the LCD, we take the highest power of each prime factor present in the factorizations:
- The highest power of 2 is 2³.
- Therefore, the LCD is 2³ = 8.
This method is particularly helpful when dealing with more complex scenarios. For instance, finding the LCD of 12 and 18:
- 12 = 2² x 3
- 18 = 2 x 3²
- LCD = 2² x 3² = 36
Method 3: Using the Greatest Common Divisor (GCD)
The greatest common divisor (GCD) is the largest number that divides both numbers without leaving a remainder. The relationship between the LCD and GCD is expressed by the following formula:
LCD(a, b) = (a x b) / GCD(a, b)
Let's apply this to 4 and 8:
- GCD(4, 8) = 4 (4 is the largest number that divides both 4 and 8)
- LCD(4, 8) = (4 x 8) / 4 = 8
This method provides a concise way to calculate the LCD, particularly helpful for larger numbers where listing multiples might be tedious.
Applying the LCD: Adding and Subtracting Fractions
The primary application of the LCD is in adding and subtracting fractions. Let's illustrate with an example using 4 and 8 as denominators.
Suppose we need to add 1/4 and 3/8:
-
Find the LCD: As we've established, the LCD of 4 and 8 is 8.
-
Convert fractions to equivalent fractions with the LCD:
-
1/4 can be converted to an equivalent fraction with a denominator of 8 by multiplying both the numerator and denominator by 2: (1 x 2) / (4 x 2) = 2/8
-
3/8 already has a denominator of 8.
-
-
Add the numerators:
- 2/8 + 3/8 = 5/8
Therefore, 1/4 + 3/8 = 5/8.
Beyond 4 and 8: Extending the Concepts
The principles we've explored for finding the LCD of 4 and 8 are applicable to any set of numbers. Let's consider a more complex example: finding the LCD of 6, 15, and 20.
-
Prime Factorization:
- 6 = 2 x 3
- 15 = 3 x 5
- 20 = 2² x 5
-
Identify the highest power of each prime factor:
- Highest power of 2: 2²
- Highest power of 3: 3¹
- Highest power of 5: 5¹
-
Multiply the highest powers together:
- LCD = 2² x 3 x 5 = 60
Therefore, the LCD of 6, 15, and 20 is 60.
Real-World Applications of LCDs
While the concept might seem purely mathematical, the LCD has practical applications in various real-world scenarios:
-
Measurement Conversions: Converting between different units of measurement often involves working with fractions. The LCD helps ensure accurate conversions. For example, converting inches to feet requires a common denominator to correctly add or subtract fractional parts.
-
Recipe Scaling: Adjusting recipe sizes frequently requires working with fractions. The LCD ensures ingredients are scaled proportionally. For instance, if a recipe calls for 1/2 cup of sugar and you want to double it, the LCD helps calculate the correct amount of sugar needed.
-
Finance and Budgeting: Calculating percentages, dividing resources, or comparing financial data involves fractions and often requires finding a common denominator for accurate calculations.
Conclusion: Mastering the LCD
Understanding how to find the least common denominator is a cornerstone of fractional arithmetic. The methods described—listing multiples, prime factorization, and using the GCD—provide versatile tools for determining the LCD in various situations. Mastering these techniques not only simplifies fraction calculations but also unlocks the ability to solve practical problems that rely on fractional arithmetic. Remember, the key is to choose the method that best suits the numbers you're working with and to always double-check your work to ensure accuracy. The ability to confidently find the LCD translates to a deeper understanding of mathematical operations and the ability to confidently tackle more advanced concepts.
Latest Posts
Latest Posts
-
Land Surrounded By Water On 3 Sides
Apr 04, 2025
-
Compare And Contrast Mixtures And Solutions
Apr 04, 2025
-
Darkest Part Of The Moons Shadow
Apr 04, 2025
-
How Much 50 Cm In Inches
Apr 04, 2025
-
Find The Sum Of The Infinite Geometric Series Calculator
Apr 04, 2025
Related Post
Thank you for visiting our website which covers about Least Common Denominator Of 4 And 8 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
