Is 65 A Multiple Of 5
Juapaving
May 12, 2025 · 5 min read
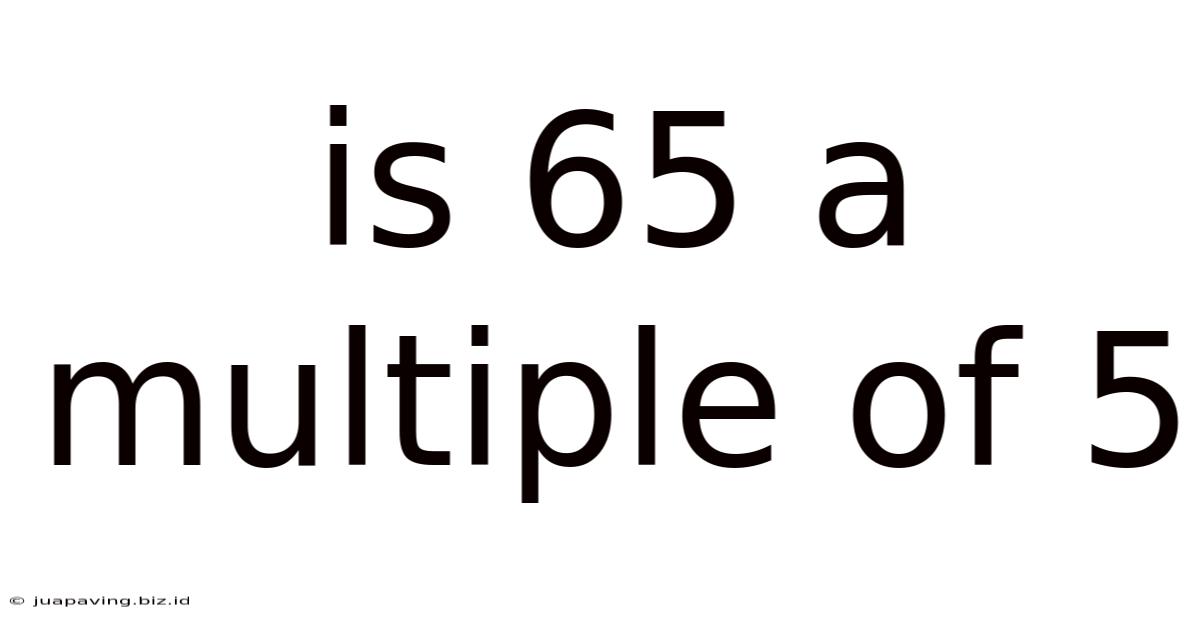
Table of Contents
Is 65 a Multiple of 5? A Deep Dive into Divisibility and Factors
The question, "Is 65 a multiple of 5?" might seem deceptively simple. A quick mental calculation might lead to an immediate answer. However, exploring this seemingly straightforward question allows us to delve into fundamental concepts of mathematics, specifically divisibility rules, prime factorization, and the broader concept of multiples and factors. This exploration will be particularly valuable for students learning these core mathematical principles and for anyone seeking a refresher on these essential concepts.
Understanding Multiples and Divisibility
Before we tackle the specific question of whether 65 is a multiple of 5, let's define the key terms involved.
Multiple: A multiple of a number is the product of that number and any integer (whole number). For example, multiples of 5 include 5 (5 x 1), 10 (5 x 2), 15 (5 x 3), 20 (5 x 4), and so on. The multiples of 5 extend infinitely in both positive and negative directions.
Divisibility: Divisibility refers to whether one number can be divided by another number without leaving a remainder. If a number is divisible by another, it means the second number is a factor of the first. In our case, we want to determine if 65 is divisible by 5.
Factor: A factor of a number is a whole number that divides evenly into that number. For example, the factors of 12 are 1, 2, 3, 4, 6, and 12.
Applying the Divisibility Rule for 5
One of the simplest divisibility rules is the rule for 5. A number is divisible by 5 if its last digit is either 0 or 5. Let's apply this rule to 65:
The last digit of 65 is 5. Therefore, according to the divisibility rule for 5, 65 is divisible by 5. This directly answers our initial question: Yes, 65 is a multiple of 5.
Proof through Division
We can further solidify our answer by performing the actual division:
65 ÷ 5 = 13
Since the division results in a whole number (13) with no remainder, this confirms that 65 is indeed divisible by 5, and thus, a multiple of 5.
Exploring Prime Factorization
Prime factorization is the process of expressing a number as a product of its prime factors (prime numbers are numbers greater than 1 that are only divisible by 1 and themselves). Understanding the prime factorization of a number can reveal valuable information about its divisors and multiples.
Let's find the prime factorization of 65:
- 65 is divisible by 5 (as we've already established).
- 65 ÷ 5 = 13
- 13 is a prime number.
Therefore, the prime factorization of 65 is 5 x 13. The presence of 5 as a prime factor explicitly demonstrates that 65 is a multiple of 5.
Practical Applications of Divisibility and Multiples
The concepts of divisibility and multiples are fundamental in various mathematical applications, including:
-
Simplification of fractions: Understanding divisibility helps us simplify fractions to their lowest terms. For example, the fraction 65/100 can be simplified by dividing both the numerator and the denominator by 5, resulting in the equivalent fraction 13/20.
-
Solving equations: Divisibility rules can aid in solving algebraic equations. For instance, if an equation involves a multiple of 5, we might be able to simplify the equation by dividing by 5.
-
Measurement and conversions: Multiples are frequently used in unit conversions. For instance, converting centimeters to meters involves understanding that 1 meter is equal to 100 centimeters, a multiple of 100.
-
Geometry: Multiples are crucial in geometrical calculations, particularly when dealing with areas and volumes of shapes.
Beyond the Basics: Exploring Further Mathematical Concepts
The seemingly simple question of whether 65 is a multiple of 5 has opened doors to broader mathematical concepts. Let's extend our exploration:
Least Common Multiple (LCM) and Greatest Common Factor (GCF)
-
Least Common Multiple (LCM): The LCM of two or more numbers is the smallest number that is a multiple of all the given numbers. Finding the LCM is often necessary when working with fractions and solving problems involving cycles or repeating patterns.
-
Greatest Common Factor (GCF): The GCF of two or more numbers is the largest number that divides evenly into all the given numbers. The GCF is useful in simplifying fractions and solving problems involving proportions.
To find the LCM and GCF of 65 and another number, say 15, we can use prime factorization.
The prime factorization of 15 is 3 x 5.
-
GCF(65, 15): The only common prime factor between 65 (5 x 13) and 15 (3 x 5) is 5. Therefore, the GCF(65, 15) = 5.
-
LCM(65, 15): To find the LCM, we take the highest power of each prime factor present in either number's factorization: 3, 5, and 13. Therefore, LCM(65, 15) = 3 x 5 x 13 = 195.
Modular Arithmetic
Modular arithmetic is a system of arithmetic for integers where numbers "wrap around" upon reaching a certain value, called the modulus. The concept of divisibility is central to modular arithmetic. For example, in modulo 5 arithmetic (where the numbers wrap around after reaching 5), 65 is equivalent to 0 because 65 leaves a remainder of 0 when divided by 5. This aligns with our conclusion that 65 is a multiple of 5.
Conclusion: The Significance of Simple Questions
The seemingly trivial question, "Is 65 a multiple of 5?", has provided a valuable opportunity to explore fundamental mathematical concepts – divisibility rules, prime factorization, multiples, factors, LCM, GCF, and even modular arithmetic. It highlights the interconnectedness of mathematical ideas and demonstrates how even simple problems can lead to deeper understanding. Mastering these foundational concepts lays the groundwork for more advanced mathematical explorations and problem-solving abilities. Remember, the seemingly simple questions often hold the key to unlocking a vast world of mathematical knowledge and application.
Latest Posts
Latest Posts
-
Examples Of Gay Lussacs Law In Real Life
May 12, 2025
-
The Answer Of Multiplication Is Called
May 12, 2025
-
How Many Ounces Are 500 Ml
May 12, 2025
-
5 Letter Words Ending In A N
May 12, 2025
-
How Many Sides And Corners Does A Triangle Have
May 12, 2025
Related Post
Thank you for visiting our website which covers about Is 65 A Multiple Of 5 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.