Is 5 7 A Rational Number
Juapaving
May 09, 2025 · 5 min read
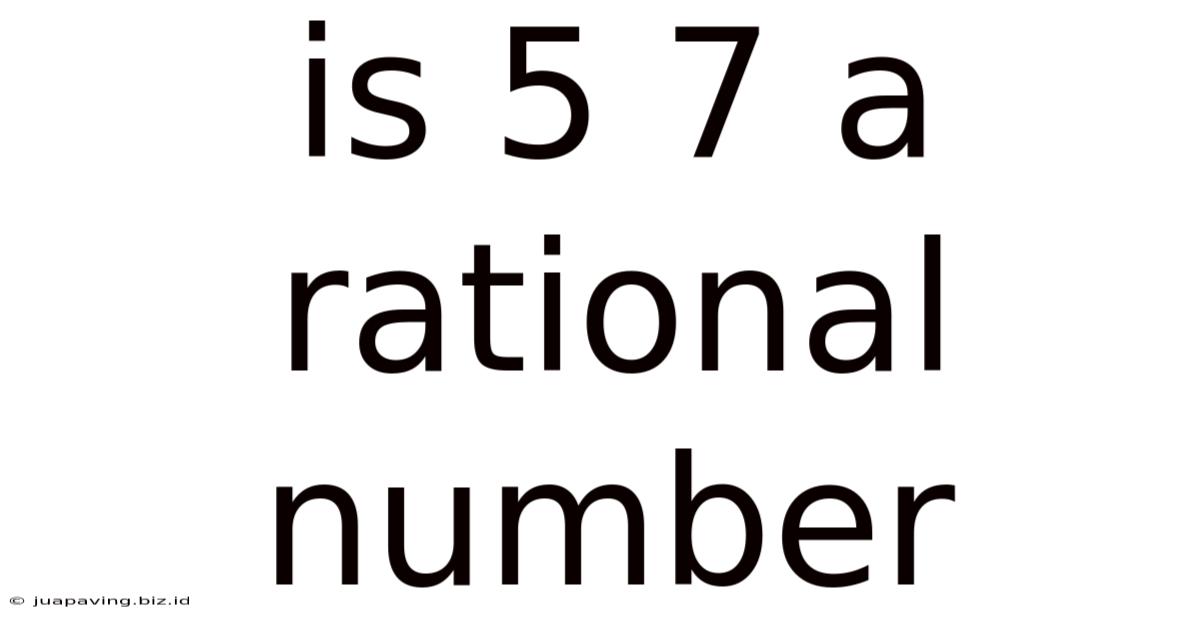
Table of Contents
Is 5/7 a Rational Number? A Deep Dive into Rational and Irrational Numbers
The question, "Is 5/7 a rational number?" seems deceptively simple. However, understanding the answer requires a deeper exploration of what constitutes a rational number and how it differs from its irrational counterpart. This article will not only definitively answer the question but also provide a comprehensive understanding of rational and irrational numbers, their properties, and how to identify them. We’ll delve into the historical context, explore various examples, and even touch upon the implications of these number types in advanced mathematics.
Understanding Rational Numbers
A rational number is any number that can be expressed as the quotient or fraction p/q of two integers, where p is the numerator and q is the non-zero denominator. The key here is the ability to represent the number as a simple fraction. This means that the decimal representation of a rational number will either terminate (end) or repeat in a predictable pattern.
Examples of Rational Numbers:
- 1/2: This is a classic example. It's a simple fraction, and its decimal equivalent is 0.5 (terminating).
- 3/4: Another straightforward example with a decimal equivalent of 0.75 (terminating).
- 2/3: This fraction yields a repeating decimal: 0.6666... (represented as 0.6̅).
- -5/8: Negative numbers can also be rational. This equals -0.625 (terminating).
- 7: Even whole numbers are rational! They can be expressed as 7/1.
- 0: Zero is rational; it can be expressed as 0/1.
The crucial point is that every rational number can be written as a fraction of two integers, and its decimal representation will either terminate or repeat.
Understanding Irrational Numbers
In contrast to rational numbers, irrational numbers cannot be expressed as a fraction of two integers. Their decimal representations are non-terminating and non-repeating. This means the digits go on forever without ever settling into a repeating pattern.
Examples of Irrational Numbers:
- π (Pi): The ratio of a circle's circumference to its diameter, approximately 3.1415926535..., is famously irrational. Its digits continue infinitely without repeating.
- √2 (Square root of 2): This number, approximately 1.41421356..., cannot be expressed as a fraction of two integers. Its decimal expansion is infinite and non-repeating.
- e (Euler's number): This fundamental constant in calculus, approximately 2.71828..., is another example of an irrational number.
- φ (Phi, the Golden Ratio): Approximately 1.618033..., this ratio appears frequently in nature and art, and it's also irrational.
Is 5/7 a Rational Number? The Definitive Answer
Given our definitions, the answer is a resounding yes. 5/7 is a rational number because:
- It's a fraction: It's expressed as the quotient of two integers: 5 (numerator) and 7 (denominator).
- Its decimal representation is either terminating or repeating: 5/7 equals approximately 0.714285714285..., which is a repeating decimal. The sequence "714285" repeats infinitely.
Therefore, 5/7 perfectly fits the definition of a rational number.
Further Exploring Rational and Irrational Numbers: Proofs and Implications
Let's delve deeper into some mathematical concepts related to rational and irrational numbers:
Proving Irrationality
Proving a number is irrational often involves proof by contradiction. A famous example is the proof that the square root of 2 is irrational. This proof assumes √2 is rational, expresses it as a fraction in its lowest terms, and then shows this leads to a contradiction, thus proving it must be irrational. Similar techniques can be applied to other numbers.
The Density of Rational and Irrational Numbers
Both rational and irrational numbers are dense on the real number line. This means that between any two distinct real numbers, you can always find both a rational and an irrational number. This might seem counterintuitive, given the seemingly "more numerous" irrational numbers, but it's a fundamental property of these number sets.
Real Numbers: The Union of Rational and Irrational Numbers
The set of real numbers encompasses all rational and irrational numbers. Every point on the number line represents a real number. Understanding the distinction between rational and irrational numbers is crucial for comprehending the structure and properties of the real number system.
Applications in Advanced Mathematics
Rational and irrational numbers play critical roles in various areas of advanced mathematics, including:
- Calculus: Understanding limits and continuity relies heavily on the properties of rational and irrational numbers.
- Number Theory: This branch of mathematics delves deeply into the properties of integers, including rational and irrational numbers derived from them.
- Algebra: Solving equations and working with algebraic structures often involves considering both rational and irrational solutions.
- Geometry: Irrational numbers, like π and √2, are fundamental in geometric calculations and formulas.
Conclusion: Mastering the Basics of Rational Numbers
Understanding the difference between rational and irrational numbers is fundamental to mathematical literacy. This article clarified that 5/7 is indeed a rational number due to its expressibility as a fraction of two integers and its repeating decimal representation. By exploring the properties, proofs, and applications of these number types, we've gained a more profound understanding of the rich tapestry of numbers that shape our mathematical world. Remember, the ability to identify and classify numbers as rational or irrational is a crucial skill for success in various mathematical fields. This knowledge serves as a solid foundation for exploring more advanced mathematical concepts and problem-solving. So, next time you encounter a number, take a moment to consider its classification – it might just unlock a deeper understanding of its properties and significance.
Latest Posts
Latest Posts
-
Difference Between Annual Biennial And Perennial Plants
May 09, 2025
-
The Unit Of Inductance Is The
May 09, 2025
-
Label The Parts Of The Female Reproductive System
May 09, 2025
-
Scientist That Proposed All Cells Come From Pre Exisiting Cells
May 09, 2025
-
What Prevents The Backflow Of Blood
May 09, 2025
Related Post
Thank you for visiting our website which covers about Is 5 7 A Rational Number . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.