Is 2 3 Bigger Than 1 2
Juapaving
May 14, 2025 · 5 min read
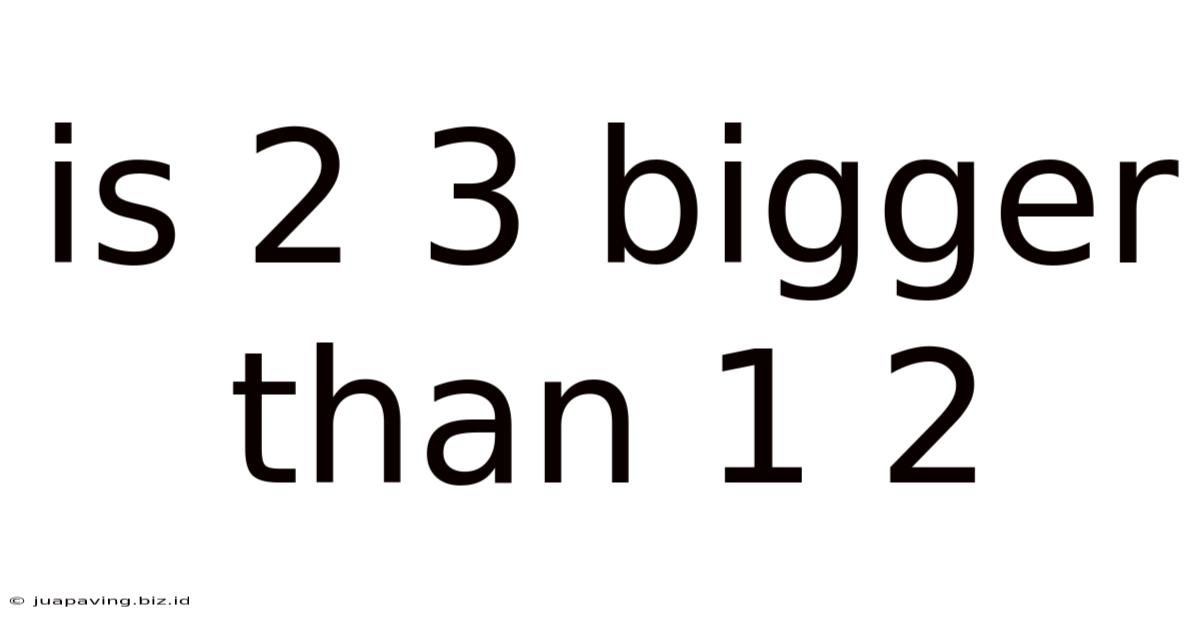
Table of Contents
Is 2/3 Bigger Than 1/2? A Deep Dive into Fraction Comparison
The question, "Is 2/3 bigger than 1/2?" might seem trivial at first glance. However, a thorough understanding of fraction comparison is crucial for various mathematical applications, from simple arithmetic to advanced calculus. This article will not only answer this specific question definitively but also explore the fundamental principles behind comparing fractions, providing you with the tools to confidently tackle similar problems in the future.
Understanding Fractions: A Refresher
Before diving into the comparison, let's ensure we have a solid grasp of what fractions represent. A fraction is a numerical representation of a part of a whole. It's expressed as a ratio of two numbers: the numerator (the top number) and the denominator (the bottom number). The numerator indicates the number of parts we have, while the denominator indicates the total number of equal parts the whole is divided into.
For example, in the fraction 2/3, the numerator is 2, and the denominator is 3. This means we have 2 parts out of a total of 3 equal parts.
Methods for Comparing Fractions
Several methods exist for comparing fractions. Let's examine the most common and effective ones:
1. Finding a Common Denominator
This is arguably the most straightforward and widely used method. The core idea is to convert both fractions to equivalent fractions with the same denominator. Once the denominators are identical, we can simply compare the numerators. The fraction with the larger numerator is the larger fraction.
Let's apply this to our question: Is 2/3 bigger than 1/2?
-
Find the Least Common Denominator (LCD): The LCD of 3 and 2 is 6.
-
Convert the fractions:
- 2/3 = (2 x 2) / (3 x 2) = 4/6
- 1/2 = (1 x 3) / (2 x 3) = 3/6
-
Compare the numerators: Since 4 > 3, we conclude that 4/6 > 3/6, meaning 2/3 > 1/2.
Therefore, yes, 2/3 is bigger than 1/2.
2. Visual Representation
A visual approach can be incredibly helpful, especially for beginners. Imagine two identical circles or rectangles.
- Represent 1/2: Divide one shape into two equal parts and shade one part.
- Represent 2/3: Divide the other shape into three equal parts and shade two parts.
By visually comparing the shaded areas, you'll readily observe that the shaded area representing 2/3 is larger than the shaded area representing 1/2. This provides a clear, intuitive understanding of the inequality.
3. Converting to Decimals
Another effective method involves converting fractions to their decimal equivalents. This is particularly useful when dealing with more complex fractions or when comparing fractions with different denominators.
- Convert 2/3 to a decimal: 2 ÷ 3 ≈ 0.6667
- Convert 1/2 to a decimal: 1 ÷ 2 = 0.5
Since 0.6667 > 0.5, we confirm that 2/3 > 1/2.
4. Cross-Multiplication
This method offers a quicker way to compare two fractions without finding a common denominator. Cross-multiply the numerators and denominators:
- Multiply the numerator of the first fraction by the denominator of the second fraction: 2 x 2 = 4
- Multiply the numerator of the second fraction by the denominator of the first fraction: 1 x 3 = 3
Compare the products: Since 4 > 3, we conclude that 2/3 > 1/2.
Extending the Concepts: More Complex Fraction Comparisons
The techniques discussed above provide a solid foundation for comparing any two fractions. Let's explore some more complex scenarios:
Scenario 1: Comparing Fractions with Larger Numerators and Denominators
Consider comparing 7/12 and 5/8. Using the common denominator method:
- The LCD of 12 and 8 is 24.
- 7/12 = 14/24
- 5/8 = 15/24
Since 15/24 > 14/24, we conclude that 5/8 > 7/12.
Scenario 2: Comparing Mixed Numbers
Mixed numbers combine a whole number and a fraction (e.g., 2 1/3). To compare mixed numbers, we can convert them into improper fractions (where the numerator is greater than or equal to the denominator) and then use any of the methods described earlier.
Scenario 3: Comparing Fractions with Negative Numbers
When comparing negative fractions, remember that the further a number is from zero on the number line, the smaller it is. For instance, -2/3 is smaller than -1/2 because -2/3 is further from zero.
Real-World Applications of Fraction Comparison
Understanding fraction comparison extends beyond the realm of theoretical mathematics; it finds practical application in various everyday situations:
-
Baking and Cooking: Recipes often require precise measurements using fractions. Accurately comparing fractions is vital for ensuring the final product is consistent and delicious.
-
Construction and Engineering: Precise measurements are critical in these fields. Comparing fractions allows for accurate calculations and the creation of structurally sound buildings and other structures.
-
Finance: Understanding fractions is essential for managing budgets, calculating interest rates, and comprehending financial reports.
-
Data Analysis: Fractions are frequently used to represent proportions and percentages in data analysis. The ability to compare fractions effectively enables meaningful interpretation of data.
Conclusion: Mastering Fraction Comparison
The ability to confidently compare fractions is a fundamental mathematical skill with wide-ranging applications. By mastering the techniques outlined in this article – finding common denominators, visual representation, decimal conversion, and cross-multiplication – you'll be equipped to tackle a wide variety of fraction comparison problems with ease and accuracy. Remember that understanding the underlying principles is key to applying these methods effectively and developing a strong intuitive understanding of fraction magnitude. Practice regularly, and you'll soon find comparing fractions to be an effortless task.
Latest Posts
Latest Posts
-
5 3 And A Half In Cm
May 14, 2025
-
How Is Buoyancy And Density Related
May 14, 2025
-
Is Heat Capacity Intensive Or Extensive
May 14, 2025
-
What Is The Difference Between Renewable And Non Renewable Energy
May 14, 2025
-
How Many Electrons Does Potassium Have
May 14, 2025
Related Post
Thank you for visiting our website which covers about Is 2 3 Bigger Than 1 2 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.