Is 1 2 Bigger Than 2 5
Juapaving
Apr 14, 2025 · 4 min read
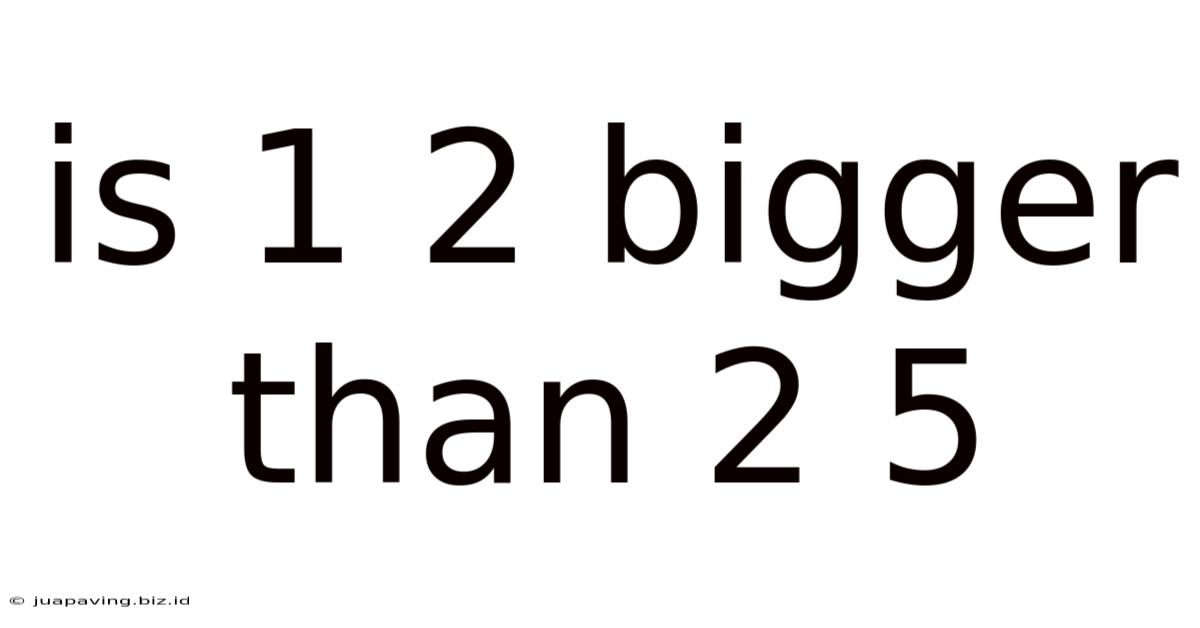
Table of Contents
Is 1/2 Bigger Than 2/5? A Deep Dive into Fraction Comparison
Determining whether 1/2 is bigger than 2/5 might seem like a simple question, but it opens the door to understanding fundamental concepts in mathematics, particularly fraction comparison. This article will explore various methods to compare these fractions, delve into the underlying mathematical principles, and offer practical applications to solidify your understanding. We'll also touch upon the broader implications of fraction comparison in everyday life and advanced mathematical contexts.
Understanding Fractions: A Refresher
Before we directly compare 1/2 and 2/5, let's refresh our understanding of fractions. A fraction represents a part of a whole. It's composed of two key components:
- Numerator: The top number, indicating the number of parts we have.
- Denominator: The bottom number, indicating the total number of equal parts the whole is divided into.
For example, in the fraction 1/2, the numerator (1) signifies that we have one part, and the denominator (2) signifies that the whole is divided into two equal parts.
Method 1: Finding a Common Denominator
One of the most common and reliable methods for comparing fractions is to find a common denominator. This involves converting both fractions so they share the same denominator, allowing for a direct comparison of their numerators.
To compare 1/2 and 2/5, we need to find the least common multiple (LCM) of the denominators, 2 and 5. The LCM of 2 and 5 is 10.
Now, we convert each fraction to have a denominator of 10:
- 1/2: To get a denominator of 10, we multiply both the numerator and the denominator by 5: (1 * 5) / (2 * 5) = 5/10
- 2/5: To get a denominator of 10, we multiply both the numerator and the denominator by 2: (2 * 2) / (5 * 2) = 4/10
Now the comparison is straightforward: 5/10 > 4/10. Therefore, 1/2 is bigger than 2/5.
Method 2: Converting to Decimals
Another effective approach involves converting the fractions into decimals. This method is particularly useful when dealing with more complex fractions or when you're comfortable with decimal comparisons.
To convert a fraction to a decimal, simply divide the numerator by the denominator:
- 1/2 = 0.5
- 2/5 = 0.4
Clearly, 0.5 > 0.4. Again, this confirms that 1/2 is bigger than 2/5.
Method 3: Visual Representation
Visual aids can be incredibly helpful, especially when explaining fraction comparison to younger learners or those who benefit from visual representations. Imagine two identical pizzas:
- Pizza 1: Cut into two equal slices. 1/2 represents one of those slices.
- Pizza 2: Cut into five equal slices. 2/5 represents two of those slices.
By visually comparing the size of one slice from Pizza 1 to two slices from Pizza 2, you can intuitively see that the single slice from the pizza cut in half (1/2) is larger. This confirms that 1/2 is bigger than 2/5.
Method 4: Cross-Multiplication
Cross-multiplication provides a quick and efficient method for comparing fractions. This involves multiplying the numerator of one fraction by the denominator of the other, and vice-versa. The larger product indicates the larger fraction.
Let's compare 1/2 and 2/5:
- Multiply 1 (numerator of 1/2) by 5 (denominator of 2/5): 1 * 5 = 5
- Multiply 2 (numerator of 2/5) by 2 (denominator of 1/2): 2 * 2 = 4
Since 5 > 4, 1/2 is bigger than 2/5.
Beyond the Basics: Applications and Extensions
The ability to compare fractions is far more than a simple mathematical exercise. It has far-reaching applications in various fields:
-
Everyday Life: From cooking (measuring ingredients) to budgeting (managing finances), comparing fractions is an integral part of daily tasks. Determining whether you have enough material for a project or comparing prices often requires this skill.
-
Science and Engineering: In fields like physics and engineering, fraction comparison is crucial for accurate calculations and measurements. Understanding ratios and proportions necessitates a solid grasp of fraction comparison.
-
Advanced Mathematics: Fractions form the foundation of more complex mathematical concepts like algebra, calculus, and even advanced topics in number theory. A firm understanding of basic fraction comparison is essential for progressing to these higher levels.
Addressing Potential Misconceptions
Some common misconceptions arise when working with fractions:
-
Larger Numerator Doesn't Always Mean a Larger Fraction: The size of the fraction is determined by both the numerator and denominator. A larger numerator does not automatically mean a larger fraction if the denominator is also significantly larger.
-
Focusing Solely on Numerators or Denominators: Comparing fractions solely based on either the numerator or the denominator without considering the other component will often lead to incorrect conclusions.
Conclusion: Mastering Fraction Comparison
Comparing fractions like 1/2 and 2/5 is a fundamental skill with wide-ranging applications. Whether you utilize the common denominator method, decimal conversion, visual representation, or cross-multiplication, mastering these techniques ensures accuracy and confidence in mathematical operations. Understanding the underlying principles helps to build a strong foundation for more advanced mathematical concepts and real-world problem-solving. Remember to practice regularly and explore different methods to reinforce your understanding and find the approach that best suits your learning style. The ability to confidently compare fractions is a key element of mathematical proficiency and a valuable skill for navigating numerous aspects of life.
Latest Posts
Latest Posts
-
Corresponding Parts Of Congruent Triangles Are Congruent
May 09, 2025
-
Greatest Common Factor Of 12 And 36
May 09, 2025
-
Burning A Chemical Or Physical Change
May 09, 2025
-
Aluminium Is A Good Conductor Of Electricity
May 09, 2025
-
Is 4 A Prime Number Or A Composite Number
May 09, 2025
Related Post
Thank you for visiting our website which covers about Is 1 2 Bigger Than 2 5 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.