How Many Lines Of Symmetry Does A Scalene Triangle Have
Juapaving
May 09, 2025 · 5 min read
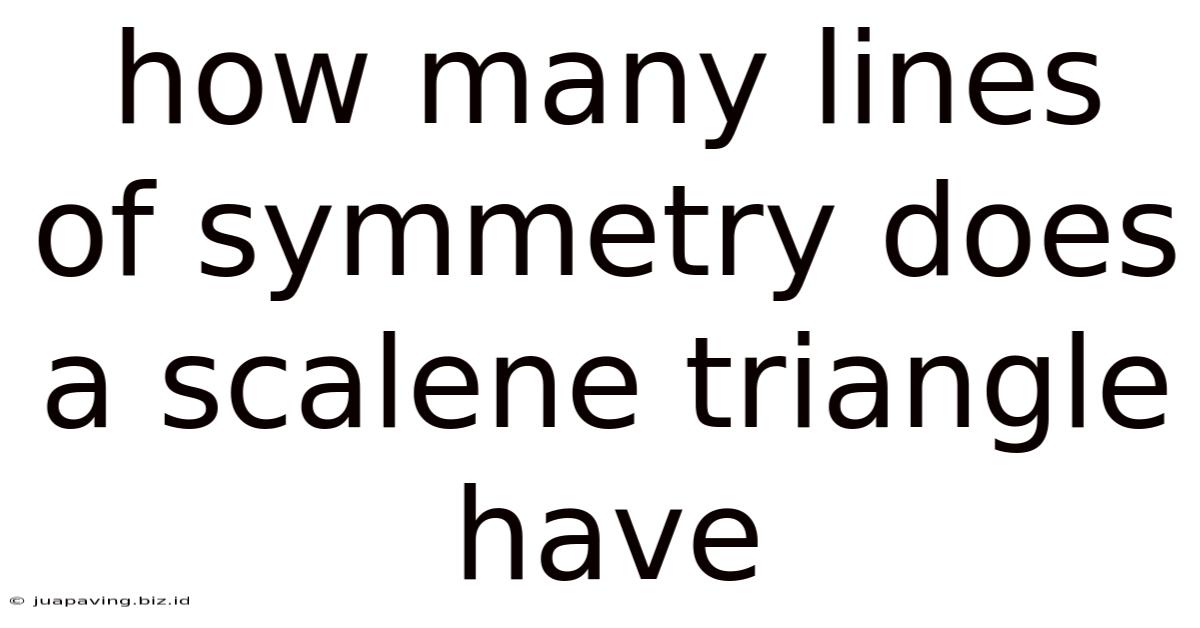
Table of Contents
How Many Lines of Symmetry Does a Scalene Triangle Have? Exploring Geometric Properties
Understanding lines of symmetry is fundamental to grasping geometric concepts. This article delves into the fascinating world of symmetry, focusing specifically on scalene triangles and their unique properties. We will explore what constitutes a line of symmetry, define a scalene triangle, and definitively answer the question: how many lines of symmetry does a scalene triangle possess? We'll also touch upon related concepts like isosceles and equilateral triangles to provide a comprehensive understanding.
Defining Symmetry and Lines of Symmetry
Symmetry, in simple terms, refers to a balanced and proportionate arrangement of parts. In geometry, we focus on line symmetry, also known as reflectional symmetry. A line of symmetry divides a shape into two identical halves, mirroring each other perfectly. If you were to fold the shape along the line of symmetry, both halves would completely overlap.
Identifying Lines of Symmetry
Identifying lines of symmetry involves visualizing the shape and determining if a line can be drawn that divides it into two congruent (identical in shape and size) parts. This line acts as a "mirror," with each point on one side having a corresponding point on the other side at an equal distance from the line.
Understanding Different Types of Triangles
Triangles are classified based on the lengths of their sides and the measures of their angles. The three main types are:
1. Equilateral Triangles
An equilateral triangle has three sides of equal length and three angles of equal measure (60° each). It possesses three lines of symmetry, each passing through a vertex and the midpoint of the opposite side.
2. Isosceles Triangles
An isosceles triangle has two sides of equal length and two angles of equal measure. It has one line of symmetry, which bisects the unequal side and passes through the vertex formed by the two equal sides.
3. Scalene Triangles
A scalene triangle is characterized by having three sides of unequal lengths and three angles of unequal measures. This is where the core of our discussion lies.
How Many Lines of Symmetry Does a Scalene Triangle Have?
The definitive answer is: A scalene triangle has zero lines of symmetry.
This is because there is no line that can divide a scalene triangle into two identical halves. Since all three sides are different lengths, and all three angles are different measures, no line can be drawn to create a perfect mirror image. Any attempt to draw a line will always result in two unequal halves.
Visualizing the Absence of Symmetry in a Scalene Triangle
Imagine trying to fold a scalene triangle in half. No matter how you attempt to fold it, the two halves will never perfectly overlap. This visual representation clearly demonstrates the absence of any lines of symmetry.
Comparing Symmetry in Different Triangle Types
The number of lines of symmetry directly relates to the type of triangle:
- Equilateral Triangle: 3 lines of symmetry
- Isosceles Triangle: 1 line of symmetry
- Scalene Triangle: 0 lines of symmetry
This comparison highlights the relationship between side lengths, angle measures, and the presence or absence of lines of symmetry.
Exploring Rotational Symmetry (Optional Extension)
While scalene triangles lack line symmetry, it's worth briefly touching upon rotational symmetry. Rotational symmetry refers to a shape's ability to be rotated around a central point and still look the same. Even though a scalene triangle doesn't exhibit line symmetry, it also doesn't have rotational symmetry unless it's a special case that approaches an isosceles triangle. A scalene triangle only exhibits rotational symmetry of order 1, meaning it has to be rotated 360° to appear identical to its original form.
Practical Applications and Real-World Examples
Understanding lines of symmetry and the properties of different types of triangles has various practical applications in diverse fields:
- Design and Art: Symmetry plays a crucial role in creating aesthetically pleasing designs in architecture, graphic design, and art. Understanding the lack of symmetry in scalene triangles can inform creative choices, leading to unique and dynamic designs.
- Engineering and Construction: Symmetrical structures often possess greater stability and strength. Understanding the lack of inherent symmetry in scalene triangles can be significant in structural engineering design, requiring careful considerations for balance and support.
- Mathematics and Geometry: The study of symmetry forms the basis for various mathematical concepts and theories. Exploring the properties of different types of triangles provides valuable insight into geometric principles.
Advanced Concepts and Further Exploration
For those interested in delving deeper, exploring concepts like:
- Congruence: Understanding how congruent figures relate to symmetry.
- Transformations: Investigating different types of geometric transformations such as reflections and rotations.
- Advanced Geometry: Studying more complex geometric shapes and their symmetry properties.
would provide a more complete understanding of this fascinating area of mathematics.
Conclusion
In conclusion, a scalene triangle, with its three unequal sides and angles, does not possess any lines of symmetry. This fundamental property distinguishes it from equilateral and isosceles triangles. Understanding this concept is essential for grasping fundamental geometric principles and their applications in various fields. The absence of symmetry in a scalene triangle does not diminish its significance; rather, it highlights its unique geometric characteristics. This exploration serves as a stepping stone for further investigation into the captivating world of geometry and its vast applications. The beauty of mathematics lies in its ability to reveal patterns and properties even in seemingly simple shapes like the scalene triangle.
Latest Posts
Latest Posts
-
What Muscle Separates The Thoracic Cavity From The Abdominal Cavity
May 09, 2025
-
A Group Of Tissues Working Together
May 09, 2025
-
What Part Of A Plant Makes The Food
May 09, 2025
-
What Is The Basic Unit Of All Living Things
May 09, 2025
-
A Solid Ab Has Zns Type Structure
May 09, 2025
Related Post
Thank you for visiting our website which covers about How Many Lines Of Symmetry Does A Scalene Triangle Have . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.