How Do You Do Expanded Notation
Juapaving
Mar 30, 2025 · 6 min read
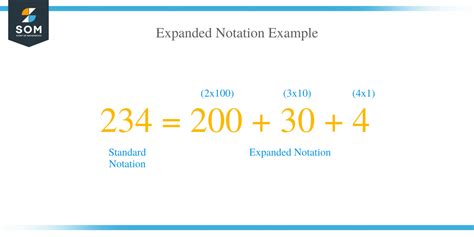
Table of Contents
How to Do Expanded Notation: A Comprehensive Guide
Expanded notation is a fundamental concept in mathematics that helps students understand the place value of digits within a number. It's a crucial stepping stone to mastering more complex mathematical operations and grasping the structure of our number system. This comprehensive guide will explore expanded notation in detail, covering its various forms, applications, and how to use it effectively. We'll delve into examples, tackle common misconceptions, and provide practical tips to help you master this important skill.
What is Expanded Notation?
Expanded notation is a way of writing a number to show the value of each digit. Instead of representing a number in its standard form (e.g., 123), we break it down to show the value of each digit based on its position (ones, tens, hundreds, thousands, etc.). This clearly demonstrates the concept of place value, which is the foundation of our base-10 number system.
Understanding Place Value
Before diving into expanded notation, let's solidify our understanding of place value. In our base-10 system, each position in a number represents a power of 10:
- Ones: 10⁰ = 1
- Tens: 10¹ = 10
- Hundreds: 10² = 100
- Thousands: 10³ = 1000
- Ten Thousands: 10⁴ = 10,000
- And so on...
Each digit in a number occupies a specific place value, contributing to the overall value of the number. For example, in the number 345, the digit 3 represents 3 hundreds (300), the digit 4 represents 4 tens (40), and the digit 5 represents 5 ones (5).
Different Forms of Expanded Notation
There are primarily two ways to represent expanded notation:
1. Additive Expanded Notation
This is the most common form of expanded notation. It expresses a number as the sum of the values of its digits. For example:
- 123: 100 + 20 + 3
- 4,567: 4000 + 500 + 60 + 7
- 23,456: 20000 + 3000 + 400 + 50 + 6
- 123,456,789: 100,000,000 + 20,000,000 + 3,000,000 + 400,000 + 50,000 + 6,000 + 700 + 80 + 9
This clearly shows the contribution of each digit to the total value of the number.
2. Multiplicative Expanded Notation
This form uses powers of 10 to represent the place value of each digit. This is particularly useful for understanding the relationship between place value and exponents. Let's revisit the examples using this method:
- 123: (1 x 10²) + (2 x 10¹) + (3 x 10⁰)
- 4,567: (4 x 10³) + (5 x 10²) + (6 x 10¹) + (7 x 10⁰)
- 23,456: (2 x 10⁴) + (3 x 10³) + (4 x 10²) + (5 x 10¹) + (6 x 10⁰)
- 123,456,789: (1 x 10⁸) + (2 x 10⁷) + (3 x 10⁶) + (4 x 10⁵) + (5 x 10⁴) + (6 x 10³) + (7 x 10²) + (8 x 10¹) + (9 x 10⁰)
This method explicitly shows the place value of each digit as a power of 10, reinforcing the concept of the base-10 number system.
Working with Expanded Notation: Practical Examples
Let's work through some examples to further solidify your understanding:
Example 1: Write 7,895 in expanded notation.
Additive: 7000 + 800 + 90 + 5
Multiplicative: (7 x 10³) + (8 x 10²) + (9 x 10¹) + (5 x 10⁰)
Example 2: Write the number represented by (3 x 10⁴) + (2 x 10²) + (5 x 10¹) + (1 x 10⁰) in standard form.
This represents: 30,000 + 200 + 50 + 1 = 30,251
Example 3: A slightly more challenging example: Write 1,002,005 in expanded notation. Notice that we have zeros as place holders.
Additive: 1,000,000 + 200 + 5
Multiplicative: (1 x 10⁶) + (2 x 10²) + (5 x 10⁰)
This highlights that even zeros play a significant role in maintaining the place value of other digits.
Decimals and Expanded Notation
Expanded notation extends seamlessly to decimal numbers. We simply continue the pattern of place values beyond the decimal point, using negative powers of 10:
- Tenths: 10⁻¹ = 0.1
- Hundredths: 10⁻² = 0.01
- Thousandths: 10⁻³ = 0.001
- And so on...
Example 4: Write 3.45 in expanded notation.
Additive: 3 + 0.4 + 0.05
Multiplicative: (3 x 10⁰) + (4 x 10⁻¹) + (5 x 10⁻²)
Example 5: Write 12.345 in expanded notation.
Additive: 10 + 2 + 0.3 + 0.04 + 0.005
Multiplicative: (1 x 10¹) + (2 x 10⁰) + (3 x 10⁻¹) + (4 x 10⁻²) + (5 x 10⁻³)
Applications of Expanded Notation
Expanded notation isn't just an abstract mathematical concept; it has several practical applications:
-
Place Value Understanding: It's fundamental for grasping the concept of place value and how digits contribute to the overall value of a number. This is essential for building a strong foundation in arithmetic.
-
Addition and Subtraction: Breaking numbers down into expanded notation can simplify addition and subtraction, particularly for larger numbers. It allows students to add or subtract like place values separately before combining the results.
-
Multiplication and Division: Expanded notation can aid in understanding the distributive property of multiplication, allowing for easier multiplication of larger numbers. Similarly, it can aid in visualizing division by breaking the dividend into smaller, manageable parts.
-
Understanding Number Systems: It provides a solid base for understanding different number systems, including binary, hexadecimal, and other bases. The principles remain the same, only the base changes.
-
Estimation and Rounding: Expanded notation helps in estimating and rounding numbers, as it provides a clear view of the value of each digit and makes identifying significant digits simpler.
Common Misconceptions and How to Avoid Them
-
Confusing expanded notation with standard form: Students might mistakenly think that writing a number in words (e.g., one hundred twenty-three) is expanded notation. Remember, expanded notation shows the numerical value of each digit.
-
Incorrect use of place value: Students may misplace the value of digits, particularly when dealing with larger numbers or decimals. Careful attention to place value is crucial.
-
Skipping zeros: Failing to include zeros as place holders can lead to incorrect expanded notation. Remember, zeros hold significant places within a number.
To avoid these misconceptions, practice regularly with diverse examples, including larger numbers and decimal numbers. Use visual aids like place value charts to reinforce understanding.
Tips for Mastering Expanded Notation
-
Practice consistently: The more you practice, the more comfortable you will become with writing numbers in expanded notation and vice versa.
-
Use visual aids: Place value charts and manipulatives can be incredibly helpful in visualizing place value and understanding expanded notation.
-
Start with smaller numbers: Begin with smaller numbers before moving on to larger numbers and decimals. This builds confidence and understanding gradually.
-
Break it down: When working with larger numbers, break them into smaller parts and apply expanded notation to each part before combining the results.
-
Seek help when needed: If you are struggling with any aspect of expanded notation, don't hesitate to seek help from a teacher, tutor, or online resources.
Conclusion
Expanded notation is a powerful tool for understanding the structure and value of numbers. By mastering this concept, you lay a strong foundation for future mathematical success. Remember the different forms of expanded notation, practice consistently, and use various examples to build your proficiency. With dedication and practice, expanded notation will become a simple and intuitive process. The key is understanding place value and consistently applying the concepts outlined in this guide. Remember to break down complex numbers into smaller, manageable parts to avoid errors and build your confidence.
Latest Posts
Latest Posts
-
The Planning Process Begins With Which Of These
Apr 01, 2025
-
Which Figure Has Exactly Four Lines Of Symmetry
Apr 01, 2025
-
All Important Equations Of Chemistry Class 10
Apr 01, 2025
-
What Is The Least Common Multiple Of 9 And 27
Apr 01, 2025
-
The Innermost Layer Of The Root Cortex Is The
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about How Do You Do Expanded Notation . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
