Evaluate The Trigonometric Function At The Quadrantal Angle
Juapaving
Apr 04, 2025 · 5 min read
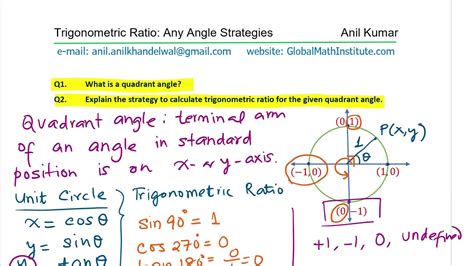
Table of Contents
Evaluating Trigonometric Functions at Quadrantal Angles: A Comprehensive Guide
Evaluating trigonometric functions at quadrantal angles is a fundamental concept in trigonometry. Understanding this process is crucial for mastering more advanced trigonometric topics and applications in calculus, physics, and engineering. This comprehensive guide will delve deep into the evaluation of sine, cosine, tangent, cotangent, secant, and cosecant functions at these specific angles, providing a clear and concise explanation supported by diagrams and examples.
Understanding Quadrantal Angles
Quadrantal angles are angles whose terminal side lies on one of the axes (x-axis or y-axis) when the angle is placed in standard position. These angles are multiples of 90 degrees (π/2 radians). The four principal quadrantal angles are:
- 0° (0 radians): Lies on the positive x-axis.
- 90° (π/2 radians): Lies on the positive y-axis.
- 180° (π radians): Lies on the negative x-axis.
- 270° (3π/2 radians): Lies on the negative y-axis.
- 360° (2π radians): Lies on the positive x-axis (coterminal with 0°).
Any angle that is a multiple of 90° will also be a quadrantal angle. For example, 450° (5π/2 radians) is a quadrantal angle because it's coterminal with 90°.
Evaluating Trigonometric Functions at Quadrantal Angles
To evaluate trigonometric functions at quadrantal angles, we use the unit circle. The unit circle is a circle with a radius of 1 centered at the origin (0,0) of a coordinate plane. Any point (x, y) on the unit circle can be represented by the coordinates (cos θ, sin θ), where θ is the angle formed by the positive x-axis and the line segment connecting the origin to the point (x, y).
Let's evaluate the six trigonometric functions for each of the principal quadrantal angles:
0° (0 radians)
- sin 0° = 0: The y-coordinate of the point on the unit circle at 0° is 0.
- cos 0° = 1: The x-coordinate of the point on the unit circle at 0° is 1.
- tan 0° = 0: tan θ = sin θ / cos θ = 0/1 = 0
- cot 0° = undefined: cot θ = cos θ / sin θ = 1/0 (division by zero is undefined)
- sec 0° = 1: sec θ = 1/cos θ = 1/1 = 1
- csc 0° = undefined: csc θ = 1/sin θ = 1/0 (division by zero is undefined)
90° (π/2 radians)
- sin 90° = 1: The y-coordinate of the point on the unit circle at 90° is 1.
- cos 90° = 0: The x-coordinate of the point on the unit circle at 90° is 0.
- tan 90° = undefined: tan θ = sin θ / cos θ = 1/0 (division by zero is undefined)
- cot 90° = 0: cot θ = cos θ / sin θ = 0/1 = 0
- sec 90° = undefined: sec θ = 1/cos θ = 1/0 (division by zero is undefined)
- csc 90° = 1: csc θ = 1/sin θ = 1/1 = 1
180° (π radians)
- sin 180° = 0: The y-coordinate of the point on the unit circle at 180° is 0.
- cos 180° = -1: The x-coordinate of the point on the unit circle at 180° is -1.
- tan 180° = 0: tan θ = sin θ / cos θ = 0/(-1) = 0
- cot 180° = undefined: cot θ = cos θ / sin θ = -1/0 (division by zero is undefined)
- sec 180° = -1: sec θ = 1/cos θ = 1/(-1) = -1
- csc 180° = undefined: csc θ = 1/sin θ = 1/0 (division by zero is undefined)
270° (3π/2 radians)
- sin 270° = -1: The y-coordinate of the point on the unit circle at 270° is -1.
- cos 270° = 0: The x-coordinate of the point on the unit circle at 270° is 0.
- tan 270° = undefined: tan θ = sin θ / cos θ = -1/0 (division by zero is undefined)
- cot 270° = 0: cot θ = cos θ / sin θ = 0/(-1) = 0
- sec 270° = undefined: sec θ = 1/cos θ = 1/0 (division by zero is undefined)
- csc 270° = -1: csc θ = 1/sin θ = 1/(-1) = -1
360° (2π radians)
The values at 360° are identical to those at 0° because they are coterminal angles.
Visualizing with the Unit Circle
The unit circle provides a powerful visual aid for understanding these values. Imagine a point moving along the circumference of the unit circle. As the point moves, its x and y coordinates directly represent the cosine and sine of the angle, respectively. When the point lies on an axis, one coordinate is 0, and the other is either 1 or -1, leading to the values we've derived above.
Using Reference Angles
For angles beyond the principal quadrantal angles, we can use reference angles to determine the values of trigonometric functions. The reference angle is the acute angle formed between the terminal side of the angle and the x-axis. The sign of the trigonometric function is determined by the quadrant in which the angle lies. For example:
- 450°: This is coterminal with 90°, so its trigonometric function values are the same as 90°.
- -90°: This is coterminal with 270°, so its trigonometric function values are the same as 270°.
- 540°: This is coterminal with 180°, so its trigonometric function values are the same as 180°.
Applications and Importance
Understanding the evaluation of trigonometric functions at quadrantal angles is fundamental to:
- Graphing Trigonometric Functions: These angles represent key points on the graphs of sine, cosine, and tangent functions, helping to understand their periodicity and amplitude.
- Solving Trigonometric Equations: Many trigonometric equations involve quadrantal angles as solutions.
- Calculus: Understanding these values is essential for evaluating limits and derivatives of trigonometric functions.
- Physics and Engineering: Applications in fields like wave mechanics, oscillatory motion, and signal processing frequently utilize quadrantal angles.
Common Mistakes and How to Avoid Them
A common mistake is confusing the signs of the trigonometric functions in different quadrants. Remember the mnemonic "All Students Take Calculus" to remember the positive functions in each quadrant:
- Quadrant I (0° - 90°): All functions are positive.
- Quadrant II (90° - 180°): Sine is positive.
- Quadrant III (180° - 270°): Tangent is positive.
- Quadrant IV (270° - 360°): Cosine is positive.
Another mistake is forgetting that division by zero is undefined. Always check the denominator when calculating tangent, cotangent, secant, and cosecant.
Conclusion
Mastering the evaluation of trigonometric functions at quadrantal angles is a cornerstone of trigonometry. By understanding the unit circle, reference angles, and the signs of trigonometric functions in different quadrants, you can confidently evaluate these functions and apply this knowledge to various mathematical and real-world problems. Consistent practice and visualization using the unit circle are crucial for developing a strong grasp of this essential concept. Remember to always double-check your work and be mindful of potential pitfalls, such as division by zero. With diligent effort, you can achieve a thorough understanding of this fundamental area of trigonometry.
Latest Posts
Latest Posts
-
The Primary Function Of The Excretory System Is To
Apr 10, 2025
-
Isosceles Triangle With A Right Angle
Apr 10, 2025
-
What Is The I U P A C Name Of The Following Compound
Apr 10, 2025
-
What Is 15 In A Decimal
Apr 10, 2025
-
Which Of The Following Is True For Displacement
Apr 10, 2025
Related Post
Thank you for visiting our website which covers about Evaluate The Trigonometric Function At The Quadrantal Angle . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.