Coefficient Of 𝑥 𝑚 X M
Juapaving
Apr 05, 2025 · 6 min read
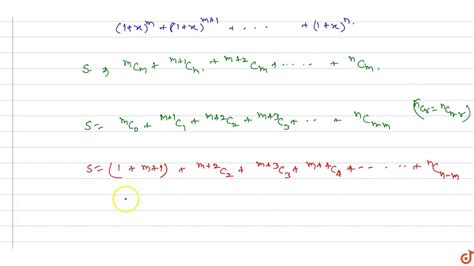
Table of Contents
Understanding the Coefficient of x<sup>m</sup>: A Comprehensive Guide
The coefficient of x<sup>m</sup>, a seemingly simple concept in algebra, underlies a vast array of mathematical applications. Understanding how to identify, calculate, and utilize these coefficients is crucial for success in various fields, from calculus and probability to computer science and physics. This comprehensive guide will delve deep into the intricacies of the coefficient of x<sup>m</sup>, exploring its definition, calculation methods, and its significance in different contexts.
What is the Coefficient of x<sup>m</sup>?
In mathematics, particularly in algebra and calculus, a polynomial is an expression consisting of variables and coefficients, involving only the operations of addition, subtraction, multiplication, and non-negative integer exponents of variables. A general polynomial in a single variable x can be represented as:
a<sub>n</sub>x<sup>n</sup> + a<sub>n-1</sub>x<sup>n-1</sup> + ... + a<sub>2</sub>x<sup>2</sup> + a<sub>1</sub>x<sup>1</sup> + a<sub>0</sub>
where:
- a<sub>i</sub> represents the coefficients, which are constants (real numbers or complex numbers).
- x is the variable.
- i represents the exponents, which are non-negative integers (0, 1, 2, ..., n).
The coefficient of x<sup>m</sup> is simply the constant, a<sub>m</sub>, that multiplies the term x<sup>m</sup> in the polynomial. If the term x<sup>m</sup> is absent from the polynomial, then the coefficient of x<sup>m</sup> is considered to be 0.
For example, consider the polynomial:
3x<sup>4</sup> + 2x<sup>3</sup> - 5x<sup>2</sup> + 7x + 1
- The coefficient of x<sup>4</sup> is 3.
- The coefficient of x<sup>3</sup> is 2.
- The coefficient of x<sup>2</sup> is -5.
- The coefficient of x<sup>1</sup> (or simply x) is 7.
- The coefficient of x<sup>0</sup> (which is equivalent to the constant term) is 1.
- The coefficient of x<sup>5</sup> is 0, as the term x<sup>5</sup> is absent.
Methods for Calculating the Coefficient of x<sup>m</sup>
The methods for calculating the coefficient of x<sup>m</sup> depend heavily on the context in which the polynomial arises. Let's explore several common scenarios:
1. Direct Identification from Expanded Polynomials:
This is the simplest case. If the polynomial is already expanded, the coefficient of x<sup>m</sup> can be identified directly by inspecting the term containing x<sup>m</sup>. For example, in the polynomial 2x<sup>5</sup> + 9x<sup>3</sup> – 4x + 1, the coefficient of x<sup>3</sup> is 9.
2. Expanding Binomial Expressions:
Binomial expressions of the form (a + b)<sup>n</sup> frequently appear. The coefficients of the terms in the expansion are given by the binomial theorem:
(a + b)<sup>n</sup> = Σ [n! / (k!(n-k)!)] * a<sup>n-k</sup> * b<sup>k</sup> where k ranges from 0 to n.
This formula generates the binomial coefficients, often represented as "n choose k," which are the entries in Pascal's triangle. To find the coefficient of x<sup>m</sup> in a binomial expansion, you need to determine the appropriate value of k that satisfies the exponent condition in the binomial theorem. Finding the correct term requires careful examination of the exponents of 'a' and 'b' to match the desired power of x.
3. Using the Multinomial Theorem:
For expressions involving more than two terms, the multinomial theorem generalizes the binomial theorem. The coefficients become significantly more complex, involving multinomial coefficients, and determining the coefficient of x<sup>m</sup> requires carefully considering all possible combinations of terms that yield x<sup>m</sup>. This often necessitates meticulous accounting for all potential exponent combinations and their respective multinomial coefficients.
4. Utilizing Generating Functions:
Generating functions provide a powerful tool for determining coefficients. A generating function represents a sequence of numbers as the coefficients of a power series. By manipulating the generating function and extracting the coefficient of x<sup>m</sup>, one can determine the desired coefficient. This method, while more abstract, can be highly effective, particularly for complex sequences or recursions where direct calculation becomes challenging.
5. Calculus Techniques:
For certain scenarios, calculus tools can assist. For instance, Taylor series expansions express functions as infinite power series. The coefficient of x<sup>m</sup> in the Taylor expansion of a function directly corresponds to a derivative of that function evaluated at a specific point (usually 0). This approach requires a good understanding of calculus and function analysis.
Applications of the Coefficient of x<sup>m</sup>
The coefficient of x<sup>m</sup> has far-reaching applications across numerous fields. Here are some prominent examples:
1. Probability and Statistics:
Binomial coefficients are fundamental in probability theory, particularly in calculating probabilities involving binomial distributions. The coefficient of x<sup>m</sup> in the expansion of (p + q)<sup>n</sup> (where p + q = 1) represents the probability of exactly m successes in n independent Bernoulli trials.
2. Combinatorics:
Combinatorial problems often involve counting the number of ways to arrange or select items. Binomial and multinomial coefficients directly relate to the solutions of many combinatorial problems. For instance, the number of ways to choose m items from a set of n items is given by the binomial coefficient "n choose m".
3. Numerical Analysis:
In numerical analysis, the coefficients of x<sup>m</sup> in approximating polynomials (such as Taylor or Chebyshev polynomials) play a crucial role in determining the accuracy and stability of numerical algorithms.
4. Computer Science:
Generating functions and recursive relations, intimately related to the concept of coefficients, are critical tools in the analysis of algorithms and data structures. Determining the computational complexity of algorithms often involves analyzing coefficients in generating functions that represent recurrence relations.
5. Physics:
In quantum mechanics, the coefficients of power series expansions of wave functions are important in characterizing physical systems. Many physical laws are represented using power series. The coefficients play a role in describing the strength or influence of specific physical properties.
Advanced Techniques and Considerations:
For more complex scenarios, several advanced techniques may be necessary. These include:
- Partial Fraction Decomposition: For rational functions, this technique simplifies expressions by decomposing them into simpler fractions, making coefficient identification easier.
- Residue Theorem (Complex Analysis): This powerful theorem from complex analysis provides a method for efficiently calculating coefficients of power series.
- Symbolic Computation Software: Software packages such as Mathematica, Maple, or SageMath can automate the calculation of coefficients for complex polynomials.
Conclusion:
The seemingly simple concept of the coefficient of x<sup>m</sup> underpins a vast landscape of mathematical applications. Understanding its different calculation methods and its significance in various contexts, from elementary algebra to advanced calculus and beyond, is essential for anyone pursuing studies or careers in mathematics, science, engineering, or computer science. The diverse applications highlighted in this article only scratch the surface of its broad utility, illustrating its fundamental importance across multiple disciplines. Mastering the techniques for identifying and working with these coefficients empowers problem-solving and analysis in a myriad of fields. Continued exploration and practice with diverse examples will undoubtedly enhance your understanding and proficiency.
Latest Posts
Latest Posts
-
The Energy Currency Used By Cells Is
Apr 06, 2025
-
How Tall Is 39 Inches In Feet
Apr 06, 2025
-
3 Letter Words Starting With I
Apr 06, 2025
-
What Is The Latin Word For Sour
Apr 06, 2025
-
What Is The Least Common Multiple For 2 And 3
Apr 06, 2025
Related Post
Thank you for visiting our website which covers about Coefficient Of 𝑥 𝑚 X M . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
